Quasilinear parabolic problems with nonlinear boundary conditions

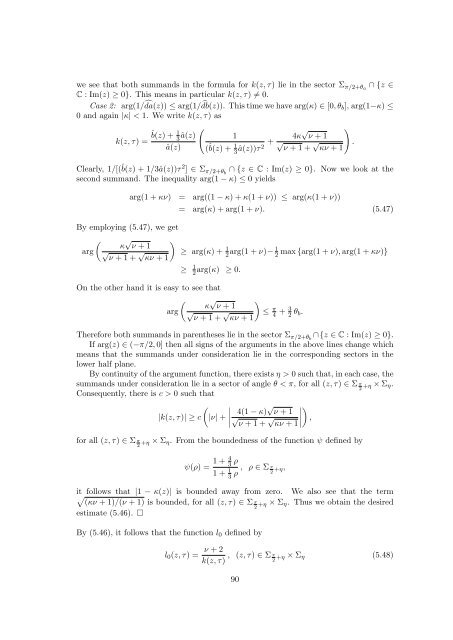
we see that both summands in the formula for k(z, τ) lie in the sector Σ π/2+θa ∩ {z ∈
C : Im(z) ≥ 0}. This means in particular k(z, τ) �= 0.
Case 2: arg(1/ � da(z)) ≤ arg(1/ � db(z)). This time we have arg(κ) ∈ [0, θb], arg(1−κ) ≤
0 and again |κ| < 1. We write k(z, τ) as
k(z, τ) = ˆb(z) + 1
3â(z) �
â(z)
1
( ˆb(z) + 1
+
3â(z))τ 2
4κ √ �
ν + 1
√ √ .
ν + 1 + κν + 1
Clearly, 1/[( ˆb(z) + 1/3â(z))τ 2 ] ∈ Σπ/2+θb ∩ {z ∈ C : Im(z) ≥ 0}. Now we look at the
second summand. The inequality arg(1 − κ) ≤ 0 yields
By employing (5.47), we get
�
κ
arg
√ �
ν + 1
√ √
ν + 1 + κν + 1
arg(1 + κν) = arg((1 − κ) + κ(1 + ν)) ≤ arg(κ(1 + ν))
= arg(κ) + arg(1 + ν). (5.47)
≥ arg(κ) + 1
1
2arg(1 + ν)− 2 max {arg(1 + ν), arg(1 + κν)}
≥ 1
2arg(κ) ≥ 0.
On the other hand it is easy to see that
�
κ
arg
√ �
ν + 1
√ √
ν + 1 + κν + 1
≤ π 3
4 + 2 θb.
Therefore both summands in parentheses lie in the sector Σπ/2+θb ∩ {z ∈ C : Im(z) ≥ 0}.
If arg(z) ∈ (−π/2, 0] then all signs of the arguments in the above lines change which
means that the summands under consideration lie in the corresponding sectors in the
lower half plane.
By continuity of the argument function, there exists η > 0 such that, in each case, the
summands under consideration lie in a sector of angle θ < π, for all (z, τ) ∈ Σ π
2 +η × Ση.
Consequently, there is c > 0 such that
�
|k(z, τ)| ≥ c |ν| +
�
�
�
4(1 − κ)
�
√ ��
ν + 1 �
√ √ �
ν + 1 + κν + 1
� ,
for all (z, τ) ∈ Σ π
2 +η × Ση. From the boundedness of the function ψ defined by
ψ(ρ) =
4 1 + 3 ρ
1 + 1
3 ρ , ρ ∈ Σ π
2 +η,
it follows that |1 − κ(z)| is bounded away from zero. We also see that the term
� (κν + 1)/(ν + 1) is bounded, for all (z, τ) ∈ Σ π
2 +η × Ση. Thus we obtain the desired
estimate (5.46). �
By (5.46), it follows that the function l0 defined by
l0(z, τ) =
ν + 2
k(z, τ) , (z, τ) ∈ Σ π
2 +η × Ση
90
(5.48)