1 6 - THE RADIATION FIELD Specific Intensity - I , I , or I ... - Physics

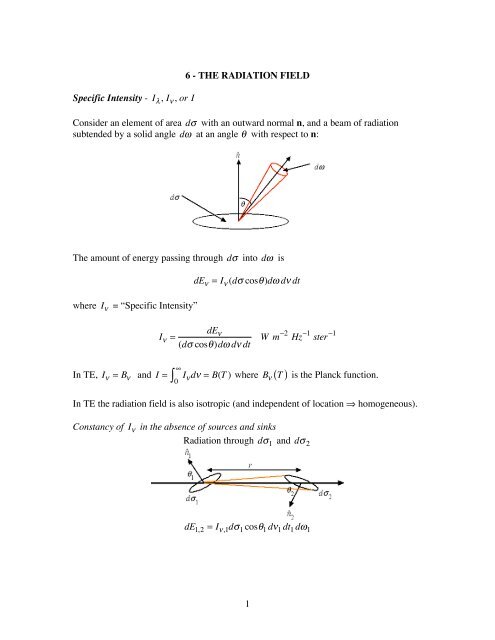
Specific Intensity - I , I , or I6 - THE RADIATION FIELDConsider an element of area d with an outward normal n, and a beam of radiationsubtended by a solid angle d at an angle with respect to n:The amount of energy passing through d into d iswhere I = “Specific Intensity”dE = I (d cos)d d dtI =dE (d cos)d d dtWm 2 Hz 1 ster 1In TE, I = B and I =I0 d = B(T ) where B T( ) is the Planck function.In TE the radiation field is also isotropic (and independent of location homogeneous).Constancy of I in the absence of sources and sinksRadiation through d 1 and d 2dE 1,2 = I ,1 d 1 cos 1 d 1 dt 1 d 11

ut d 1 = d 2 cos 2r 2 (d 1 includes the energy passing through d 2 )dE ,2 = I ,2 d 2 cos 2 d 2 dt 2 d 2 , but d 2 = d 1 cos 1r 2If we let d 1 = d 2 , dt 1 = dt 2 (measure both similarly), in the absence of sources or sinksof radiation, dE ,1 = dE ,2 , yielding:I ,1 = I ,2Basically, this says that the surface brightness of an object will be independent of itsdistance.In general, I may depend on both the angles , in a spherical coordinate systemMean Intensity (Mean Specific Intensity) J , J , or J (some books use I )I dJ = = 1 I d 4 d[For a review of the integration of d over a unit sphere, see the discussion at the end ofthis chapter]Special Cases:1. Isotropic radiation field, i.e. I is not a function of , :J = 14 I d = I 4d = I J = I 2

2. Isotropically-emitting surface, whereI (,) = I for = 0 to 2I (,) = 0 for = 2 to J = 144 I d = 12 =0 2 =02 I sindd + 0sindd =0 = 2 J = I 2=03. Axisymmetric radiation fieldI (,) = I ()J = 142 I =0 =0 ()sindd= 1 I ()sind2 =0define μ cos dμ = sind1J = 1 I 2 (μ)(dμ) Zeroth moment of I+1so, J = 1 2+1I1 (μ)dμ3

FluxThe net rate of energy flowing through ddE = I d cos dt d dExamples:dE d dtd = I cosd erg cm2s 1 Hz 1 or W m 2 Hz 11. Isotropic I = I 0, = I 0,2. Isotropically-emitting surface2 1cosd = I 0 cos sindd = 2 I =0 =00,2 sin2 2 =0I = I 0, for = 0 to = I2 =0 0,= 0 for = 2 to 20= 0cos sinddsin 2 2= 2 I 0, = I0,2 0For a blackbody,I = B so = B and = B = T 4 = T 4 Wm -2Thus, the net flux of a blackbody (per unit area) is T 4 Wm 2For a spherical star of radius R,Luminosity = L = 4 R 2 T 4W4

3. Axisymmetric case I = I () =2 I =0 =0 d ()cos sindd= 2 I ()cos sind =0+1I1 2 (μ)μdμ 1st Moment of IThere are also 2 other definitions of “Flux” in use in the literature:“astrophysical flux”“Eddington flux” = F = 2 4 = H = 1 21I1 1I1 (μ)μdμ(μ)μdμRadiation PressureCases:rate of transfer of momentum= momentum of radiation projected area component to arearate of transfer of momentum =rate of transfer of momentumd dt d I c d cos cos d dt d= P r= 1 cI cos 2 d1. Isotropic I = I 0For a blackbodyP r= I 0,c= 43c I 0,2 =0 =0cos 2 sinddP r= 43c B P r = 43c B = 43cwhereac4 T 4 = 4 ac3c 41 T 4 = 1 3 aT 4= and a = "radiation constant"5

2. Axisymmetric caseP r= 2 c= 1 c2 I =0 =0 1I1 ()cos 2 sindd (μ)μ 2 dμ 2nd Moment of IOne also sees this expressed asSUMMARYK = cP r 4 = 1 21I1 (μ)μ 2 dμGenerally Isotropic AxisymmetricJ = 14 I d J = I 0 J = 1 211I(μ)dμ =P r = 1 cI cosdI cos 2 d = 0F = 0H = 0P r = 43c I 0K = 1 3 I 03K = J = 2F = 2H = 1 2P r = 2 cK = 1 211111111P r = 4 c KI(μ)μdμI(μ)μdμ11I(μ)μdμI(μ)μ 2 dμI(μ)μ 2 dμAnd, if TE applies,I = B NOTE:JH = 1 2K 11012I μ μ dμ( )6

Energy DensitydU =dE d ( d cdt) = I c dU = 1 cU = 3P r I d = 4 c J = 4 c B (T )in TEif in TEAPPENDIXINTEGRATION OF SOLID ANGLEd = Arear 22( )( r sind )r r= rd2= sinddd = sindd = d sind =0 =0[ ] 0 = 2 1= 2 cos =0 =0( )+ 1 = 47