Metrics of curves in shape optimization and analysis - Andrea Carlo ...

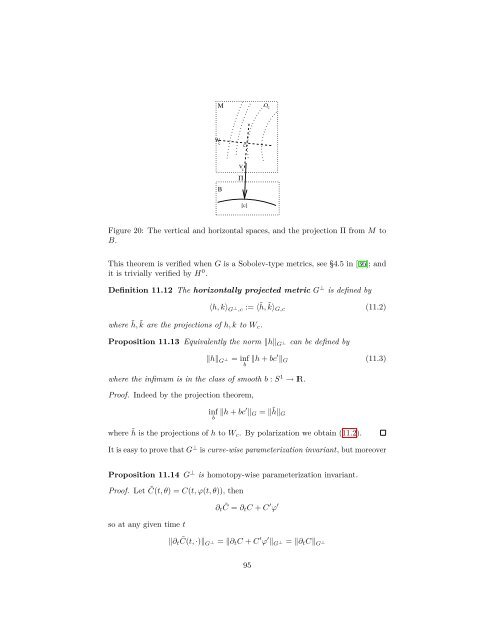
MO cW ccBVcΠ[c]Figure 20: The vertical and horizontal spaces, and the projection Π from M toB.This theorem is verified when G is a Sobolev-type metrics, see §4.5 in [36]; andit is trivially verified by H 0 .Definition 11.12 The horizontally projected metric G ⊥ is defined bywhere ˜h, ˜k are the projections of h, k to W c .〈h, k〉 G ⊥ ,c := 〈˜h, ˜k〉 G,c (11.2)Proposition 11.13 Equivalently the norm ‖h‖ G ⊥can be defined by‖h‖ G ⊥where the infimum is in the class of smooth b : S 1 → lR.Proof. Indeed by the projection theorem,= infb ‖h + bc′ ‖ G (11.3)inf ‖h +bbc′ ‖ G = ‖˜h‖ Gwhere ˜h is the projections of h to W c . By polarization we obtain (11.2).It is easy to prove that G ⊥ is curve-wise parameterization invariant, but moreoverProposition 11.14 G ⊥ is homotopy-wise parameterization invariant.Proof. Let ˜C(t, θ) = C(t, ϕ(t, θ)), then∂ t ˜C = ∂t C + C ′ ϕ ′so at any given time t‖∂ t ˜C(t, ·)‖G ⊥ = ‖∂ t C + C ′ ϕ ′ ‖ G ⊥ = ‖∂ t C‖ G ⊥95