Metrics of curves in shape optimization and analysis - Andrea Carlo ...

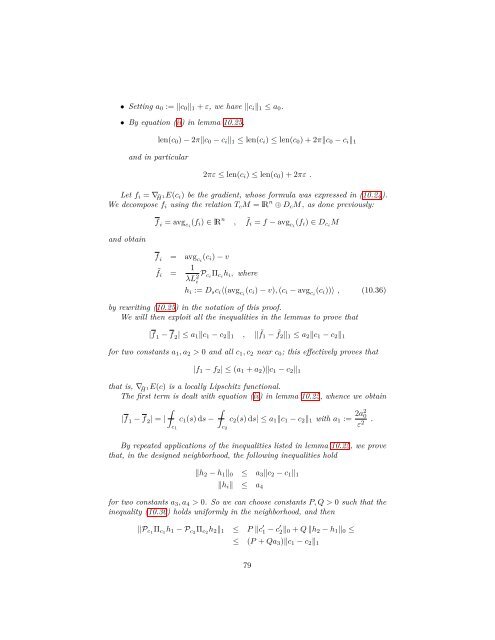
• Setting a 0 := ‖c 0 ‖ 1 + ε, we have ‖c i ‖ 1 ≤ a 0 .• By equation (ii) in lemma 10.25,len(c 0 ) − 2π‖c 0 − c i ‖ 1 ≤ len(c i ) ≤ len(c 0 ) + 2π‖c 0 − c i ‖ 1and in particular2πε ≤ len(c i ) ≤ len(c 0 ) + 2πε .Let f i = ∇˜H1E(c i ) be the gradient, whose formula was expressed in (10.24).We decompose f i using the relation T c M = lR n ⊕ D c M, as done previously:and obtainf i = avg ci(f i ) ∈ lR n , ˜fi = f − avg ci(f i ) ∈ D ci Mf i = avg ci(c i ) − v1˜f i = P ci Π ci h i , whereλL 2 ih i := D s c i 〈(avg ci(c i ) − v), (c i − avg ci(c i ))〉 , (10.36)by rewriting (10.25) in the notation of this proof.We will then exploit all the inequalities in the lemmas to prove that|f 1 − f 2 | ≤ a 1 ‖c 1 − c 2 ‖ 1 , ‖ ˜f 1 − ˜f 2 ‖ 1 ≤ a 2 ‖c 1 − c 2 ‖ 1for two constants a 1 , a 2 > 0 and all c 1 , c 2 near c 0 ; this effectively proves that|f 1 − f 2 | ≤ (a 1 + a 2 )‖c 1 − c 2 ‖ 1that is, ∇˜H1E(c) is a locally Lipschitz functional.The first term is dealt with equation (iv) in lemma 10.25, whence we obtain∫∫|f 1 − f 2 | = | c 1 (s) ds − c 2 (s) ds| ≤ a 1 ‖c 1 − c 2 ‖ 1 with a 1 := 2a2 0c 1 c 2ε 2 .By repeated applications of the inequalities listed in lemma 10.25, we provethat, in the designed neighborhood, the following inequalities hold‖h 2 − h 1 ‖ 0 ≤ a 3 ‖c 2 − c 1 ‖ 1‖h i ‖ ≤ a 4for two constants a 3 , a 4 > 0. So we can choose constants P, Q > 0 such that theinequality (10.30) holds uniformly in the neighborhood, and then‖P c1 Π c1 h 1 − P c2 Π c2 h 2 ‖ 1 ≤ P ‖c ′ 1 − c ′ 2‖ 0 + Q ‖h 2 − h 1 ‖ 0 ≤≤ (P + Qa 3 )‖c 1 − c 2 ‖ 179