Metrics of curves in shape optimization and analysis - Andrea Carlo ...
Metrics of curves in shape optimization and analysis - Andrea Carlo ... Metrics of curves in shape optimization and analysis - Andrea Carlo ...
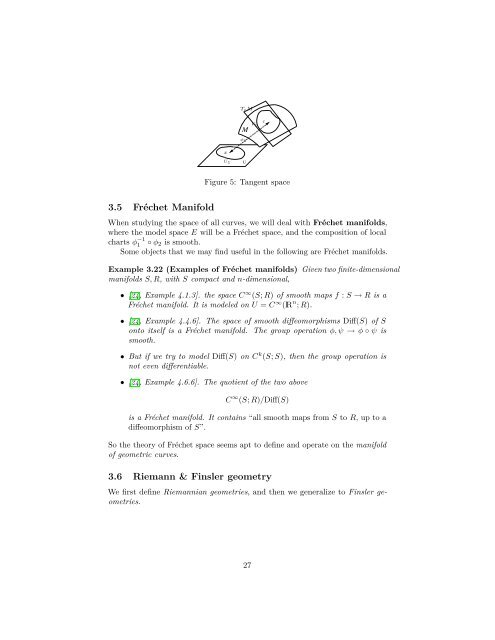
Since φ k are homeomorphisms, then the topology of M is “identical” to thetopology of U. The rôle of the charts is to define the differentiable structure ofM mimicking that of U. See for example the definition of directional derivativein eqn. (3.4).3.3.1 SubmanifoldDefinition 3.19 Suppose A, B are open subsets of two linear spaces. A diffeomorphismis an invertible C 1 function φ : A → B, whose inverse φ −1 is againC 1 .Let U be a fixed closed linear subspace of a l.c.t.v.s. X.Definition 3.20 A submanifold is a subset Mof X, such that, at any point c ∈ M we may finda chart φ k : U k → V k , with V k , U k ⊂ X open sets,c ∈ V k . The maps φ k are diffeomorphisms, andφ k maps U ∩ U k onto M ∩ V k .Most often, M = {Φ(c) = 0} where Φ : X → Y ; so, to prove that M is asubmanifold, we will use the implicit function theorem.Note that M is itself an abstract manifold, and the model space is U; sodim(M) = dim(U) ≤ dim(X). Vice versa any abstract manifold is a submanifoldof some large X (by well known embedding theorems).3.4 Tangent space and tangent bundleLet us fix a differentiable manifold M, and c ∈ M. We want to define thetangent space T c M of M at c.Definition 3.21 • The tangent space T c M is more easily described forsubmanifolds; in this case, we choose a chart φ k and a point x ∈ U k s.t.φ k (x) = c; T c M is the image of the linear space U under the derivativeD x φ k . T c M is itself a linear subspace in X. In figure 5 on the followingpage, we graphically represent T c M though as an affine subspace, bytranslating it to the point c.• The tangent bundle T M is the collection of all tangent spaces. If M isa submanifold of the vector space X, thenT M := {(c, h) | c ∈ M, h ∈ T c M} ⊂ X × X .The tangent bundle T M is itself a differentiable manifold; its charts are of theform (φ k , Dφ k ), where φ k are the charts for M.XU 1Mφ 1UV 1c26
T cMMV 1cφ 1xU 1UFigure 5: Tangent space3.5 Fréchet ManifoldWhen studying the space of all curves, we will deal with Fréchet manifolds,where the model space E will be a Fréchet space, and the composition of localcharts φ −11 ◦ φ 2 is smooth.Some objects that we may find useful in the following are Fréchet manifolds.Example 3.22 (Examples of Fréchet manifolds) Given two finite-dimensionalmanifolds S, R, with S compact and n-dimensional,• [24, Example 4.1.3]. the space C ∞ (S; R) of smooth maps f : S → R is aFréchet manifold. It is modeled on U = C ∞ (lR n ; R).• [24, Example 4.4.6]. The space of smooth diffeomorphisms Diff(S) of Sonto itself is a Fréchet manifold. The group operation φ, ψ → φ ◦ ψ issmooth.• But if we try to model Diff(S) on C k (S; S), then the group operation isnot even differentiable.• [24, Example 4.6.6]. The quotient of the two aboveC ∞ (S; R)/Diff(S)is a Fréchet manifold. It contains “all smooth maps from S to R, up to adiffeomorphism of S”.So the theory of Fréchet space seems apt to define and operate on the manifoldof geometric curves.3.6 Riemann & Finsler geometryWe first define Riemannian geometries, and then we generalize to Finsler geometries.27
- Page 1 and 2: Metrics of curves in shape optimiza
- Page 3 and 4: shape analysis where we study a fam
- Page 5 and 6: • F (c) = F (c ◦ φ) for all cu
- Page 7 and 8: κ > 0HNNHNHκ < 0Figure 1: Example
- Page 9 and 10: In the case of planar curves c 1 ,
- Page 11 and 12: (a) (b) (c) (d) (e)Figure 3: Segmen
- Page 13 and 14: where φ may be chosen to beφ(x) =
- Page 15 and 16: 2.4.1 Example: geometric heat flowW
- Page 17 and 18: 2.4.5 Centroid energyWe will now pr
- Page 20 and 21: shapes. Unfortunately, H 0 does not
- Page 22 and 23: • If the second request is waived
- Page 24 and 25: • The Fréchet space of smooth fu
- Page 28 and 29: 3.6.1 Riemann metric, lengthDefinit
- Page 30 and 31: Theorem 3.30 Suppose that M is a sm
- Page 32 and 33: Example 3.38 Let M = C ∞ ([−1,
- Page 34 and 35: • sometimes S 1 will be identifie
- Page 36 and 37: The proof is by direct computation.
- Page 38 and 39: The term preshape space is sometime
- Page 40 and 41: 5 Representation/embedding/quotient
- Page 42 and 43: 6.1.1 Length induced by a distanceI
- Page 44 and 45: 0101200000000000011111111111110000
- Page 46 and 47: 6.2.4 Applications in computer visi
- Page 48 and 49: of a small ball from A. The motion
- Page 50 and 51: CutΩ(The arrows represent the dist
- Page 52 and 53: 7.3 L 1 metric and Plateau problemI
- Page 54 and 55: Definition 8.3 (Flat curves) Let Z
- Page 56 and 57: Proof. Fix α 0 ∈ S \ Z. Let T =
- Page 58 and 59: 8.2.2 RepresentationThe Stiefel man
- Page 60 and 61: 9.3 Conformal metricsYezzi and Menn
- Page 62 and 63: 10.1.1 Related worksA family of met
- Page 64 and 65: Definition 10.7 (Convolution) A arc
- Page 66 and 67: and̂∇˜HjE(0) = ̂∇ H 0E(0),̂
- Page 68 and 69: and we simply integrate twice! More
- Page 70 and 71: Corollary 10.18 In particular, the
- Page 72 and 73: 10.6 Existence of gradient flowsWe
- Page 74 and 75: • The length functional (from C 1
T cMMV 1cφ 1xU 1UFigure 5: Tangent space3.5 Fréchet ManifoldWhen study<strong>in</strong>g the space <strong>of</strong> all <strong>curves</strong>, we will deal with Fréchet manifolds,where the model space E will be a Fréchet space, <strong>and</strong> the composition <strong>of</strong> localcharts φ −11 ◦ φ 2 is smooth.Some objects that we may f<strong>in</strong>d useful <strong>in</strong> the follow<strong>in</strong>g are Fréchet manifolds.Example 3.22 (Examples <strong>of</strong> Fréchet manifolds) Given two f<strong>in</strong>ite-dimensionalmanifolds S, R, with S compact <strong>and</strong> n-dimensional,• [24, Example 4.1.3]. the space C ∞ (S; R) <strong>of</strong> smooth maps f : S → R is aFréchet manifold. It is modeled on U = C ∞ (lR n ; R).• [24, Example 4.4.6]. The space <strong>of</strong> smooth diffeomorphisms Diff(S) <strong>of</strong> Sonto itself is a Fréchet manifold. The group operation φ, ψ → φ ◦ ψ issmooth.• But if we try to model Diff(S) on C k (S; S), then the group operation isnot even differentiable.• [24, Example 4.6.6]. The quotient <strong>of</strong> the two aboveC ∞ (S; R)/Diff(S)is a Fréchet manifold. It conta<strong>in</strong>s “all smooth maps from S to R, up to adiffeomorphism <strong>of</strong> S”.So the theory <strong>of</strong> Fréchet space seems apt to def<strong>in</strong>e <strong>and</strong> operate on the manifold<strong>of</strong> geometric <strong>curves</strong>.3.6 Riemann & F<strong>in</strong>sler geometryWe first def<strong>in</strong>e Riemannian geometries, <strong>and</strong> then we generalize to F<strong>in</strong>sler geometries.27