Chapter 13 - Davidson Physics

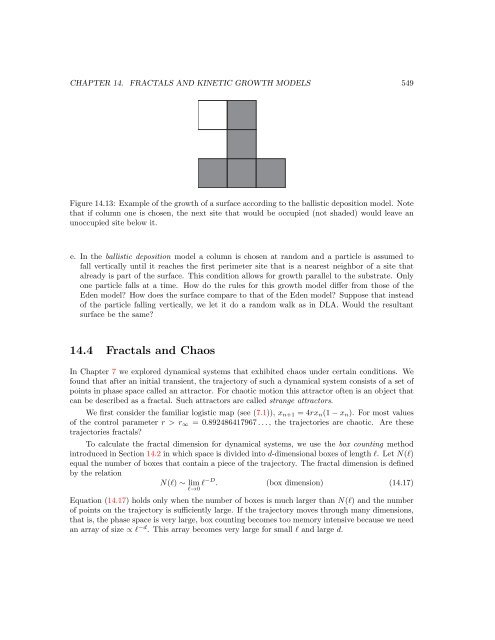
CHAPTER 14. FRACTALS AND KINETIC GROWTH MODELS 549Figure 14.13: Example of the growth of a surface according to the ballistic deposition model. Notethat if column one is chosen, the next site that would be occupied (not shaded) would leave anunoccupied site below it.e. In the ballistic deposition model a column is chosen at random and a particle is assumed tofall vertically until it reaches the first perimeter site that is a nearest neighbor of a site thatalready is part of the surface. This condition allows for growth parallel to the substrate. Onlyone particle falls at a time. How do the rules for this growth model differ from those of theEden model? How does the surface compare to that of the Eden model? Suppose that insteadof the particle falling vertically, we let it do a random walk as in DLA. Would the resultantsurface be the same?14.4 Fractals and ChaosIn Chapter 7 we explored dynamical systems that exhibited chaos under certain conditions. Wefound that after an initial transient, the trajectory of such a dynamical system consists of a set ofpoints in phase space called an attractor. For chaotic motion this attractor often is an object thatcan be described as a fractal. Such attractors are called strange attractors.We first consider the familiar logistic map (see (7.1)), x n+1 = 4rx n (1 − x n ). For most valuesof the control parameter r > r ∞ = 0.892486417967 . . . , the trajectories are chaotic. Are thesetrajectories fractals?To calculate the fractal dimension for dynamical systems, we use the box counting methodintroduced in Section 14.2 in which space is divided into d-dimensional boxes of length l. Let N(l)equal the number of boxes that contain a piece of the trajectory. The fractal dimension is definedby the relationN(l) ∼ lim l −D . (box dimension) (14.17)l→0Equation (14.17) holds only when the number of boxes is much larger than N(l) and the numberof points on the trajectory is sufficiently large. If the trajectory moves through many dimensions,that is, the phase space is very large, box counting becomes too memory intensive because we needan array of size ∝ l −d . This array becomes very large for small l and large d.