Solution - Career Point

More documents

Recommendations

Info

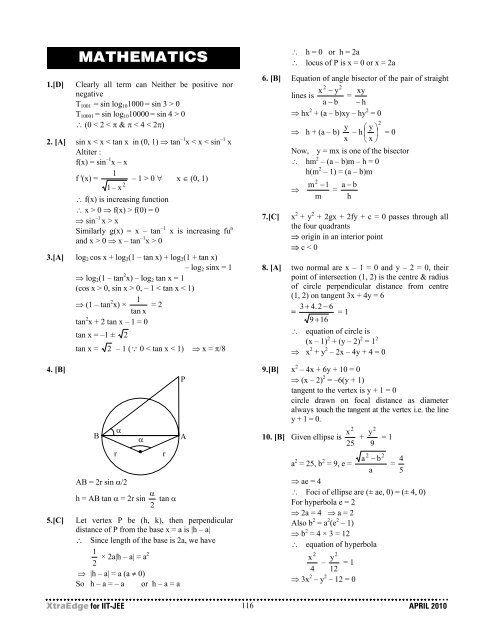
1.[D]MATHEMATICSClearly all term can Neither be positive nornegativeT 1001 = sin log 10 1000 = sin 3 > 0T 10001 = sin log 10 10000 = sin 4 > 0∴ (0 < 2 < π & π < 4 < 2π)2. [A] sin x < x < tan x in (0, 1) ⇒ tan –1 x < x < sin –1 xAltiter :f(x) = sin –1 x – xf '(x) =1– 1 > 0 ∀ x ∈ (0, 1)21– x∴ f(x) is increasing function∴ x > 0 ⇒ f(x) > f(0) = 0⇒ sin –1 x > xSimilarly g(x) = x – tan –1 x is increasing fu nand x > 0 ⇒ x – tan –1 x > 03.[A] log 2 cos x + log 2 (1 – tan x) + log 2 (1 + tan x)– log 2 sinx = 1⇒ log 2 (1 – tan 2 x) – log 2 tan x = 1(cos x > 0, sin x > 0, – 1 < tan x < 1)⇒ (1 – tan 2 1x) × = 2tan xtan 2 x + 2 tan x – 1 = 0tan x = –1 ± 2tan x = 2 – 1 (Q 0 < tan x < 1) ⇒ x = π/8∴ h = 0 or h = 2a∴ locus of P is x = 0 or x = 2a6. [B] Equation of angle bisector of the pair of straight2 2x − y xylines is =a − b − h⇒ hx 2 + (a – b)xy – hy 2 = 07.[C]2y ⎛ y ⎞⇒ h + (a – b) – h ⎜ ⎟⎠ = 0x ⎝ xNow, y = mx is one of the bisector∴ hm 2 – (a – b)m – h = 0h(m 2 – 1) = (a – b)m⇒m 2 − 1 a − b =m hx 2 + y 2 + 2gx + 2fy + c = 0 passes through allthe four quadrants⇒ origin in an interior point⇒ c < 08. [A] two normal are x – 1 = 0 and y – 2 = 0, theirpoint of intersection (1, 2) is the centre & radiusof circle perpendicular distance from centre(1, 2) on tangent 3x + 4y = 63 + 4.2 − 6== 19 + 16∴ equation of circle is(x – 1) 2 + (y – 2) 2 = 1 2⇒ x 2 + y 2 – 2x – 4y + 4 = 04. [B]5.[C]BrαAB = 2r sin α/2αh = AB tan α = 2r sin 2α tan αLet vertex P be (h, k), then perpendiculardistance of P from the base x = a is |h – a|∴ Since length of the base is 2a, we have1 × 2a|h – a| = a22⇒ |h – a| = a (a ≠ 0)So h – a = – a or h – a = arPA9.[B] x 2 – 4x + 6y + 10 = 0⇒ (x – 2) 2 = –6(y + 1)tangent to the vertex is y + 1 = 0circle drawn on focal distance as diameteralways touch the tangent at the vertex i.e. the liney + 1 = 0.x 210. [B] Given ellipse is + 25y 2 = 192 −a 2 = 25, b 2 a b 4= 9, e = =a 5⇒ ae = 4∴ Foci of ellipse are (± ae, 0) = (± 4, 0)For hyperbola e = 2⇒ 2a = 4 ⇒ a = 2Also b 2 = a 2 (e 2 – 1)⇒ b 2 = 4 × 3 = 12∴ equation of hyperbola4x 2 – 12y 2 = 1⇒ 3x 2 – y 2 – 12 = 02XtraEdge for IIT-JEE 116APRIL 2010
11.[B]→ →∴ α & β are two mutually perpendicularunit vector.→ →∴ α × β is a unit vector perpendicular toboth → α & → β . So we can consider → α , → β , → α ×→β as î , ĵ & kˆ .Given vector are coplanar soa a c1 0 1 = 0c c b⇒ a(– c) + a(c – b) + c 2 = 0 ⇒ c 2 = ab12. [D] S 1 = x 2 + y 2 + z 2 + 2x – 4y – 4z – 7 = 0centre C 1 = (–1, 2, 2) and radius r 1 = 4S 2 = x 2 + y 2 + z 2 + 2x – 4y – 16z + 65 = 0centre C 2 = (–1, 2, 8) radius r 2 = 2C 1 C 2 = 6; r 1 + r 2 = 6∴ sphere S 1 & S 2 touches externaly∴ point of contact divides C 1 C 2 in the ratio 4 : 2∴ point of contact = (–1, 2, 6)413.[D] y = f(x) = 3 –2x − 4x + 8⇒ (3 – y)x 2 – 4(3 – y)x + 20 – 8y = 0x ∈ R ∴ D ≥ 0⇒ 16(3 – y) 2 – 4(3 – y) (20 – 8y) ≥ 0, y ≠ 3⇒ –y 2 + 5y – 6 ≥ 0; y ≠ 3⇒ (y – 2) (y – 3) ≤ 0 ⇒ 2 ≤ y < 314. [B] x > 0, g(x) is boundednxf (x)e + g(x)∴ limn→∞nx1+enxf (x) + g(x) / elim= f(x)n→∞nx1+1 e[g(x) is bounded ⇒15.[B] a 1 = 1, a n = n(1 + a n–1 )a ng(x)nxefinite⇒ = 0] infinte⇒ 1 + a n–1 =n⎛ 1 ⎞ ⎛ 1 ⎞ ⎛ 1∴ limn→∞⎜1+ ⎟⎝ a⎜1 + ⎟ ....1 ⎠ ⎝ a⎜1+2 ⎠ ⎝ a nlim ⎛ 1+an→∞⎟ ⎞⎜1⎛ 1+a ⎞⎝ a⎜2⎛ 1+a⎟ ....1 ⎠ ⎝ a⎜2 ⎠ ⎝ a n= lim a 2 a. 3 a........ n + 1 1 .n→∞2 3 n + 1 a1a2...a na n+1 (1 + a n )(1 + n)= lim = limn →∞ n + 1 n →∞ n + 1⎞⎟⎠n⎞⎟⎠⎛ ⎞= ⎜ 1 a ⎛nlim + ⎟ = ⎜ 1 1 a n−1lim + +n→∞⎜n n ⎟ n ∞⎜n n −1n −⎝ ⎠ ⎝⎪⎧1 1 1 1 a ⎪⎫1= lim ⎨ + + .... + + ⎬n→∞n n −12 1 1⎪⎩⎪⎭⎪⎧1 1 1 ⎪⎫= lim ⎨1+ + + .... ⎬ {Q a 1 = 1}n→∞1 2 n⎪⎩⎪⎭= e⎧−2⎪16.[A] 2 sgn 2x = ⎨ 0⎪⎩ 2;;;→ 1x < 0x = 0x > 0⎧4x ≠ 0f(x) = |2 sgn 2x| + 2 = ⎨⎩2x = 0∴ By defining f(0) = 4, f(x) will becomecontinuous function at x = 0 as thenf(0 – 0) = f(0 + 0) = 4Hence at x = 0 f(x) has removable discontinuity17.[A] Q 1 < x < 2 ⇒ [x] = 1π 3⎛ ⎞∴ f(x) = cos ⎜ − x ⎟ = sin x 3⎝ 2 ⎠f ´(x) = cos x 3 . 3x 22/ 3⎛ ⎞⇒ f ´ ⎜π⎟π ⎛ π3 = 3 cos . ⎝ 2 ⎠ 2 2 ⎟ ⎞⎜ = 0⎝ ⎠18.[C] ye x = cos x ...(1)ye x + y 1 e x = – sin x ...(2)again differentiatingye x + y 1 e x + y 1 e x + y 2 e x = – cos xye x + 2y 1 e x + y 2 e x = – ye x (from (1))⇒ 2y + 2y 1 + y 2 = 0 ...(3)again differentiating2y 1 + 2y 2 + y 3 = 0again differentiating2y 2 + 2y 3 + y 4 = 0 ...(4)from (1) (2) & (3)4y + y 4 = 0y∴ 4 = – 4y19.[A] fog = I ⇒ fog(x) = I(x) = x∴ fg(x) = x⇒ f´g(x) × g´(x) = 1⇒ f´g(a) × g´(a) = 1⇒ f´(b) × 2 = 1⇒ f´(b) = 21⎞⎟⎟⎠XtraEdge for IIT-JEE 117APRIL 2010
Page 3 and 4:
Volume - 5 Issue - 10April, 2010 (M
Page 5 and 6:
Volume-5 Issue-10April, 2010 (Month
Page 7 and 8:
Hospital in Parel and ApolloHospita
Page 9:
KNOW IIT-JEEBy Previous Exam Questi
Page 12 and 13:
CHEMISTRY6. From the following data
Page 14 and 15:
(ii)OHH + CH 3OH⊕PorClPClClOCH 3O
Page 16 and 17:
Physics Challenging ProblemsSet #12
Page 18 and 19:
8 QuestionsSolutionSet # 11Physics
Page 20 and 21:
8 QuestionsSolutionSet # 12Physics
Page 22 and 23:
PHYSICSSStudents'ForumExpert’s So
Page 24 and 25:
If the body loses this heat in time
Page 26 and 27:
Perfect gas equation :From the kine
Page 28 and 29:
The internal energy of n molecules
Page 30 and 31:
* The binding energy per nucleon is
Page 32 and 33:
KEY CONCEPTOrganicChemistryFundamen
Page 34 and 35:
KEY CONCEPTPhysicalChemistryFundame
Page 36 and 37:
UNDERSTANDINGOrganic Chemistry1. An
Page 38 and 39:
The starting compound (A) reacts wi
Page 40 and 41:
`tà{xÅtà|vtÄ V{tÄÄxÇzxá12Se
Page 43 and 44:
MATHEMATICAL CHALLENGESSOLUTION FOR
Page 45 and 46:
A 2 ⎛ 3s − 2s ⎞ s≤ s ⎜
Page 47 and 48:
5. Let z 1 , z 2 and z 3 are unimod
Page 49 and 50:
If I n =∫x n . e ax dx, then I n
Page 51 and 52:
The common difference of an A.P. is
Page 53 and 54:
XtraEdge for IIT-JEE 51APRIL 2010
Page 55 and 56:
4. Consider the following reactions
Page 57 and 58:
15. What would be the structure of
Page 59 and 60:
14. If line lies inside the region
Page 61:
6. A BTwo containers A & B contain
Page 64 and 65:
MOCK TEST FOR IIT-JEEPAPER - IITime
Page 66 and 67:
SECTION - IVInteger answer typeThis
Page 68 and 69: X Y Z W0123456789012345678912. If z
Page 70 and 71: (C) Pressure at A > pressureat B(D)
Page 72 and 73: 7. Experimental verification of New
Page 74 and 75: 28. Assertion : A rocket moves forw
Page 76 and 77: 60. Point out the incorrect stateme
Page 78 and 79: MOCK TEST - BIT-SATTime : 3 Hours T
Page 80 and 81: 22. The displacement of interfering
Page 82: 40. A body of mass 2 kg is being dr
Page 85 and 86: 10. The foci of a hyperbola coincid
Page 87 and 88: LOGICAL REASONING1. Fill in the bla
Page 89 and 90: SOLUTION FOR MOCK TESTIIT-JEE (PAPE
Page 91 and 92: or 0.1 v α + 1 = 2 + 2 × 10 -2 ×
Page 93 and 94: 12.[A, B, C, D]13.[B]2x t − 5t +
Page 95 and 96: R l=sin 45º sin 30ºR sin 45º = =
Page 97 and 98: 1.[C]2.[B]3.[B]SOLUTION FOR MOCK TE
Page 99 and 100: 5.[A,B,D] We have adj A = |A| A -1a
Page 101 and 102: (1, 1)7λ 5.5× 10t min = =P4n 4×1
Page 103 and 104: K TFor a cylinder = 2 in case ofK R
Page 105 and 106: ∴17.[C]Potential of shell A is,1
Page 107 and 108: 39.[B] Solution is decinormal, that
Page 109 and 110: 71.[B]which is a H.P.n th term of c
Page 111 and 112: 85.[C] Letters of word 'STATISTICS'
Page 113 and 114: 13. [B] Point P lies on the arms CD
Page 115 and 116: 33. [B] For quarter revolution∆ V
Page 117: 16.[A]NH 2⎯ 0−5°C+ NaNO 2 + 2H
Page 121 and 122: 1/ 21 -2∫2 x 2 dx⇒⇒01 2 - 3 2
Page 124 and 125: 8.[A]9.[A]10.[B]To take off :irrele
Page 126: CAREER POINTIn associationwithLaunc
show all

Solution - Career Point

Create successful ePaper yourself

Delete template?

Save as template?