Algebra I: Section 6. The structure of groups. 6.1 Direct products of ...
Algebra I: Section 6. The structure of groups. 6.1 Direct products of ...
Algebra I: Section 6. The structure of groups. 6.1 Direct products of ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
a = [1]n <strong>of</strong> Zn to an element b = [r]m in Um that satisfies the compatibility condition b m = [1]m,<br />
then the corresponding group operation in the semidirect product Zm ×φ Zn takes the form<br />
(26) ([i]m, [j]n) · ([k]m, [ℓ]n) = ([i + r j k]m , [j + ℓ]n)<br />
Pro<strong>of</strong>: Since Zn is an additive group the j th “power” <strong>of</strong> the generator a is j · a = a + . . . + a,<br />
and since Φ is a homomorphism from an additive group to a multiplicative group we get<br />
Φ(j · a) = Φ(a + . . . + a)<br />
= Φ(a) ◦ . . . ◦ Φ(a)<br />
= Φ(a) j = τ j<br />
[r] = τ [r] j = τ [r j ]<br />
Hence, (suppressing some subscripts m, n for clarity), we get<br />
as required �<br />
([i]m, [j]n) · ([k]m, [ℓ]n) = ([i] + τ j<br />
[r] ([k]), [j] + [ℓ])<br />
We now turn to various other computed examples.<br />
= ([i] + [r] j [k], [j + ℓ]) = ([i + r j k]m , [j + ℓ]n)<br />
<strong>6.</strong>2.22 Example. Determine the group <strong>of</strong> units (U5, · ) and its isomorphism type. <strong>The</strong>n find all<br />
possible homomorphisms Φ : Z3 → U5. Describe the possible semidirect <strong>products</strong> G = Z5×φZ3.<br />
Discussion: Obviously<br />
Aut(Z5, +) ∼ = U5 = {[k] : gcd(k, 5) = 1} = {[1], [2], [3], [4]}<br />
and |U5| = 4. <strong>The</strong>re are two possibilities: U5 ∼ = Z4 and U5 ∼ = Z2 × Z2. If we calculate the<br />
orders <strong>of</strong> a few elements in U5 we find that o([2]) = 4, so (U5, · ) ∼ = (Z4, +).<br />
If Φ : Z3 → U5 is a homomorphism, the size |Φ(Z3)| <strong>of</strong> the image subgroup in U5 must be a<br />
divisor <strong>of</strong> both 3 and 4. Since gcd(3, 4) = 1, the range <strong>of</strong> Φ must be the trivial subgroup {[1]}<br />
in U5, so the only action Z3 × Z5 → Z5 by automorphisms is the trivial action. <strong>The</strong> direct<br />
product Z5 × Z3 ∼ = Z15 is the only possible semidirect product. (Notice that we didn’t need to<br />
know the <strong>structure</strong> <strong>of</strong> U5 to see this!) �<br />
<strong>6.</strong>2.23 Example. Repeat the last example taking N = Z9 and H = Z3.<br />
Discussion: Now Aut(N) = U9 = {[1], [2], [4], [5], [7], [8]} and |U9| = <strong>6.</strong> Up to isomorphism<br />
there are just two <strong>groups</strong> <strong>of</strong> order |G| = 6, and since the group <strong>of</strong> units is abelian we see that<br />
U9 ∼ = (Z6, +), without inspecting the orders <strong>of</strong> any elements in U9. [ In fact, o([2]) = 6 so<br />
a = [2] is a cyclic generator <strong>of</strong> U9.] However, it is useful to actually write down the orders o(x)<br />
<strong>of</strong> elements in U9 and the <strong>groups</strong> 〈x〉 they generate, see the Table below.<br />
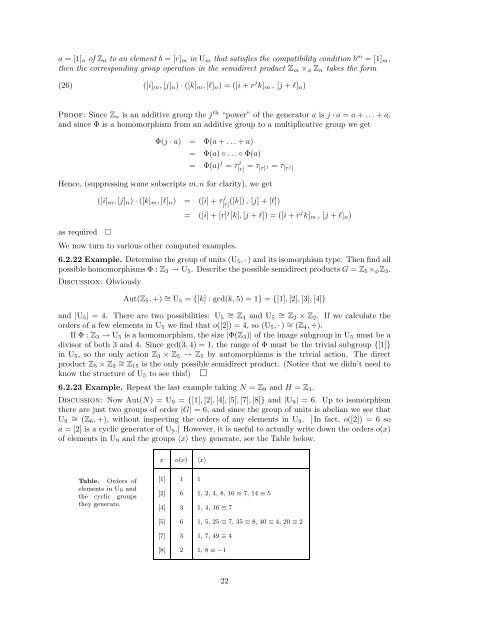
Table. Orders <strong>of</strong><br />
elements in U9 and<br />
the cyclic <strong>groups</strong><br />
they generate.<br />
x o(x) 〈x〉<br />
[1] 1 1<br />
[2] 6 1, 2, 4, 8, 16 ≡ 7, 14 ≡ 5<br />
[4] 3 1, 4, 16 ≡ 7<br />
[5] 6 1, 5, 25 ≡ 7, 35 ≡ 8, 40 ≡ 4, 20 ≡ 2<br />
[7] 3 1, 7, 49 ≡ 4<br />
[8] 2 1, 8 ≡ −1<br />
22