v2010.10.26 - Convex Optimization

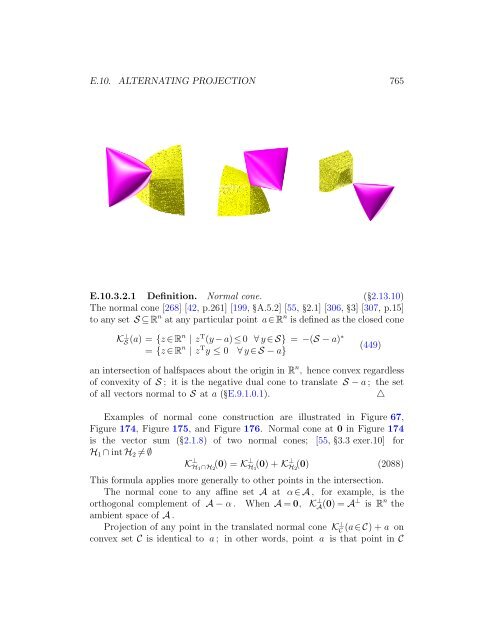
E.10. ALTERNATING PROJECTION 765E.10.3.2.1 Definition. Normal cone. (2.13.10)The normal cone [268] [42, p.261] [199,A.5.2] [55,2.1] [306,3] [307, p.15]to any set S ⊆ R n at any particular point a∈ R n is defined as the closed coneK ⊥ S (a) = {z ∈ Rn | z T (y −a)≤0 ∀y ∈ S} = −(S − a) ∗= {z ∈ R n | z T y ≤ 0 ∀y ∈ S − a}(449)an intersection of halfspaces about the origin in R n , hence convex regardlessof convexity of S ; it is the negative dual cone to translate S − a ; the setof all vectors normal to S at a (E.9.1.0.1).△Examples of normal cone construction are illustrated in Figure 67,Figure 174, Figure 175, and Figure 176. Normal cone at 0 in Figure 174is the vector sum (2.1.8) of two normal cones; [55,3.3 exer.10] forH 1 ∩ int H 2 ≠ ∅K ⊥ H 1 ∩ H 2(0) = K ⊥ H 1(0) + K ⊥ H 2(0) (2088)This formula applies more generally to other points in the intersection.The normal cone to any affine set A at α∈ A , for example, is theorthogonal complement of A − α . When A = 0, KA ⊥(0) = A⊥ is R n theambient space of A .Projection of any point in the translated normal cone KC ⊥ (a∈ C) + a onconvex set C is identical to a ; in other words, point a is that point in C