v2010.10.26 - Convex Optimization
v2010.10.26 - Convex Optimization v2010.10.26 - Convex Optimization
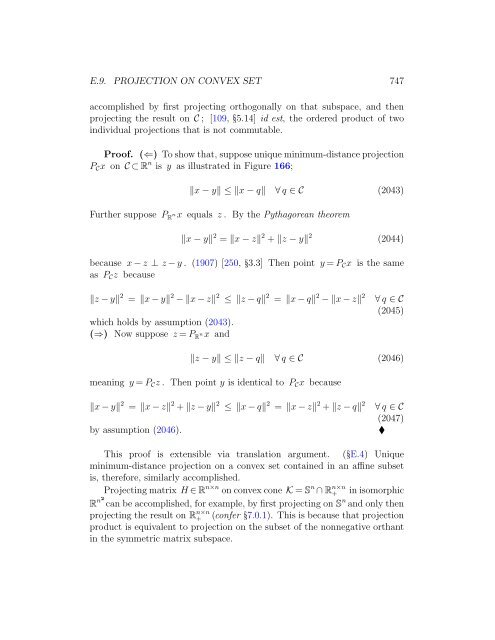
746 APPENDIX E. PROJECTION❇❇❇❇❇❇❇❇ x ❜❜R n⊥ ❙❇ ❜❇❇❇❇◗◗◗◗ ❙❙❙❙❙❙ ❜❜❜❜❜z❜y❜❜❜❜✧ ✧✧✧✧✧✧✧✧✧✧✧✧✧ R n❜❜❜C❜❜❜❜❜x −z ⊥ z −y ❜❇❜❇❇❇❇ ❜❜❜❜❜ ✧ ✧✧✧✧✧✧✧✧✧✧✧✧✧Figure 166: Closed convex set C belongs to subspace R n (shown boundedin sketch and drawn without proper perspective). Point y is uniqueminimum-distance projection of x on C ; equivalent to product of orthogonalprojection of x on R n and minimum-distance projection of result z on C .
E.9. PROJECTION ON CONVEX SET 747accomplished by first projecting orthogonally on that subspace, and thenprojecting the result on C ; [109,5.14] id est, the ordered product of twoindividual projections that is not commutable.Proof. (⇐) To show that, suppose unique minimum-distance projectionP C x on C ⊂ R n is y as illustrated in Figure 166;‖x − y‖ ≤ ‖x − q‖ ∀q ∈ C (2043)Further suppose P Rn x equals z . By the Pythagorean theorem‖x − y‖ 2 = ‖x − z‖ 2 + ‖z − y‖ 2 (2044)because x − z ⊥ z − y . (1907) [250,3.3] Then point y = P C x is the sameas P C z because‖z − y‖ 2 = ‖x − y‖ 2 − ‖x − z‖ 2 ≤ ‖z − q‖ 2 = ‖x − q‖ 2 − ‖x − z‖ 2which holds by assumption (2043).(⇒) Now suppose z = P Rn x and∀q ∈ C(2045)‖z − y‖ ≤ ‖z − q‖ ∀q ∈ C (2046)meaning y = P C z . Then point y is identical to P C x because‖x − y‖ 2 = ‖x − z‖ 2 + ‖z − y‖ 2 ≤ ‖x − q‖ 2 = ‖x − z‖ 2 + ‖z − q‖ 2by assumption (2046).∀q ∈ C(2047)This proof is extensible via translation argument. (E.4) Uniqueminimum-distance projection on a convex set contained in an affine subsetis, therefore, similarly accomplished.Projecting matrix H ∈ R n×n on convex cone K = S n ∩ R n×n+ in isomorphicR n2 can be accomplished, for example, by first projecting on S n and only thenprojecting the result on R n×n+ (confer7.0.1). This is because that projectionproduct is equivalent to projection on the subset of the nonnegative orthantin the symmetric matrix subspace.
- Page 695 and 696: D.2. TABLES OF GRADIENTS AND DERIVA
- Page 697 and 698: D.2. TABLES OF GRADIENTS AND DERIVA
- Page 699 and 700: Appendix EProjectionFor any A∈ R
- Page 701 and 702: 701U T = U † for orthonormal (inc
- Page 703 and 704: E.1. IDEMPOTENT MATRICES 703where A
- Page 705 and 706: E.1. IDEMPOTENT MATRICES 705order,
- Page 707 and 708: E.1. IDEMPOTENT MATRICES 707are lin
- Page 709 and 710: E.3. SYMMETRIC IDEMPOTENT MATRICES
- Page 711 and 712: E.3. SYMMETRIC IDEMPOTENT MATRICES
- Page 713 and 714: E.3. SYMMETRIC IDEMPOTENT MATRICES
- Page 715 and 716: E.4. ALGEBRA OF PROJECTION ON AFFIN
- Page 717 and 718: E.5. PROJECTION EXAMPLES 717a ∗ 2
- Page 719 and 720: E.5. PROJECTION EXAMPLES 719where Y
- Page 721 and 722: E.5. PROJECTION EXAMPLES 721(B.4.2)
- Page 723 and 724: E.6. VECTORIZATION INTERPRETATION,
- Page 725 and 726: E.6. VECTORIZATION INTERPRETATION,
- Page 727 and 728: E.6. VECTORIZATION INTERPRETATION,
- Page 729 and 730: E.7. PROJECTION ON MATRIX SUBSPACES
- Page 731 and 732: E.7. PROJECTION ON MATRIX SUBSPACES
- Page 733 and 734: E.8. RANGE/ROWSPACE INTERPRETATION
- Page 735 and 736: E.9. PROJECTION ON CONVEX SET 735As
- Page 737 and 738: E.9. PROJECTION ON CONVEX SET 737Wi
- Page 739 and 740: E.9. PROJECTION ON CONVEX SET 739R(
- Page 741 and 742: E.9. PROJECTION ON CONVEX SET 741E.
- Page 743 and 744: E.9. PROJECTION ON CONVEX SET 743E.
- Page 745: E.9. PROJECTION ON CONVEX SET 745Un
- Page 749 and 750: E.10. ALTERNATING PROJECTION 749bC
- Page 751 and 752: E.10. ALTERNATING PROJECTION 7510
- Page 753 and 754: E.10. ALTERNATING PROJECTION 753E.1
- Page 755 and 756: E.10. ALTERNATING PROJECTION 755y 2
- Page 757 and 758: E.10. ALTERNATING PROJECTION 757Def
- Page 759 and 760: E.10. ALTERNATING PROJECTION 759Dis
- Page 761 and 762: E.10. ALTERNATING PROJECTION 761mat
- Page 763 and 764: E.10. ALTERNATING PROJECTION 763K
- Page 765 and 766: E.10. ALTERNATING PROJECTION 765E.1
- Page 767 and 768: E.10. ALTERNATING PROJECTION 767E.1
- Page 769 and 770: Appendix FNotation and a few defini
- Page 771 and 772: 771A ij or A(i, j) , ij th entry of
- Page 773 and 774: 773⊞orthogonal vector sum of sets
- Page 775 and 776: 775x +vector x whose negative entri
- Page 777 and 778: 777X point list ((76) having cardin
- Page 779 and 780: 779SDPSVDSNRdBEDMS n 1S n hS n⊥hS
- Page 781 and 782: 781vectorentrycubixquartixfeasible
- Page 783 and 784: 783Oorder of magnitude information
- Page 785 and 786: 785cofmatrix of cofactors correspon
- Page 787 and 788: Bibliography[1] Edwin A. Abbott. Fl
- Page 789 and 790: BIBLIOGRAPHY 789[23] Dror Baron, Mi
- Page 791 and 792: BIBLIOGRAPHY 791[49] Leonard M. Blu
- Page 793 and 794: BIBLIOGRAPHY 793[74] Yves Chabrilla
- Page 795 and 796: BIBLIOGRAPHY 795[102] Etienne de Kl
E.9. PROJECTION ON CONVEX SET 747accomplished by first projecting orthogonally on that subspace, and thenprojecting the result on C ; [109,5.14] id est, the ordered product of twoindividual projections that is not commutable.Proof. (⇐) To show that, suppose unique minimum-distance projectionP C x on C ⊂ R n is y as illustrated in Figure 166;‖x − y‖ ≤ ‖x − q‖ ∀q ∈ C (2043)Further suppose P Rn x equals z . By the Pythagorean theorem‖x − y‖ 2 = ‖x − z‖ 2 + ‖z − y‖ 2 (2044)because x − z ⊥ z − y . (1907) [250,3.3] Then point y = P C x is the sameas P C z because‖z − y‖ 2 = ‖x − y‖ 2 − ‖x − z‖ 2 ≤ ‖z − q‖ 2 = ‖x − q‖ 2 − ‖x − z‖ 2which holds by assumption (2043).(⇒) Now suppose z = P Rn x and∀q ∈ C(2045)‖z − y‖ ≤ ‖z − q‖ ∀q ∈ C (2046)meaning y = P C z . Then point y is identical to P C x because‖x − y‖ 2 = ‖x − z‖ 2 + ‖z − y‖ 2 ≤ ‖x − q‖ 2 = ‖x − z‖ 2 + ‖z − q‖ 2by assumption (2046).∀q ∈ C(2047)This proof is extensible via translation argument. (E.4) Uniqueminimum-distance projection on a convex set contained in an affine subsetis, therefore, similarly accomplished.Projecting matrix H ∈ R n×n on convex cone K = S n ∩ R n×n+ in isomorphicR n2 can be accomplished, for example, by first projecting on S n and only thenprojecting the result on R n×n+ (confer7.0.1). This is because that projectionproduct is equivalent to projection on the subset of the nonnegative orthantin the symmetric matrix subspace.