v2010.10.26 - Convex Optimization

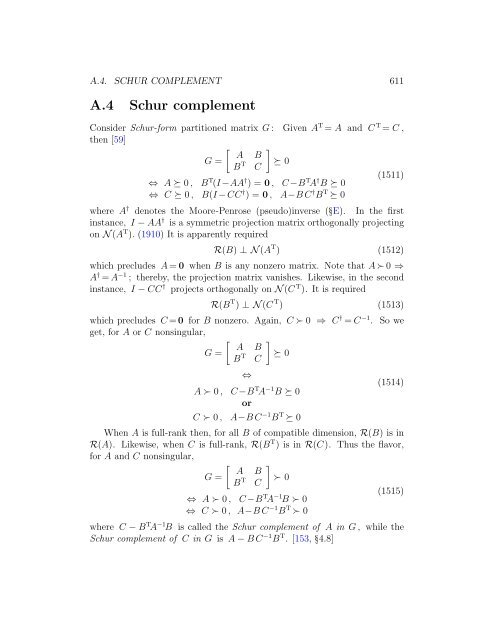
A.4. SCHUR COMPLEMENT 611A.4 Schur complementConsider Schur-form partitioned matrix G : Given A T = A and C T = C ,then [59][ ] A BG =B T ≽ 0C(1511)⇔ A ≽ 0, B T (I −AA † ) = 0, C −B T A † B ≽ 0⇔ C ≽ 0, B(I −CC † ) = 0, A−BC † B T ≽ 0where A † denotes the Moore-Penrose (pseudo)inverse (E). In the firstinstance, I − AA † is a symmetric projection matrix orthogonally projectingon N(A T ). (1910) It is apparently requiredR(B) ⊥ N(A T ) (1512)which precludes A = 0 when B is any nonzero matrix. Note that A ≻ 0 ⇒A † =A −1 ; thereby, the projection matrix vanishes. Likewise, in the secondinstance, I − CC † projects orthogonally on N(C T ). It is requiredR(B T ) ⊥ N(C T ) (1513)which precludes C =0 for B nonzero. Again, C ≻ 0 ⇒ C † = C −1 . So weget, for A or C nonsingular,[ ] A BG =B T ≽ 0C⇔A ≻ 0, C −B T A −1 B ≽ 0orC ≻ 0, A−BC −1 B T ≽ 0(1514)When A is full-rank then, for all B of compatible dimension, R(B) is inR(A). Likewise, when C is full-rank, R(B T ) is in R(C). Thus the flavor,for A and C nonsingular,[ ] A BG =B T ≻ 0C⇔ A ≻ 0, C −B T A −1 B ≻ 0⇔ C ≻ 0, A−BC −1 B T ≻ 0(1515)where C − B T A −1 B is called the Schur complement of A in G , while theSchur complement of C in G is A − BC −1 B T . [153,4.8]