v2010.10.26 - Convex Optimization
v2010.10.26 - Convex Optimization v2010.10.26 - Convex Optimization
492 CHAPTER 5. EUCLIDEAN DISTANCE MATRIXc i ===[ ]−1 0 12 N−2 (N −2)! 2 det T1 −D i(−1) N2 N−2 (N −2)! 2 detD i(1167)(1 T D −1i 1 ) (1168)(−1) N2 N−2 (N −2)! 2 1T cof(D i ) T 1 (1169)where D i is the i th principal N −1×N −1 submatrix 5.62 of D ∈ EDM N ,and cof(D i ) is the N −1×N −1 matrix of cofactors [331,4] correspondingto D i . The number of principal 3 × 3 submatrices in D is, of course, equalto the number of triangular facets in the tetrahedron; four (N!/(3!(N −3)!))when N = 4.5.14.3.1.1 Exercise. Sufficiency conditions for an EDM of four points.Triangle inequality (property 4) and area inequality (1165) are conditionsnecessary for D to be an EDM. Prove their sufficiency in conjunction withthe remaining three Euclidean metric properties.5.14.3.2 N = 5Moving to the next level, we might encounter a Euclidean body calledpolychoron: a bounded polyhedron in four dimensions. 5.63 Our polychoronhas five (N!/(4!(N −4)!)) facets, each of them a general tetrahedron whosevolume-square c i is calculated using the same formula; (1167) whereD is the EDM corresponding to the polychoron, and D i is the EDMcorresponding to the i th facet (the principal 4 × 4 submatrix of D ∈ EDM Ncorresponding to the i th tetrahedron). The analogue to triangle & distanceis now polychoron & facet-volume. We could then write another generalized“triangle” inequality like (1165) but in terms of facet volume; [379,IV]√ci ≤ √ c j + √ c k + √ c l + √ c m , i≠j ≠k≠l≠m∈{1... 5} (1170)5.62 Every principal submatrix of an EDM remains an EDM. [235,4.1]5.63 The simplest polychoron is called a pentatope [373]; a regular simplex hence convex.(A pentahedron is a three-dimensional body having five vertices.)
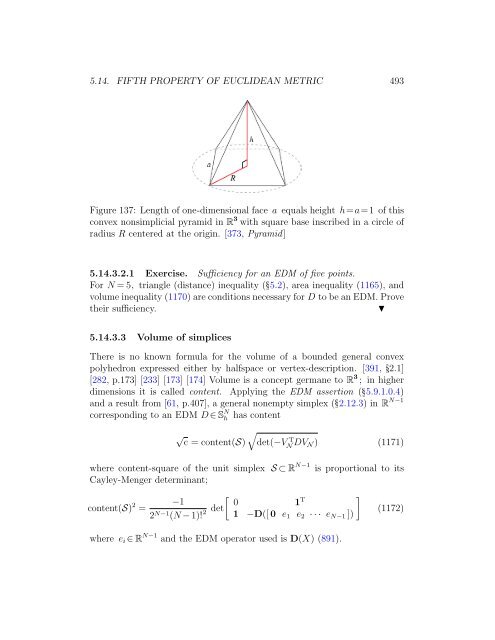
5.14. FIFTH PROPERTY OF EUCLIDEAN METRIC 493.Figure 137: Length of one-dimensional face a equals height h=a=1 of thisconvex nonsimplicial pyramid in R 3 with square base inscribed in a circle ofradius R centered at the origin. [373, Pyramid]5.14.3.2.1 Exercise. Sufficiency for an EDM of five points.For N = 5, triangle (distance) inequality (5.2), area inequality (1165), andvolume inequality (1170) are conditions necessary for D to be an EDM. Provetheir sufficiency.5.14.3.3 Volume of simplicesThere is no known formula for the volume of a bounded general convexpolyhedron expressed either by halfspace or vertex-description. [391,2.1][282, p.173] [233] [173] [174] Volume is a concept germane to R 3 ; in higherdimensions it is called content. Applying the EDM assertion (5.9.1.0.4)and a result from [61, p.407], a general nonempty simplex (2.12.3) in R N−1corresponding to an EDM D ∈ S N h has content√ c = content(S)√det(−V T N DV N) (1171)where content-square of the unit simplex S ⊂ R N−1 is proportional to itsCayley-Menger determinant;content(S) 2 =[ ]−1 0 12 N−1 (N −1)! 2 det T1 −D([0 e 1 e 2 · · · e N−1 ])where e i ∈ R N−1 and the EDM operator used is D(X) (891).(1172)
- Page 441 and 442: 5.6. INJECTIVITY OF D & UNIQUE RECO
- Page 443 and 444: 5.6. INJECTIVITY OF D & UNIQUE RECO
- Page 445 and 446: 5.7. EMBEDDING IN AFFINE HULL 4455.
- Page 447 and 448: 5.7. EMBEDDING IN AFFINE HULL 447Fo
- Page 449 and 450: 5.7. EMBEDDING IN AFFINE HULL 4495.
- Page 451 and 452: 5.8. EUCLIDEAN METRIC VERSUS MATRIX
- Page 453 and 454: 5.8. EUCLIDEAN METRIC VERSUS MATRIX
- Page 455 and 456: 5.8. EUCLIDEAN METRIC VERSUS MATRIX
- Page 457 and 458: 5.8. EUCLIDEAN METRIC VERSUS MATRIX
- Page 459 and 460: 5.9. BRIDGE: CONVEX POLYHEDRA TO ED
- Page 461 and 462: 5.9. BRIDGE: CONVEX POLYHEDRA TO ED
- Page 463 and 464: 5.9. BRIDGE: CONVEX POLYHEDRA TO ED
- Page 465 and 466: 5.10. EDM-ENTRY COMPOSITION 465of a
- Page 467 and 468: 5.10. EDM-ENTRY COMPOSITION 467Then
- Page 469 and 470: 5.11. EDM INDEFINITENESS 4695.11.1
- Page 471 and 472: 5.11. EDM INDEFINITENESS 471we have
- Page 473 and 474: 5.11. EDM INDEFINITENESS 473So beca
- Page 475 and 476: 5.11. EDM INDEFINITENESS 475holds o
- Page 477 and 478: 5.12. LIST RECONSTRUCTION 477where
- Page 479 and 480: 5.12. LIST RECONSTRUCTION 479(a)(c)
- Page 481 and 482: 5.13. RECONSTRUCTION EXAMPLES 481Wi
- Page 483 and 484: 5.13. RECONSTRUCTION EXAMPLES 483d
- Page 485 and 486: 5.13. RECONSTRUCTION EXAMPLES 485Th
- Page 487 and 488: 5.14. FIFTH PROPERTY OF EUCLIDEAN M
- Page 489 and 490: 5.14. FIFTH PROPERTY OF EUCLIDEAN M
- Page 491: 5.14. FIFTH PROPERTY OF EUCLIDEAN M
- Page 495 and 496: 5.14. FIFTH PROPERTY OF EUCLIDEAN M
- Page 497 and 498: Chapter 6Cone of distance matricesF
- Page 499 and 500: 6.1. DEFINING EDM CONE 4996.1 Defin
- Page 501 and 502: 6.2. POLYHEDRAL BOUNDS 501This cone
- Page 503 and 504: 6.4. EDM DEFINITION IN 11 T 503That
- Page 505 and 506: 6.4. EDM DEFINITION IN 11 T 505N(1
- Page 507 and 508: 6.4. EDM DEFINITION IN 11 T 507Then
- Page 509 and 510: 6.4. EDM DEFINITION IN 11 T 5096.4.
- Page 511 and 512: 6.5. CORRESPONDENCE TO PSD CONE S N
- Page 513 and 514: 6.5. CORRESPONDENCE TO PSD CONE S N
- Page 515 and 516: 6.5. CORRESPONDENCE TO PSD CONE S N
- Page 517 and 518: 6.6. VECTORIZATION & PROJECTION INT
- Page 519 and 520: 6.6. VECTORIZATION & PROJECTION INT
- Page 521 and 522: 6.6. VECTORIZATION & PROJECTION INT
- Page 523 and 524: 6.7. A GEOMETRY OF COMPLETION 523(b
- Page 525 and 526: 6.7. A GEOMETRY OF COMPLETION 525[3
- Page 527 and 528: 6.7. A GEOMETRY OF COMPLETION 527wh
- Page 529 and 530: 6.8. DUAL EDM CONE 529to the geomet
- Page 531 and 532: 6.8. DUAL EDM CONE 531Proof. First,
- Page 533 and 534: 6.8. DUAL EDM CONE 533EDM 2 = S 2 h
- Page 535 and 536: 6.8. DUAL EDM CONE 535therefore the
- Page 537 and 538: 6.8. DUAL EDM CONE 537Elegance of t
- Page 539 and 540: 6.8. DUAL EDM CONE 5396.8.1.5 Affin
- Page 541 and 542: 6.8. DUAL EDM CONE 5416.8.1.7 Schoe
5.14. FIFTH PROPERTY OF EUCLIDEAN METRIC 493.Figure 137: Length of one-dimensional face a equals height h=a=1 of thisconvex nonsimplicial pyramid in R 3 with square base inscribed in a circle ofradius R centered at the origin. [373, Pyramid]5.14.3.2.1 Exercise. Sufficiency for an EDM of five points.For N = 5, triangle (distance) inequality (5.2), area inequality (1165), andvolume inequality (1170) are conditions necessary for D to be an EDM. Provetheir sufficiency.5.14.3.3 Volume of simplicesThere is no known formula for the volume of a bounded general convexpolyhedron expressed either by halfspace or vertex-description. [391,2.1][282, p.173] [233] [173] [174] Volume is a concept germane to R 3 ; in higherdimensions it is called content. Applying the EDM assertion (5.9.1.0.4)and a result from [61, p.407], a general nonempty simplex (2.12.3) in R N−1corresponding to an EDM D ∈ S N h has content√ c = content(S)√det(−V T N DV N) (1171)where content-square of the unit simplex S ⊂ R N−1 is proportional to itsCayley-Menger determinant;content(S) 2 =[ ]−1 0 12 N−1 (N −1)! 2 det T1 −D([0 e 1 e 2 · · · e N−1 ])where e i ∈ R N−1 and the EDM operator used is D(X) (891).(1172)