v2010.10.26 - Convex Optimization
Share Embed Flag
Download ePaper

v2010.10.26 - Convex Optimization

v2010.10.26 - Convex Optimization v2010.10.26 - Convex Optimization

convexoptimization.com
from convexoptimization.com More from this publisher
12.07.2015 Views

458 CHAPTER 5. EUCLIDEAN DISTANCE MATRIX5.9 Bridge: Convex polyhedra to EDMsThe criteria for the existence of an EDM include, by definition (891) (958),the properties imposed upon its entries d ij by the Euclidean metric. From5.8.1 and5.8.2, we know there is a relationship of matrix criteria to thoseproperties. Here is a snapshot of what we are sure: for i , j , k ∈{1... N}(confer5.2)√dij ≥ 0, i ≠ j√dij = 0, i = j√dij = √ d ji √dij≤ √ d ik + √ d kj , i≠j ≠k⇐−V T N DV N ≽ 0δ(D) = 0D T = D(1074)all implied by D ∈ EDM N . In words, these four Euclidean metric propertiesare necessary conditions for D to be a distance matrix. At the moment,we have no converse. As of concern in5.3, we have yet to establishmetric requirements beyond the four Euclidean metric properties that wouldallow D to be certified an EDM or might facilitate polyhedron or listreconstruction from an incomplete EDM. We deal with this problem in5.14.Our present goal is to establish ab initio the necessary and sufficient matrixcriteria that will subsume all the Euclidean metric properties and any furtherrequirements 5.44 for all N >1 (5.8.3); id est,−V T N DV N ≽ 0D ∈ S N h}⇔ D ∈ EDM N (910)or for EDM definition (967),}Ω ≽ 0√δ(d) ≽ 0⇔ D = D(Ω,d) ∈ EDM N (1075)5.44 In 1935, Schoenberg [312, (1)] first extolled matrix product −VN TDV N (1053)(predicated on symmetry and self-distance) specifically incorporating V N , albeitalgebraically. He showed: nonnegativity −y T VN TDV N y ≥ 0, for all y ∈ R N−1 , is necessaryand sufficient for D to be an EDM. Gower [162,3] remarks how surprising it is that sucha fundamental property of Euclidean geometry was obtained so late.

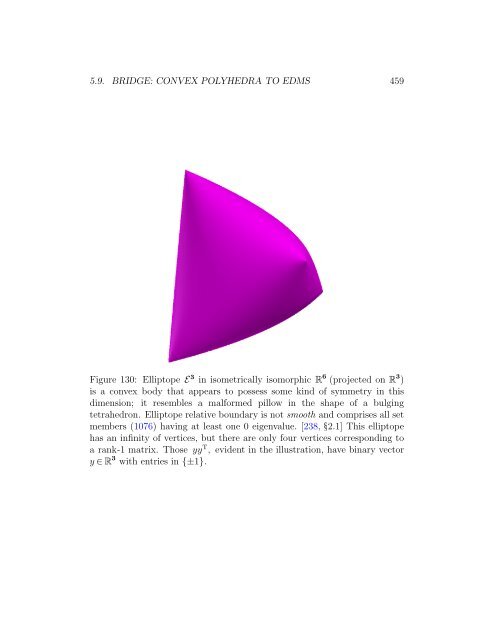
5.9. BRIDGE: CONVEX POLYHEDRA TO EDMS 459Figure 130: Elliptope E 3 in isometrically isomorphic R 6 (projected on R 3 )is a convex body that appears to possess some kind of symmetry in thisdimension; it resembles a malformed pillow in the shape of a bulgingtetrahedron. Elliptope relative boundary is not smooth and comprises all setmembers (1076) having at least one 0 eigenvalue. [238,2.1] This elliptopehas an infinity of vertices, but there are only four vertices corresponding toa rank-1 matrix. Those yy T , evident in the illustration, have binary vectory ∈ R 3 with entries in {±1}.

5.9. BRIDGE: CONVEX POLYHEDRA TO EDMS 459Figure 130: Elliptope E 3 in isometrically isomorphic R 6 (projected on R 3 )is a convex body that appears to possess some kind of symmetry in thisdimension; it resembles a malformed pillow in the shape of a bulgingtetrahedron. Elliptope relative boundary is not smooth and comprises all setmembers (1076) having at least one 0 eigenvalue. [238,2.1] This elliptopehas an infinity of vertices, but there are only four vertices corresponding toa rank-1 matrix. Those yy T , evident in the illustration, have binary vectory ∈ R 3 with entries in {±1}.

logo

products

Resources

Company

Terms of service
Privacy policy
Cookie policy
Cookie settings
Imprint
Change language
Made with love in Switzerland
© 2024 Yumpu.com all rights reserved

Hooray! Your file is uploaded and ready to be published.

Saved successfully!

Ooh no, something went wrong!