v2010.10.26 - Convex Optimization

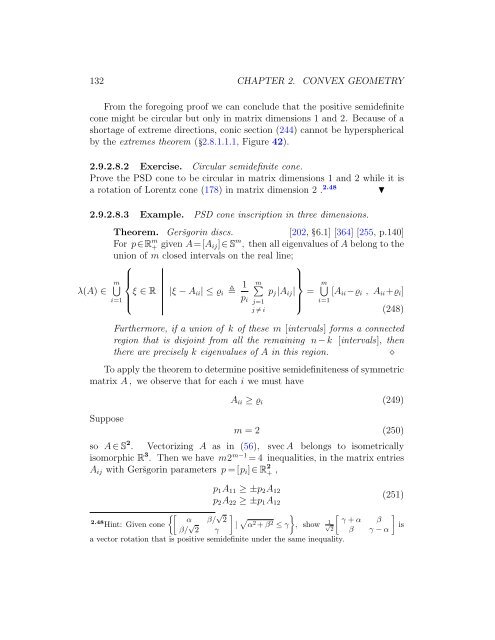
132 CHAPTER 2. CONVEX GEOMETRYFrom the foregoing proof we can conclude that the positive semidefinitecone might be circular but only in matrix dimensions 1 and 2. Because of ashortage of extreme directions, conic section (244) cannot be hypersphericalby the extremes theorem (2.8.1.1.1, Figure 42).2.9.2.8.2 Exercise. Circular semidefinite cone.Prove the PSD cone to be circular in matrix dimensions 1 and 2 while it isa rotation of Lorentz cone (178) in matrix dimension 2 . 2.48 2.9.2.8.3 Example. PSD cone inscription in three dimensions.Theorem. Geršgorin discs. [202,6.1] [364] [255, p.140]For p∈R m + given A=[A ij ]∈ S m , then all eigenvalues of A belong to theunion of m closed intervals on the real line;⎧⎫⋃⎪⎨λ(A) ∈ m ξ ∈ R |ξ − A ii | ≤ ̺i 1 m∑⎪⎬ ⋃p j |A ij | = m [A ii −̺i , A ii +̺i]i=1p ⎪⎩i j=1i=1 ⎪⎭j ≠ i(248)Furthermore, if a union of k of these m [intervals] forms a connectedregion that is disjoint from all the remaining n −k [intervals], thenthere are precisely k eigenvalues of A in this region.⋄To apply the theorem to determine positive semidefiniteness of symmetricmatrix A , we observe that for each i we must haveSupposeA ii ≥ ̺i (249)m = 2 (250)so A ∈ S 2 . Vectorizing A as in (56), svec A belongs to isometricallyisomorphic R 3 . Then we have m2 m−1 = 4 inequalities, in the matrix entriesA ij with Geršgorin parameters p =[p i ]∈ R 2 + ,p 1 A 11 ≥ ±p 2 A 12p 2 A 22 ≥ ±p 1 A 12(251){[2.48 α β/ √ ]2Hint: Given coneβ/ √ | √ } [ ]α2 γ2 + β 2 ≤ γ , show √ γ + α β12isβ γ − αa vector rotation that is positive semidefinite under the same inequality.