+ 2 - Math with JM - home

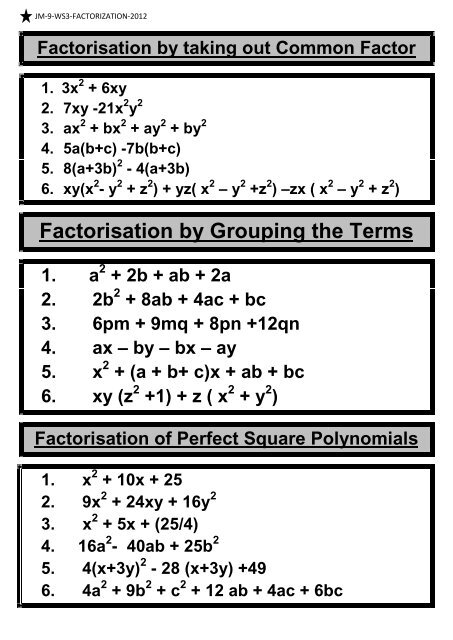
JM-9-WS3-FACTORIZATION-2012Factorisation by taking out Common Factor1. 3x 2 + 6xy2. 7xy -21x 2 y 23. ax 2 + bx 2 + ay 2 + by 24. 5a(b+c) -7b(b+c)5. 8(a+3b) 2 - 4(a+3b)6. xy(x 2 - y 2 + z 2 ) + yz( x 2 – y 2 +z 2 ) –zx ( x 2 – y 2 + z 2 )Factorisation by Grouping the Terms1. a 2 + 2b + ab + 2a2. 2b 2 + 8ab + 4ac + bc3. 6pm + 9mq + 8pn +12qn4. ax – by – bx – ay5. x 2 + (a + b+ c)x + ab + bc6. xy (z 2 +1) + z ( x 2 + y 2 )Factorisation of Perfect Square Polynomials1. x 2 + 10x + 252. 9x 2 + 24xy + 16y 23. x 2 + 5x + (25/4)4. 16a 2 - 40ab + 25b 25. 4(x+3y) 2 - 28 (x+3y) +496. 4a 2 + 9b 2 + c 2 + 12 ab + 4ac + 6bc

RAPID COMMUNICATIONSJ. FIURÁŠEK, S. IBLISDIR, S. MASSAR, AND N. J. CERF PHYSICAL REVIEW A 65 040302RWe will measure the quality of the transformation by theaverage single-clone fidelity F (M). Denoting by Tr 1,anc thepartial trace over the ancilla and all the clones but the firstone, we getwhereF M d Tr 1,anc out out i jk ijkR i jk R i jkijkA ijk ,Ai jkijk n,n nTr 1 M,kM,knd d n0 d n*0d i0 d j1 d i*0d* j 1.The coefficients Ai jkijk can be considered as matrix elementsof an operator A acting on the space H K, where H denotesthe Hilbert space of the two input qubits and K denotes theHilbert space of symmetric states of M output qubits. Similarly,i jkijk Rijk R i jk define matrix elements of an operator also acting on H K. The formula 4 for the fidelitythus simplifies to F (M)Tr H,K A. The operator uniquely represents the completely positive cloning map,which transforms operators supported on H onto operatorssupported on K. By definition, the operators A and areHermitian and positive semidefinite, A0 and 0.Of course, the transformation 1 should be unitary, whichreads k R i jkR ijk i i j j . This is equivalent to4 j,M 1 j 12M1 3M2 j2MM1M2,with j,(M j) denoting a totally symmetric state of Mqubits where j qubits are in state and M j qubits are instate . The first M output qubits contain the clones ofstate while the other M qubits contain the clones of or anticlones. We shall prove below that this transformationis indeed optimal.We stress here that the cloning transformation 8 is unitary.Since this is by no means obvious from Eq. 8, let uspresent a proof of this. We can expand any state j,(M j) in the basis M,k asM j,M j k0e i( jk) D M kj M,k.910We will not need an explicit expression for the functionsD M kj () here, but will only use some of their properties.Since the functions D M kj () are elements of a real unitarymatrix, they satisfy the orthogonality relation,Mj0D M kj D M lj kl .We will also use the following recurrence formula 11:2 jM D M kj 2kM cos D M kj 11Tr K 1 H ,5Msin k1Mk D k1,j where 1 H is the identity operator on H. Thus, introducing aset of Lagrange multipliers i jij for these unitarity constraints,our problem amounts to extremize the quantity WTr H,K (A) under the constraint 0, where 1 K and is the matrix of Lagrange multipliers (1 K is theidentity operator on K). Varying W with respect to the eigenstatesof the operator , we get the extremal equationA0for the optimal . Following 10, this equation can be furthertransformed into a form suitable for numerical solutionvia repeated applications of6 1 AA 1 , Tr K AA 1/2 . 7Note that the matrix 0 is determined from the unitarityconstraints.By numerically solving Eq. 7 for M2,...,15, wehave guessed the general solution of Eq. 7. The transformationwe obtain isM, → j,M M j, j M j , j,j08Msin kMk1 D k1,j .12For the purpose of the proof it is convenient to apply(i y ) M on the last M qubits at the output of the cloner.Thus, (M j) , j→(1) j (M j)*, j * where* i d i0*i. Next we expand (M j), j and (M j)*, j * in the basis M,k according to Eq. 10, andthen utilize the recurrence formula 12. Finally, we cancarry out the sum over j with the help of Eq. 11, resulting inM out k0k0a M b M 2kM cos 2 M,k M,k2Ma M b M 2kM sin 2 M,k M,k2Me i k0 b MMkk1sin M,kMM,k1e i k0 b MkMk1sin M,k M,k1,13where040302-2where the coefficients a M and b M read