May 2011 - Career Point
May 2011 - Career Point May 2011 - Career Point
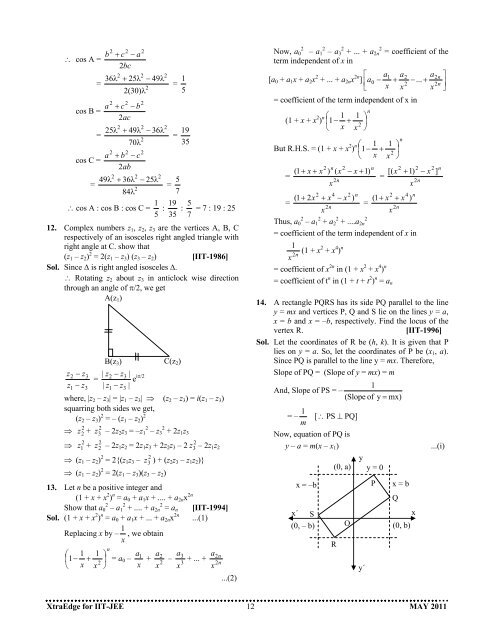
∴ cos A ==cos B ==cos C ==b22+ c − a2bc236λa2+ 25λ2222(30) λ2+ c − b2ac225λa2249λ222+ 49λ270λ+ b − c2ab22+ 36λ22− 49λ2− 36λ2− 25λ= 5119= 35= 7584λ1 19 5∴ cos A : cos B : cos C = : : = 7 : 19 : 255 35 712. Complex numbers z 1 , z 2 , z 3 are the vertices A, B, Crespectively of an isosceles right angled triangle withright angle at C. show that(z 1 – z 2 ) 2 = 2(z 1 – z 3 ) (z 3 – z 2 ) [IIT-1986]Sol. Since ∆ is right angled isosceles ∆.∴ Rotating z 2 about z 3 in anticlock wise directionthrough an angle of π/2, we getA(z 1 )B(z 3 )C(z 2 )z2− z3| z2− z3|= e iπ/2z1− z3| z1− z3|where, |z 2 – z 3 | = |z 1 – z 3 | ⇒ (z 2 – z 3 ) = i(z 1 – z 3 )squarring both sides we get,(z 2 – z 3 ) 2 = – (z 1 – z 2 ) 22 2⇒ z 2 + z 3 – 2z 2 z 3 = –z 2 1 – z 2 3 + 2z 1 z 32 2⇒ z 1 + z 2 – 2z 1 z 2 = 2z 1 z 3 + 2z 2 z 3 – 2 z 2 3 – 2z 1 z 2⇒ (z 1 – z 2 ) 2 2= 2{(z 1 z 3 – z 3 ) + (z 2 z 3 – z 1 z 2 )}⇒ (z 1 – z 2 ) 2 = 2(z 1 – z 3 )(z 3 – z 2 )13. Let n be a positive integer and(1 + x + x 2 ) n = a 0 + a 1 x + .... + a 2n x 2nShow that a 2 0 – a 2 1 + .... + a 2 2n = a n [IIT-1994]Sol. (1 + x + x 2 ) n = a 0 + a 1 x + ... + a 2n x 2n ...(1)Replacing x by – x1 , we obtain⎛ 1 1 ⎞⎜1 − + ⎟ = a⎝ x2 0 –x ⎠na 1 a +2x2x–a 3 a3 + ... +2n2nx x...(2)2Now, a 0 – a 2 1 – a 2 3 + ... + a 2 2n = coefficient of theterm independent of x in[a 0 + a 1 x + a 2 x 2 + ... + a 2n x 2n ⎡ a1a2a2n⎤] ⎢a0 − + −...+n ⎥⎣ x2 2x x ⎦= coefficient of the term independent of x inn(1 + x + x 2 ) n ⎛ 1 1 ⎞⎜1− + ⎟⎝ x2x ⎠But R.H.S. = (1 + x + x 2 ) n ⎛ 1⎜1− +⎝ x x=2n( 1+x + x ) ( x − x + 1)2x2n422nn12n⎞⎟⎠2[( x + 1) − x ]=2nx( 1+2x+ x − x ) ( 1+x + x )==2n2nxxThus, a 2 0 – a 2 1 + a 2 22 + ....a 2n= coefficient of the term independent of x in1n (1 + x 2 + x 4 ) nx 2= coefficient of x 2n in (1 + x 2 + x 4 ) n= coefficient of t n in (1 + t + t 2 ) n = a n14. A rectangle PQRS has its side PQ parallel to the liney = mx and vertices P, Q and S lie on the lines y = a,x = b and x = –b, respectively. Find the locus of thevertex R.[IIT-1996]Sol. Let the coordinates of R be (h, k). It is given that Plies on y = a. So, let the coordinates of P be (x 1 , a).Since PQ is parallel to the line y = mx. Therefore,Slope of PQ = (Slope of y = mx) = mAnd, Slope of PS = –= – m1[∴ PS ⊥ PQ]Now, equation of PQ isy – a = m(x – x 1 )x = –bx´ S(0, – b)(0, a)R1(Slope of y = mx)Oyy = 0y´P24x = bQ(0, b)nx22n...(i)XtraEdge for IIT-JEE 12 MAY 2011
It is given that Q lies on x = b. So, Q is the point ofintersection if (i) and x = b.Putting x = b in (i), we gety = a + m(b – x 1 )So, coordinates of Q are (b, a + m(b – x 1 )).Since PS passes through P(x 1 , a) and has slope – m1 .So, Equation of PS isy – a = – m1 (x – x1 )...(ii)It is given that S lies on x = – b. So, S is the point ofintersection of (ii) and x = –b.Solving (ii) and x = – b, we gety = a + m1 (b + x1 )⎛ 1 ⎞So, coordinates of S are ⎜− b , a + ( b + x1)⎟⎝ m ⎠1k − a − ( b + x1)Now, Slope of RS =m= mh + bBut RS is parallel to PQ.1k − a − ( b + x1)∴m= mh + b⇒ b + x 1 = m(k – a) – m 2 (h + b) ...(iii)Similarly,k − a − m( b − x1)Slope of RQ =h − bBut, RQ is perpendicular to PQ whose slope is m.∴k − a − m( b − x1 ) 1= –h − b m1 1⇒ b – x 1 = (k – a) + (h – a) ...(iv)m2mWe have only one variable x 1 . To eliminate x 1 , add(iii) and (iv) to obtain⎛ 1 ⎞2b = (k – a) ⎜m + ⎟ – m 2 (h + b) +⎝ m ⎠12m(h – b)⎛2⎞⇒ 2b = (k – a) ⎜m + 1 ⎛4⎞⎟– h ⎜m + 1 ⎛4⎞⎟⎝ m2– b ⎜m + 1⎟⎠ ⎝ m2⎠ ⎝ m ⎠⎛2⎞⇒ (k – a) ⎜m + 1⎟–⎝ m ⎠2h ( m −1)(m + 1)2m–22b ( m + 1)2m2= 02 −2 +h ( m 1)b ( m 1)⇒ (k – a) – – = 0m m⇒ m(k – a) – h(m 2 – 1) – b(m 2 + 1) = 0Hence, the locus of R(h, k) ism(y – a) – x(m 2 – 1) – b(m 2 + 1) = 015. Let f [(x + y)/2] = {f (x) + f (y)} / 2 for all real x and y,If f ´(0) exists and equals –1 and f (0) = 1, find f (2).[IIT- 1995]⎛ x + y ⎞ f ( x)+ f ( y)Sol. f ⎜ ⎟ =∀ x, y ∈ R (given)⎝ 2 ⎠ 2Putting y = 0, we get⎛ x ⎞ f ( x)+ f (0) 1f ⎜ ⎟ == [1 + f (x)] [Q f (0) = 1]⎝ 2 ⎠ 2 2⇒ 2f (x/2) = f (x) + 1⇒ f (x) = 2f (x/2) – 1 ∀x, y ∈ R ...(1)Since f´(0) = –1, we getf (0 + h)− f (0)f ( h)−1lim = – 1 ⇒ lim = –1h→0 hh→0 hNow, let x ∈ R then applying formula ofdifferentiability.⎛ 2x+ 2h⎞f ⎜ ⎟ − f ( x)f ( x + h)− f ( x)2f ´(x) = lim = lim⎝ ⎠h→0 hh→0 hf (2x)+ f (2h)− f ( x)= lim2h→0h1 ⎧ ⎛ 2x⎞ ⎛ 2h⎞ ⎫⎨2f ⎜ ⎟ −1+2 f ⎜ ⎟ −1⎬− f ( x)2 22= lim⎩ ⎝ ⎠ ⎝ ⎠ ⎭h→0h[Using equations (1)1{2 f ( x)−1+2 f ( h)−1}− f ( x)= lim2h→0hf ( x)+ f ( h)−1−f ( x)= limh→0hf ( h)−1= lim = –1h→0 hTherefore f ´(x) = – 1 ∀ x ∈ R⇒∫f ´( x)dx =∫ −1dx⇒ f (x) = –x + k where k is a constant.But f (0) = 1, therefore f (0) = – 0 + k⇒ f (x) = 1 – x ∀ x ∈ R ⇒ f (2) = – 1XtraEdge for IIT-JEE 13 MAY 2011
- Page 3 and 4: Volume - 6 Issue - 11May, 2011 (Mon
- Page 5 and 6: Volume-6 Issue-11May, 2011 (Monthly
- Page 7 and 8: writer, broadcaster and member of t
- Page 9 and 10: KNOW IIT-JEEBy Previous Exam Questi
- Page 11 and 12: 60 cmK 1 K 2VB5. A solid sphere of
- Page 16 and 17: Physics Challenging ProblemsSet # 1
- Page 18 and 19: PHYSICSStudents'ForumExpert’s Sol
- Page 20 and 21: Q 1 = 1200 JEnginel = 21 cma = 6 cm
- Page 22 and 23: PHYSICS FUNDAMENTAL FOR IIT-JEEElec
- Page 24 and 25: the indivual forces. It's often hel
- Page 26 and 27: Hence, E x = -∴∂V∂ xE = - ayi
- Page 28 and 29: For (x - a) 2 + (y - b) 2 = r 2 , t
- Page 30 and 31: (If the velocities are not along th
- Page 32 and 33: KEY CONCEPTPhysicalChemistryFundame
- Page 34 and 35: ⎛ n ⎞ ⎛ n ⎞Correction term
- Page 36 and 37: Kinetic Isotope Effects :The kineti
- Page 38 and 39: (m / 32) 1Moles fraction of O 2 ==(
- Page 40 and 41: `tà{xÅtà|vtÄ V{tÄÄxÇzxá1 Se
- Page 42 and 43: Students' ForumExpert’s Solution
- Page 44 and 45: MATHSCOMPLEX NUMBERMathematics Fund
- Page 46 and 47: MATHSMATRICES &DETERMINANTSMathemat
- Page 48 and 49: aBased on New PatternIIT-JEE 2012Xt
- Page 50 and 51: dxO12. The time of crossing the riv
- Page 52 and 53: 6. Choose the INCORRECT statement f
- Page 54 and 55: 7. If the equation 3x 4 - 16x 3 + 3
- Page 56 and 57: Based on New PatternIIT-JEE 2013Xtr
- Page 58 and 59: 17. A stone is projected from level
- Page 60 and 61: 16. Half a mole of photon is used t
- Page 62 and 63: 17. Ifsin 3θcos 2θ2-147137= 0Then
∴ cos A ==cos B ==cos C ==b22+ c − a2bc236λa2+ 25λ2222(30) λ2+ c − b2ac225λa2249λ222+ 49λ270λ+ b − c2ab22+ 36λ22− 49λ2− 36λ2− 25λ= 5119= 35= 7584λ1 19 5∴ cos A : cos B : cos C = : : = 7 : 19 : 255 35 712. Complex numbers z 1 , z 2 , z 3 are the vertices A, B, Crespectively of an isosceles right angled triangle withright angle at C. show that(z 1 – z 2 ) 2 = 2(z 1 – z 3 ) (z 3 – z 2 ) [IIT-1986]Sol. Since ∆ is right angled isosceles ∆.∴ Rotating z 2 about z 3 in anticlock wise directionthrough an angle of π/2, we getA(z 1 )B(z 3 )C(z 2 )z2− z3| z2− z3|= e iπ/2z1− z3| z1− z3|where, |z 2 – z 3 | = |z 1 – z 3 | ⇒ (z 2 – z 3 ) = i(z 1 – z 3 )squarring both sides we get,(z 2 – z 3 ) 2 = – (z 1 – z 2 ) 22 2⇒ z 2 + z 3 – 2z 2 z 3 = –z 2 1 – z 2 3 + 2z 1 z 32 2⇒ z 1 + z 2 – 2z 1 z 2 = 2z 1 z 3 + 2z 2 z 3 – 2 z 2 3 – 2z 1 z 2⇒ (z 1 – z 2 ) 2 2= 2{(z 1 z 3 – z 3 ) + (z 2 z 3 – z 1 z 2 )}⇒ (z 1 – z 2 ) 2 = 2(z 1 – z 3 )(z 3 – z 2 )13. Let n be a positive integer and(1 + x + x 2 ) n = a 0 + a 1 x + .... + a 2n x 2nShow that a 2 0 – a 2 1 + .... + a 2 2n = a n [IIT-1994]Sol. (1 + x + x 2 ) n = a 0 + a 1 x + ... + a 2n x 2n ...(1)Replacing x by – x1 , we obtain⎛ 1 1 ⎞⎜1 − + ⎟ = a⎝ x2 0 –x ⎠na 1 a +2x2x–a 3 a3 + ... +2n2nx x...(2)2Now, a 0 – a 2 1 – a 2 3 + ... + a 2 2n = coefficient of theterm independent of x in[a 0 + a 1 x + a 2 x 2 + ... + a 2n x 2n ⎡ a1a2a2n⎤] ⎢a0 − + −...+n ⎥⎣ x2 2x x ⎦= coefficient of the term independent of x inn(1 + x + x 2 ) n ⎛ 1 1 ⎞⎜1− + ⎟⎝ x2x ⎠But R.H.S. = (1 + x + x 2 ) n ⎛ 1⎜1− +⎝ x x=2n( 1+x + x ) ( x − x + 1)2x2n422nn12n⎞⎟⎠2[( x + 1) − x ]=2nx( 1+2x+ x − x ) ( 1+x + x )==2n2nxxThus, a 2 0 – a 2 1 + a 2 22 + ....a 2n= coefficient of the term independent of x in1n (1 + x 2 + x 4 ) nx 2= coefficient of x 2n in (1 + x 2 + x 4 ) n= coefficient of t n in (1 + t + t 2 ) n = a n14. A rectangle PQRS has its side PQ parallel to the liney = mx and vertices P, Q and S lie on the lines y = a,x = b and x = –b, respectively. Find the locus of thevertex R.[IIT-1996]Sol. Let the coordinates of R be (h, k). It is given that Plies on y = a. So, let the coordinates of P be (x 1 , a).Since PQ is parallel to the line y = mx. Therefore,Slope of PQ = (Slope of y = mx) = mAnd, Slope of PS = –= – m1[∴ PS ⊥ PQ]Now, equation of PQ isy – a = m(x – x 1 )x = –bx´ S(0, – b)(0, a)R1(Slope of y = mx)Oyy = 0y´P24x = bQ(0, b)nx22n...(i)XtraEdge for IIT-JEE 12 MAY <strong>2011</strong>