Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...

4. Disorder—Results and Limiting Cases
The disordered Bogoliubov problem contains three different length scales:
the excitation wave length λ = 2π/k, the healing length ξ, and the disorder
correlation length σ. The physics is not determined by the absolute values
of these length scales, but rather by their values relative to each other. The
three relevant dimensionless parameters are the following:
• The ratio σ/ξ indicates, whether the disordered condensate is in the
Thomas-Fermi regime (σ ≫ ξ) or in the smoothing regime (σ ≪ ξ),
see subsection 2.2.3.
• The parameter kξ indicates, whether the excitations are sound-wave
like (kξ ≪ 1) or particle like (kξ ≫ 1), see subsection 2.3.1.
• The parameter kσ discriminates the point-scatterer regime (kσ ≪ 1)
from a very smooth scattering potential kσ ≫ 1.
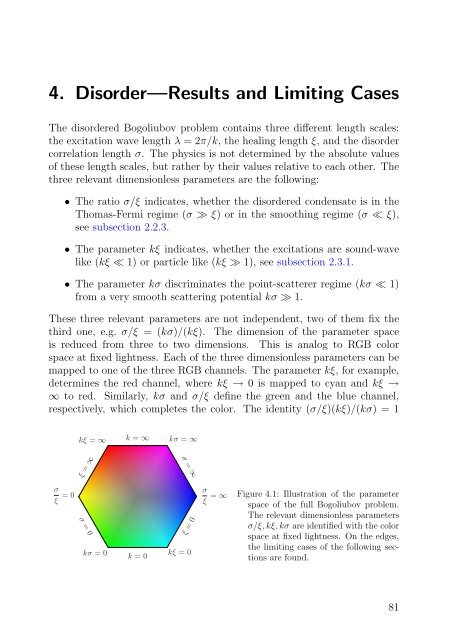
These three relevant parameters are not independent, two of them fix the
third one, e.g. σ/ξ = (kσ)/(kξ). The dimension of the parameter space
is reduced from three to two dimensions. This is analog to RGB color
space at fixed lightness. Each of the three dimensionless parameters can be
mapped to one of the three RGB channels. The parameter kξ, for example,
determines the red channel, where kξ → 0 is mapped to cyan and kξ →
∞ to red. Similarly, kσ and σ/ξ define the green and the blue channel,
respectively, which completes the color. The identity (σ/ξ)(kξ)/(kσ) = 1
σ
ξ
= 0
kξ = ∞
σ = 0
ξ = ∞
kσ = 0
k = ∞
k = 0
kσ = ∞
σ = ∞
ξ = 0
kξ = 0
σ
ξ
= ∞
Figure 4.1: Illustration of the parameter
space of the full Bogoliubov problem.
The relevant dimensionless parameters
σ/ξ, kξ, kσ are identified with the color
space at fixed lightness. On the edges,
the limiting cases of the following sections
are found.
81