Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...

3. Disorder
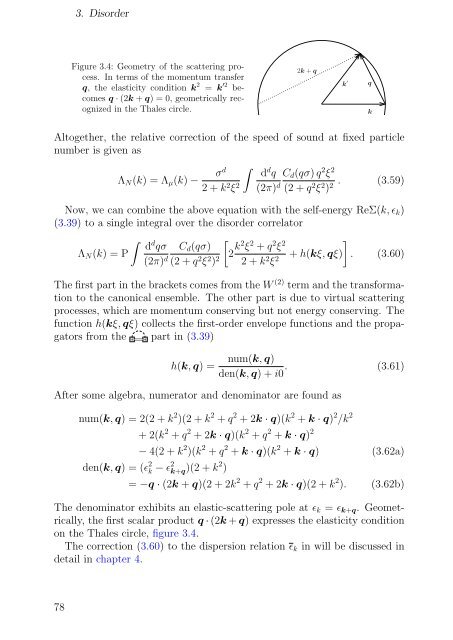
Figure 3.4: Geometry of the scattering process.
In terms of the momentum transfer
q, the elasticity condition k 2 = k ′2 becomes
q · (2k + q) = 0, geometrically recognized
in the Thales circle.
2k + q
Altogether, the relative correction of the speed of sound at fixed particle
number is given as
ΛN(k) = Λµ(k) −
σd
2 + k2ξ2 � d d q
(2π) d
Cd(qσ) q2ξ2 (2 + q2ξ2 . (3.59)
) 2
Now, we can combine the above equation with the self-energy ReΣ(k, ɛk)
(3.39) to a single integral over the disorder correlator
� d d qσ
ΛN(k) = P
(2π) d
Cd(qσ)
(2 + q2ξ2 ) 2
�
2 k2ξ2 + q2ξ2 2 + k2 + h(kξ, qξ)
ξ2 k ′
q
k
�
. (3.60)
The first part in the brackets comes from the W (2) term and the transformation
to the canonical ensemble. The other part is due to virtual scattering
processes, which are momentum conserving but not energy conserving. The
function h(kξ, qξ) collects the first-order envelope functions and the propagators
from the ⊛ ⊛ part in (3.39)
h(k, q) =
num(k, q)
. (3.61)
den(k, q) + i0
After some algebra, numerator and denominator are found as
num(k, q) = 2(2 + k 2 )(2 + k 2 + q 2 + 2k · q)(k 2 + k · q) 2 /k 2
+ 2(k 2 + q 2 + 2k · q)(k 2 + q 2 + k · q) 2
− 4(2 + k 2 )(k 2 + q 2 + k · q)(k 2 + k · q) (3.62a)
den(k, q) = (ɛ 2 k − ɛ 2 k+q)(2 + k 2 )
= −q · (2k + q)(2 + 2k 2 + q 2 + 2k · q)(2 + k 2 ). (3.62b)
The denominator exhibits an elastic-scattering pole at ɛk = ɛk+q. Geometrically,
the first scalar product q · (2k + q) expresses the elasticity condition
on the Thales circle, figure 3.4.
The correction (3.60) to the dispersion relation ɛk in will be discussed in
detail in chapter 4.
78