Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...
Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...
Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
2.5. Exact diagonalization <strong>of</strong> the <strong>Bogoliubov</strong> problem<br />
In this form, the non-condensed density requires the <strong>Bogoliubov</strong> eigenstates<br />
to be computed explicitly. This will be done in the numerical study <strong>of</strong> the<br />
disordered <strong>Bogoliubov</strong> problem in subsection 4.3.3.<br />
Non-condensed density in the homogeneous BEC<br />
In the homogeneous <strong>Bogoliubov</strong> problem (subsection 2.3.1), we use equation<br />
(2.27) and (2.33) and write<br />
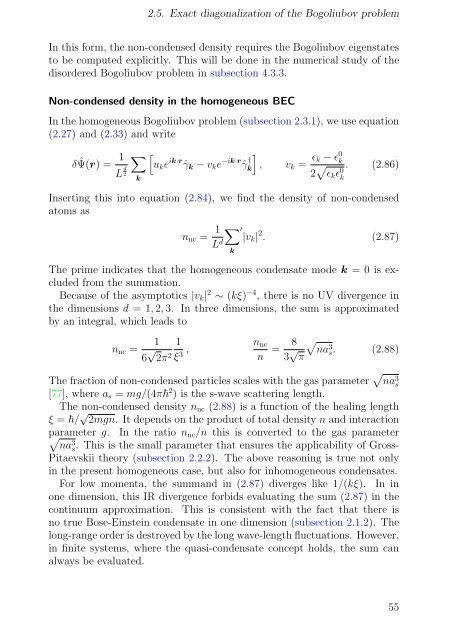
δ ˆ Ψ(r) = 1<br />
L d<br />
2<br />
� �<br />
k<br />
uke ik·r ˆγk − vke −ik·r ˆγ †<br />
k<br />
�<br />
, vk = ɛk − ɛ0 k<br />
2 � ɛkɛ0 . (2.86)<br />
k<br />
Inserting this into equation (2.84), we find the density <strong>of</strong> non-condensed<br />
atoms as<br />
nnc = 1<br />
Ld �′<br />
|vk| 2 . (2.87)<br />
The prime indicates that the homogeneous condensate mode k = 0 is excluded<br />
from the summation.<br />
Because <strong>of</strong> the asymptotics |vk| 2 ∼ (kξ) −4 , there is no UV divergence in<br />
the dimensions d = 1, 2, 3. In three dimensions, the sum is approximated<br />
by an integral, which leads to<br />
nnc =<br />
1<br />
6 √ 2π 2<br />
1<br />
,<br />
ξ3 k<br />
nnc<br />
n<br />
8<br />
=<br />
3 √ �<br />
na3 π<br />
s. (2.88)<br />
The fraction <strong>of</strong> non-condensed particles scales with the gas parameter � na 3 s<br />
[77], where as = mg/(4π� 2 ) is the s-wave scattering length.<br />
The non-condensed density nnc (2.88) is a function <strong>of</strong> the healing length<br />
ξ = �/ √ 2mgn. It depends on the product <strong>of</strong> total density n and interaction<br />
parameter g. In the ratio nnc/n this is converted to the gas parameter<br />
� na 3 s. This is the small parameter that ensures the applicability <strong>of</strong> Gross-<br />
Pitaevskii theory (subsection 2.2.2). The above reasoning is true not only<br />
in the present homogeneous case, but also for inhomogeneous condensates.<br />
For low momenta, the summand in (2.87) diverges like 1/(kξ). In in<br />
one dimension, this IR divergence forbids evaluating the sum (2.87) in the<br />
continuum approximation. This is consistent with the fact that there is<br />
no true <strong>Bose</strong>-<strong>Einstein</strong> condensate in one dimension (subsection 2.1.2). The<br />
long-range order is destroyed by the long wave-length fluctuations. However,<br />
in finite systems, where the quasi-condensate concept holds, the sum can<br />
always be evaluated.<br />
55