Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...

T
1
B 2
2.5. Exact diagonalization of the Bogoliubov problem
0 0.5 1 1.5 2
kξ
2.5 3 3.5 4
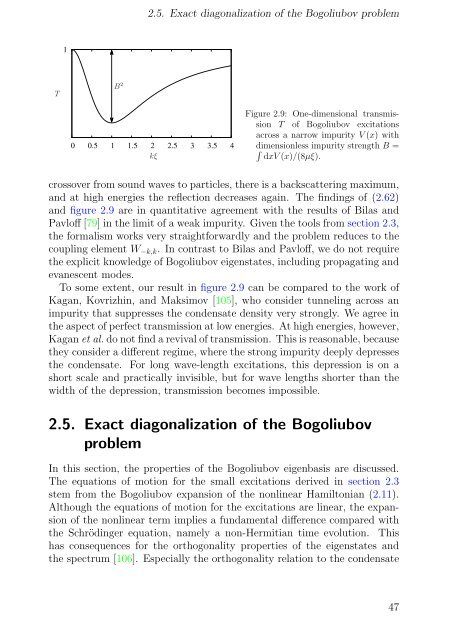
Figure 2.9: One-dimensional transmission
T of Bogoliubov excitations
across a narrow impurity V (x) with
dimensionless impurity strength B =
� dxV (x)/(8µξ).
crossover from sound waves to particles, there is a backscattering maximum,
and at high energies the reflection decreases again. The findings of (2.62)
and figure 2.9 are in quantitative agreement with the results of Bilas and
Pavloff [79] in the limit of a weak impurity. Given the tools from section 2.3,
the formalism works very straightforwardly and the problem reduces to the
coupling element W−k,k. In contrast to Bilas and Pavloff, we do not require
the explicit knowledge of Bogoliubov eigenstates, including propagating and
evanescent modes.
To some extent, our result in figure 2.9 can be compared to the work of
Kagan, Kovrizhin, and Maksimov [105], who consider tunneling across an
impurity that suppresses the condensate density very strongly. We agree in
the aspect of perfect transmission at low energies. At high energies, however,
Kagan et al. do not find a revival of transmission. This is reasonable, because
they consider a different regime, where the strong impurity deeply depresses
the condensate. For long wave-length excitations, this depression is on a
short scale and practically invisible, but for wave lengths shorter than the
width of the depression, transmission becomes impossible.
2.5. Exact diagonalization of the Bogoliubov
problem
In this section, the properties of the Bogoliubov eigenbasis are discussed.
The equations of motion for the small excitations derived in section 2.3
stem from the Bogoliubov expansion of the nonlinear Hamiltonian (2.11).
Although the equations of motion for the excitations are linear, the expansion
of the nonlinear term implies a fundamental difference compared with
the Schrödinger equation, namely a non-Hermitian time evolution. This
has consequences for the orthogonality properties of the eigenstates and
the spectrum [106]. Especially the orthogonality relation to the condensate
47