Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...

6.2. Model
with 1/m(t) = 2 cos p(t). Higher spatial derivatives of A have been neglected.
Note that we choose an immobile wave packet with p(0) = 0 as
initial condition, which fixes the phase for the subsequent Bloch oscillations.
Let us consider solutions of (6.8) in two simple limits (a) and (b).
(a) Linear Bloch oscillation
In absence of the nonlinear term, a Schrödinger equation with a timedependent
mass is to be solved. This can be done easily in Fourier space
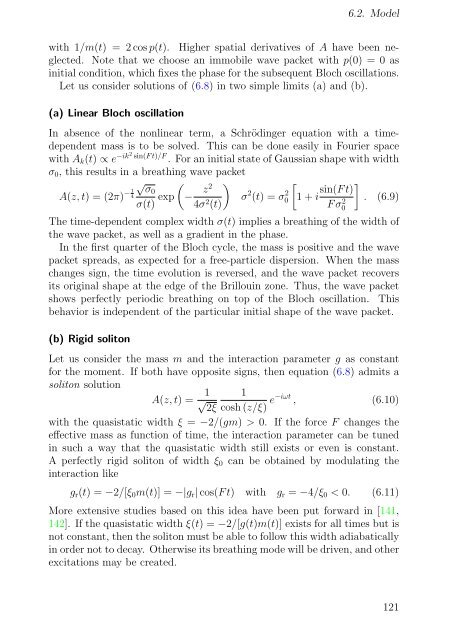
with Ak(t) ∝ e −ik2 sin(F t)/F . For an initial state of Gaussian shape with width
σ0, this results in a breathing wave packet
√
1 −
σ0
A(z, t) = (2π) 4
σ(t) exp
�
− z2
4σ2 �
(t)
σ 2 (t) = σ 2 0
�
sin(F t)
1 + i
F σ2 �
0
. (6.9)
The time-dependent complex width σ(t) implies a breathing of the width of
the wave packet, as well as a gradient in the phase.
In the first quarter of the Bloch cycle, the mass is positive and the wave
packet spreads, as expected for a free-particle dispersion. When the mass
changes sign, the time evolution is reversed, and the wave packet recovers
its original shape at the edge of the Brillouin zone. Thus, the wave packet
shows perfectly periodic breathing on top of the Bloch oscillation. This
behavior is independent of the particular initial shape of the wave packet.
(b) Rigid soliton
Let us consider the mass m and the interaction parameter g as constant
for the moment. If both have opposite signs, then equation (6.8) admits a
soliton solution
A(z, t) = 1
√ 2ξ
1
cosh (z/ξ) e−iωt , (6.10)
with the quasistatic width ξ = −2/(gm) > 0. If the force F changes the
effective mass as function of time, the interaction parameter can be tuned
in such a way that the quasistatic width still exists or even is constant.
A perfectly rigid soliton of width ξ0 can be obtained by modulating the
interaction like
gr(t) = −2/[ξ0m(t)] = −|gr| cos(F t) with gr = −4/ξ0 < 0. (6.11)
More extensive studies based on this idea have been put forward in [141,
142]. If the quasistatic width ξ(t) = −2/[g(t)m(t)] exists for all times but is
not constant, then the soliton must be able to follow this width adiabatically
in order not to decay. Otherwise its breathing mode will be driven, and other
excitations may be created.
121