Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...

6. Bloch Oscillations and Time-Dependent Interactions
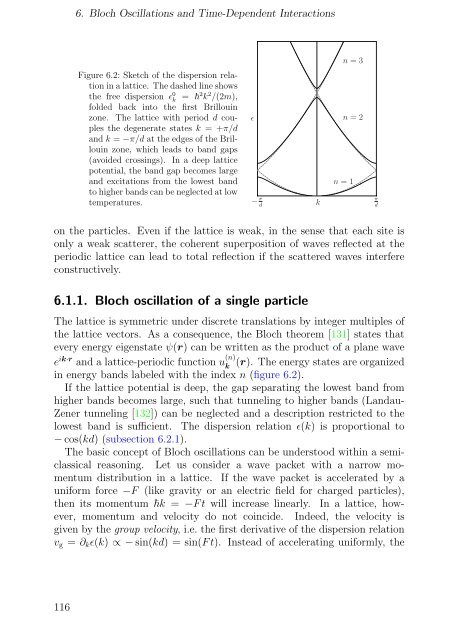
Figure 6.2: Sketch of the dispersion relation
in a lattice. The dashed line shows
the free dispersion ɛ 0 k = �2 k 2 /(2m),
folded back into the first Brillouin
zone. The lattice with period d couples
the degenerate states k = +π/d
and k = −π/d at the edges of the Brillouin
zone, which leads to band gaps
(avoided crossings). In a deep lattice
potential, the band gap becomes large
and excitations from the lowest band
to higher bands can be neglected at low
temperatures. − k
π
ǫ
d
n = 3
n = 2
n = 1
on the particles. Even if the lattice is weak, in the sense that each site is
only a weak scatterer, the coherent superposition of waves reflected at the
periodic lattice can lead to total reflection if the scattered waves interfere
constructively.
6.1.1. Bloch oscillation of a single particle
The lattice is symmetric under discrete translations by integer multiples of
the lattice vectors. As a consequence, the Bloch theorem [131] states that
every energy eigenstate ψ(r) can be written as the product of a plane wave
eik·r and a lattice-periodic function u (n)
k (r). The energy states are organized
in energy bands labeled with the index n (figure 6.2).
If the lattice potential is deep, the gap separating the lowest band from
higher bands becomes large, such that tunneling to higher bands (Landau-
Zener tunneling [132]) can be neglected and a description restricted to the
lowest band is sufficient. The dispersion relation ɛ(k) is proportional to
− cos(kd) (subsection 6.2.1).
The basic concept of Bloch oscillations can be understood within a semiclassical
reasoning. Let us consider a wave packet with a narrow momentum
distribution in a lattice. If the wave packet is accelerated by a
uniform force −F (like gravity or an electric field for charged particles),
then its momentum �k = −F t will increase linearly. In a lattice, however,
momentum and velocity do not coincide. Indeed, the velocity is
given by the group velocity, i.e. the first derivative of the dispersion relation
vg = ∂kɛ(k) ∝ − sin(kd) = sin(F t). Instead of accelerating uniformly, the
116
π
d