Bogoliubov Excitations of Inhomogeneous Bose-Einstein ...

4. Disorder—Results and Limiting Cases
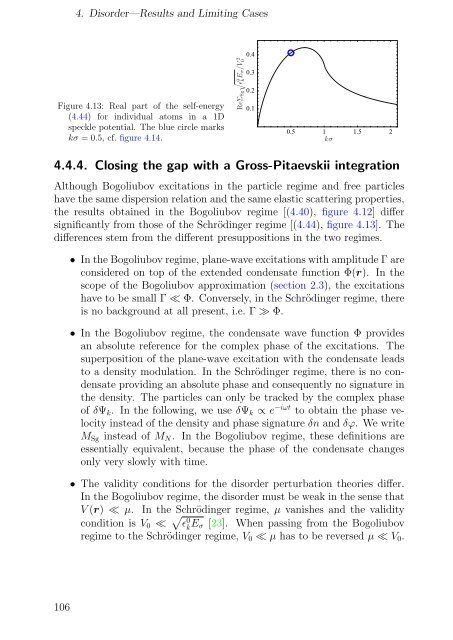
Figure 4.13: Real part of the self-energy
(4.44) for individual atoms in a 1D
speckle potential. The blue circle marks
kσ = 0.5, cf. figure 4.14.
2 Eσ/V0 �
ReΣSg ǫ0 k
0.4
0.3
0.2
0.1
0.5 1 1.5 2
kσ
4.4.4. Closing the gap with a Gross-Pitaevskii integration
Although Bogoliubov excitations in the particle regime and free particles
have the same dispersion relation and the same elastic scattering properties,
the results obtained in the Bogoliubov regime [(4.40), figure 4.12] differ
significantly from those of the Schrödinger regime [(4.44), figure 4.13]. The
differences stem from the different presuppositions in the two regimes.
106
• In the Bogoliubov regime, plane-wave excitations with amplitude Γ are
considered on top of the extended condensate function Φ(r). In the
scope of the Bogoliubov approximation (section 2.3), the excitations
have to be small Γ ≪ Φ. Conversely, in the Schrödinger regime, there
is no background at all present, i.e. Γ ≫ Φ.
• In the Bogoliubov regime, the condensate wave function Φ provides
an absolute reference for the complex phase of the excitations. The
superposition of the plane-wave excitation with the condensate leads
to a density modulation. In the Schrödinger regime, there is no condensate
providing an absolute phase and consequently no signature in
the density. The particles can only be tracked by the complex phase
of δΨk. In the following, we use δΨk ∝ e −iωt to obtain the phase velocity
instead of the density and phase signature δn and δϕ. We write
MSg instead of MN. In the Bogoliubov regime, these definitions are
essentially equivalent, because the phase of the condensate changes
only very slowly with time.
• The validity conditions for the disorder perturbation theories differ.
In the Bogoliubov regime, the disorder must be weak in the sense that
V (r) ≪ µ. In the Schrödinger regime, µ vanishes and the validity
condition is V0 ≪ � ɛ 0 k Eσ [23]. When passing from the Bogoliubov
regime to the Schrödinger regime, V0 ≪ µ has to be reversed µ ≪ V0.