Chapter 1 Topics in Analytic Geometry

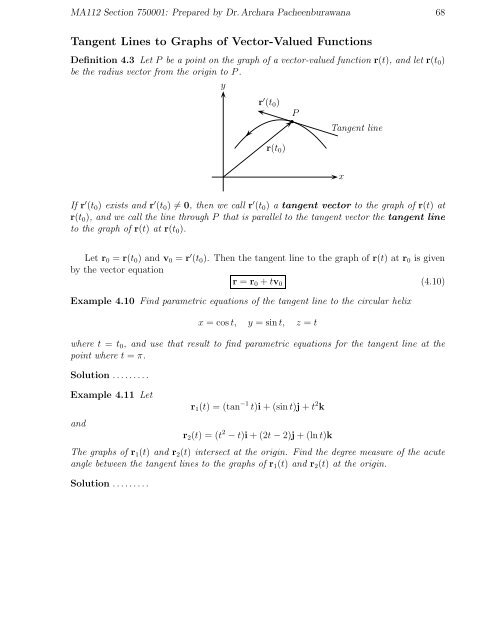
•MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 68Tangent Lines to Graphs of Vector-Valued FunctionsDefinition 4.3 Let P be a point on the graph of a vector-valued function r(t), and let r(t 0 )be the radius vector from the origin to P.yr ′ (t 0 )r(t 0 )PTangent linexIf r ′ (t 0 ) exists and r ′ (t 0 ) ≠ 0, then we call r ′ (t 0 ) a tangent vector to the graph of r(t) atr(t 0 ), and we call the line through P that is parallel to the tangent vector the tangent lineto the graph of r(t) at r(t 0 ).Let r 0 = r(t 0 ) and v 0 = r ′ (t 0 ). Then the tangent line to the graph of r(t) at r 0 is givenby the vector equationr = r 0 +tv 0 (4.10)Example 4.10 Find parametric equations of the tangent line to the circular helixx = cost, y = sint, z = twhere t = t 0 , and use that result to find parametric equations for the tangent line at thepoint where t = π.Solution .........Example 4.11 Letandr 1 (t) = (tan −1 t)i+(sint)j+t 2 kr 2 (t) = (t 2 −t)i+(2t−2)j+(lnt)kThe graphs of r 1 (t) and r 2 (t) intersect at the origin. Find the degree measure of the acuteangle between the tangent lines to the graphs of r 1 (t) and r 2 (t) at the origin.Solution .........