Chapter 1 Topics in Analytic Geometry
Chapter 1 Topics in Analytic Geometry Chapter 1 Topics in Analytic Geometry
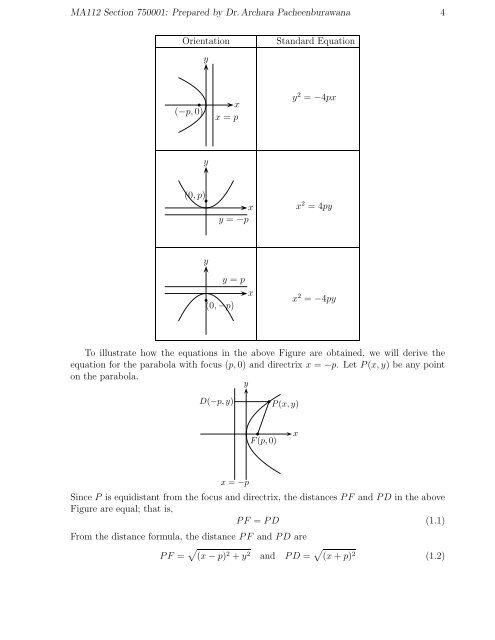
•MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 4OrientationyStandard Equation•(−p,0)xx = py 2 = −4pxy(0,p) •xy = −px 2 = 4pyyy = px•(0,−p)x 2 = −4pyTo illustrate how the equations in the above Figure are obtained, we will derive theequation for the parabola with focus (p,0) and directrix x = −p. Let P(x,y) be any pointon the parabola.yD(−p,y)P(x,y)•F(p,0)xx = −pSince P is equidistant from the focus and directrix, the distances PF and PD in the aboveFigure are equal; that is,PF = PD (1.1)From the distance formula, the distance PF and PD arePF = √ (x−p) 2 +y 2 and PD = √ (x+p) 2 (1.2)
•••••MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 5Substituting in (3.22) and squaring yieldsand after simplifying(x−p) 2 +y 2 = (x+p) 2 (1.3)y 2 = 4px (1.4)The derivations of the other equations in the previous Figure are similar.Example 1.1 Find the focus and directrix of the parabola x 2 = −6y and sketch its graph.Solution .........Example 1.2(a) Find an equation of a parabola that has vertex at the origin, opens right, and passesthrough the point P(7,−3).(b) Find the focus.Solution .........Equations of Ellipses in Standard PositionIt is traditional in the study of ellipse to denote the length of the major axis by 2a, thelength of the minor axis by 2b, and the distance between the foci by 2c.ccbbaaThe number a is called the semimajor axis and the number b the semiminor axis.There is a basic relationship between a, b, and c that can be obtained by examining thesum of the distances to the foci from a point P at the end of the major axis and from apoint Q at the end of the minor axis.√b2 +c 2Qbc c a−c√b2 +c 2•P
- Page 1 and 2: ••Chapter 1Topics in Analytic G
- Page 3: MA112 Section 750001: Prepared by D
- Page 7 and 8: MA112 Section 750001: Prepared by D
- Page 9 and 10: MA112 Section 750001: Prepared by D
- Page 11 and 12: •MA112 Section 750001: Prepared b
- Page 13 and 14: MA112 Section 750001: Prepared by D
- Page 15 and 16: •••••••••••MA
- Page 17 and 18: MA112 Section 750001: Prepared by D
- Page 19 and 20: MA112 Section 750001: Prepared by D
- Page 21 and 22: MA112 Section 750001: Prepared by D
- Page 23 and 24: MA112 Section 750001: Prepared by D
- Page 25 and 26: MA112 Section 750001: Prepared by D
- Page 27 and 28: MA112 Section 750001: Prepared by D
- Page 29 and 30: MA112 Section 750001: Prepared by D
- Page 31 and 32: MA112 Section 750001: Prepared by D
- Page 33 and 34: MA112 Section 750001: Prepared by D
- Page 35 and 36: MA112 Section 750001: Prepared by D
- Page 37: MA112 Section 750001: Prepared by D
- Page 41 and 42: •MA112 Section 750001: Prepared b
- Page 43 and 44: MA112 Section 750001: Prepared by D
- Page 45 and 46: MA112 Section 750001: Prepared by D
- Page 47 and 48: MA112 Section 750001: Prepared by D
- Page 49 and 50: ••••••MA112 Section 750
- Page 51 and 52: ••MA112 Section 750001: Prepare
- Page 53 and 54: MA112 Section 750001: Prepared by D
•MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 4OrientationyStandard Equation•(−p,0)xx = py 2 = −4pxy(0,p) •xy = −px 2 = 4pyyy = px•(0,−p)x 2 = −4pyTo illustrate how the equations <strong>in</strong> the above Figure are obta<strong>in</strong>ed, we will derive theequation for the parabola with focus (p,0) and directrix x = −p. Let P(x,y) be any po<strong>in</strong>ton the parabola.yD(−p,y)P(x,y)•F(p,0)xx = −pS<strong>in</strong>ce P is equidistant from the focus and directrix, the distances PF and PD <strong>in</strong> the aboveFigure are equal; that is,PF = PD (1.1)From the distance formula, the distance PF and PD arePF = √ (x−p) 2 +y 2 and PD = √ (x+p) 2 (1.2)