The Matrix Padé Approximation in Systems of Differential ... - WSEAS

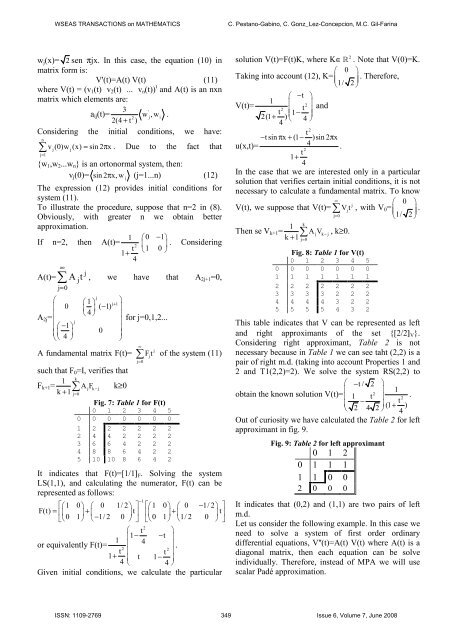
WSEAS TRANSACTIONS on MATHEMATICSC. Pestano-Gabino, C. Gonz_Lez-Concepcion, M.C. Gil-Farinaw j (x)= 2 sen πjx. In this case, the equation (10) inmatrix form is:V'(t)=A(t) V(t) (11)where V(t) = (v 1 (t) v 2 (t) ... v n (t)) t and A(t) is an nxnmatrix which elements are:3 'a ij (t)= w2 j, wi.2(4 + t )Considering the initial conditions, we have:n∑ vj(0)w j(x)= sin 2πx. Due to the fact thatj=1{w 1 ,w 2 ...w n } is an ortonormal system, then:v j (0)= sin 2π x, wj(j=1...n) (12)The expression (12) provides initial conditions forsystem (11).To illustrate the procedure, suppose that n=2 in (8).Obviously, with greater n we obtain betterapproximation.1 ⎛0 −1⎞If n=2, then A(t)=2 ⎜ ⎟t 1 0. Considering1+⎝ ⎠4A(t)=∞∑j= 0jA jtj⎛ ⎛ 1 ⎞⎜ 0 ⎜ ⎟ ( −1)4A 2j =⎜ ⎝ ⎠⎜ j⎛ −1⎞⎜⎜⎟ 0⎝⎝4 ⎠, we have that A 2j+1 =0,j+1A fundamental matrix F(t)=such that F 0 =I, verifies thatk1F k+1 = ∑ AjFk − jk≥0k + 1j=0⎞⎟⎟⎟for j=0,1,2...⎟⎠Fig. 7: Table 1 for F(t)0 1 2 3 4 50 0 0 0 0 0 01 2 2 2 2 2 22 4 4 2 2 2 23 6 6 4 2 2 24 8 8 6 4 2 25 10 10 8 6 4 2∞j∑ Fjtof the system (11)j=0It indicates that F(t)=[1/1] F . Solving the systemLS(1,1), and calculating the numerator, F(t) can berepresented as follows:⎡⎛1 0⎞ ⎛ 0 1/ 2⎞ ⎤ ⎡⎛1 0⎞ ⎛ 0 −1/ 2⎞⎤F(t) = ⎢⎜ ⎟ + ⎜ ⎟ t⎥ ⎢⎜ ⎟ + ⎜ ⎟ t⎥⎣⎝0 1⎠ ⎝ −1/ 2 0 ⎠ ⎦ ⎣⎝0 1⎠ ⎝1/ 2 0 ⎠ ⎦2⎛ t ⎞1 t1⎜ − − ⎟or equivalently F(t)= ⎜ 4 ⎟ .2 2t ⎜ t ⎟1+ t 1−4⎜⎟⎝ 4 ⎠Given initial conditions, we calculate the particular−1solution V(t)=F(t)K, where K∈ R 2 . Note that V(0)=K.⎛ 0 ⎞Taking into account (12), K=⎜1/ 2 ⎟. Therefore,⎝ ⎠⎛ −t⎞1V(t)= ⎜ 2 ⎟2 t andt 1−2(1 + ) ⎜ ⎟⎝ 4 ⎠42t−t sin π x + (1 − )sin 2πxu(x,t)=4 .2t1+4In the case that we are interested only in a particularsolution that verifies certain initial conditions, it is notnecessary to calculate a fundamental matrix. To know∞⎛ 0 ⎞jV(t), we suppose that V(t)= ∑ Vjt, with V 0 =⎜j=01/ 2 ⎟.⎝ ⎠k1Then se V k+1 = ∑ AjVk − j, k≥0.k + 1j=0Fig. 8: Table 1 for V(t)0 1 2 3 4 50 0 0 0 0 0 01 1 1 1 1 1 12 2 2 2 2 2 23 3 3 3 2 2 24 4 4 4 3 2 25 5 5 5 4 3 2This table indicates that V can be represented as leftand right approximants of the set {[2/2] V }.Considering right approximant, Table 2 is notnecessary because in Table 1 we can see taht (2,2) is apair of right m.d. (taking into account Properties 1 and2 and T1(2,2)=2). We solve the system RS(2,2) to⎛ −t / 2 ⎞⎜ ⎟ 1obtain the known solution V(t)= 2⎜ 1 t ⎟ .2⎜ − ⎟t⎝ 2 4 2 ⎠(1 + )4Out of curiosity we have calculated the Table 2 for leftapproximant in fig. 9.Fig. 9: Table 2 for left approximant0 1 20 1 1 11 1 0 02 0 0 0It indicates that (0,2) and (1,1) are two pairs of leftm.d.Let us consider the following example. In this case weneed to solve a system of first order ordinarydifferential equations, V''(t)=A(t) V(t) where A(t) is adiagonal matrix, then each equation can be solveindividually. Therefore, instead of MPA we will usescalar Padé approximation.ISSN: 1109-2769 349 Issue 6, Volume 7, June 2008