1mZ2hsN

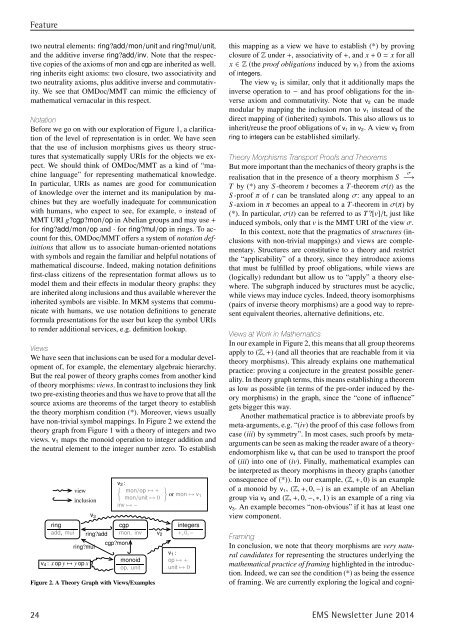
Featuretwo neutral elements: ring?add/mon/unit and ring?mul/unit,and the additive inverse ring?add/inv. Note that the respectivecopies of the axioms of mon and cgp are inherited as well.ring inherits eight axioms: two closure, two associativity andtwo neutrality axioms, plus additive inverse and commutativity.We see that OMDoc/MMT can mimic the efficiency ofmathematical vernacular in this respect.NotationBefore we go on with our exploration of Figure 1, a clarificationof the level of representation is in order. We have seenthat the use of inclusion morphisms gives us theory structuresthat systematically supply URIs for the objects we expect.We should think of OMDoc/MMT as a kind of “machinelanguage” for representing mathematical knowledge.In particular, URIs as names are good for communicationof knowledge over the internet and its manipulation by machinesbut they are woefully inadequate for communicationwith humans, who expect to see, for example, ◦ instead ofMMT URI g?cgp?mon/op in Abelian groups and may use +for ring?add/mon/op and · for ring?mul/op in rings. To accountfor this, OMDoc/MMT offers a system of notation definitionsthat allow us to associate human-oriented notationswith symbols and regain the familiar and helpful notations ofmathematical discourse. Indeed, making notation definitionsfirst-class citizens of the representation format allows us tomodel them and their effects in modular theory graphs: theyare inherited along inclusions and thus available wherever theinherited symbols are visible. In MKM systems that communicatewith humans, we use notation definitions to generateformula presentations for the user but keep the symbol URIsto render additional services, e.g. definition lookup.ViewsWe have seen that inclusions can be used for a modular developmentof, for example, the elementary algebraic hierarchy.But the real power of theory graphs comes from another kindof theory morphisms: views. In contrast to inclusions they linktwo pre-existing theories and thus we have to prove that all thesource axioms are theorems of the target theory to establishthe theory morphism condition (*). Moreover, views usuallyhave non-trivial symbol mappings. In Figure 2 we extend thetheory graph from Figure 1 with a theory of integers and twoviews. v 1 maps the monoid operation to integer addition andthe neutral element to the integer number zero. To establishringadd, mulviewinclusionring?mulv 4 : x op y → y op xv 3ring?addv 2 :mon/op → +mon/unit → 0inv → −cgp?moncgpmon, invmonoidop, unitFigure 2. A Theory Graph with Views/Examplesv 2or mon → v 1integers+, 0, −v 1 :op → +unit → 0this mapping as a view we have to establish (*) by provingclosure of Z under +, associativity of +, and x + 0 = x for allx ∈ Z (the proof obligations induced by v 1 ) from the axiomsof integers.The view v 2 is similar, only that it additionally maps theinverse operation to − and has proof obligations for the inverseaxiom and commutativity. Note that v 2 can be mademodular by mapping the inclusion mon to v 1 instead of thedirect mapping of (inherited) symbols. This also allows us toinherit/reuse the proof obligations of v 1 in v 2 . A view v 3 fromring to integers can be established similarly.Theory Morphisms Transport Proofs and TheoremsBut more important than the mechanics of theory graphs is theσrealisation that in the presence of a theory morphism S −→T by (*) any S -theorem t becomes a T-theorem σ(t) as theS -proof π of t can be translated along σ: any appeal to anS -axiom in π becomes an appeal to a T-theorem in σ(π) by(*). In particular, σ(t) can be referred to as T?[v]/t, just likeinduced symbols, only that v is the MMT URI of the view σ.In this context, note that the pragmatics of structures (inclusionswith non-trivial mappings) and views are complementary.Structures are constitutive to a theory and restrictthe “applicability” of a theory, since they introduce axiomsthat must be fulfilled by proof obligations, while views are(logically) redundant but allow us to “apply” a theory elsewhere.The subgraph induced by structures must be acyclic,while views may induce cycles. Indeed, theory isomorphisms(pairs of inverse theory morphisms) are a good way to representequivalent theories, alternative definitions, etc.Views at Work in MathematicsIn our example in Figure 2, this means that all group theoremsapply to (Z, +) (and all theories that are reachable from it viatheory morphisms). This already explains one mathematicalpractice: proving a conjecture in the greatest possible generality.In theory graph terms, this means establishing a theoremas low as possible (in terms of the pre-order induced by theorymorphisms) in the graph, since the “cone of influence”gets bigger this way.Another mathematical practice is to abbreviate proofs bymeta-arguments, e.g. “(iv) the proof of this case follows fromcase (iii) by symmetry”. In most cases, such proofs by metaargumentscan be seen as making the reader aware of a theoryendomorphismlike v 4 that can be used to transport the proofof (iii) into one of (iv). Finally, mathematical examples canbe interpreted as theory morphisms in theory graphs (anotherconsequence of (*)). In our example, (Z, +, 0) is an exampleof a monoid by v 1 ,(Z, +, 0, −) is an example of an Abeliangroup via v 2 and (Z, +, 0, −, ∗, 1) is an example of a ring viav 3 . An example becomes “non-obvious” if it has at least oneview component.FramingIn conclusion, we note that theory morphisms are very naturalcandidates for representing the structures underlying themathematical practice of framing highlighted in the introduction.Indeed, we can see the condition (*) as being the essenceof framing. We are currently exploring the logical and cogni-24 EMS Newsletter June 2014