Plane Geometry - Bruce E. Shapiro
Plane Geometry - Bruce E. Shapiro Plane Geometry - Bruce E. Shapiro
90 SECTION 17. THE CROSSBAR THEOREMTheorem 17.2 (MacLane’s Continuity Axiom a ) A point D is in theinterior of angle ∠BAC if and only if the ray −→ AD intersects the interior ofthe segment BC.a MacLane [MacLane, 1959] takes this result as an axiom and uses this name because itimplies Birkhoff’s [Birkhoff, 1932] continuity axiom (theorem 20.2).Figure 17.6: Illustration of MacLane’s Continuity Axiom (theorem 17.2.)Proof. (⇒) Suppose that D is in the interior of ∠BAC.−→crossbar theorem, AD intersects BC.Then by theSince the intersection point lies on a ray that is interior to ∠BAC, it doesnot lie on an endpoint of BC (definition of interior; else it would lie on one ofthe two rays that define the angle, not the intersection of their half-planes);hence the intersection point is interior to BC.(⇐) Suppose that −→ AD intersects the interior of BC. Call the point ofintersection E.−→ −→ −→ −→ −→Hence B ∗ E ∗ C. By theorem 16.8, AB ∗ AE ∗ AC. Hence AE = AD is inthe interior of angle ∠BAC. Hence D is in the interior of ∠BAC.« CC BY-NC-ND 3.0. Revised: 18 Nov 2012
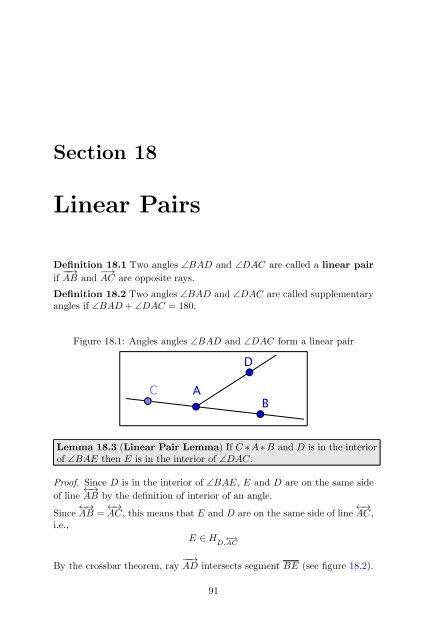
Section 18Linear PairsDefinition 18.1 Two angles ∠BAD and ∠DAC are called a linear pair−→ −→if AB and AC are opposite rays.Definition 18.2 Two angles ∠BAD and ∠DAC are called supplementaryangles if ∠BAD + ∠DAC = 180.Figure 18.1: Angles angles ∠BAD and ∠DAC form a linear pairLemma 18.3 (Linear Pair Lemma) If C ∗ A ∗ B and D is in the interiorof ∠BAE then E is in the interior of ∠DAC.Proof. Since D is in the interior of ∠BAE, E and D are on the same sideof line ←→ AB by the definition of interior of an angle.Since ←→ AB = ←→ AC, this means that E and D are on the same side of line ←→ AC,i.e.,E ∈ H ←→ D, ACBy the crossbar theorem, ray −→ AD intersects segment BE (see figure 18.2).91
- Page 44 and 45: 40 SECTION 9. BIRKHOFF/MACLANE AXIO
- Page 46 and 47: 42 SECTION 10. THE SMSG AXIOMSP is
- Page 48 and 49: 44 SECTION 10. THE SMSG AXIOMS« CC
- Page 50 and 51: 46 SECTION 11. THE UCSMP AXIOMSin s
- Page 52 and 53: 48 SECTION 11. THE UCSMP AXIOMS« C
- Page 54 and 55: 50 SECTION 12. VENEMA’S AXIOMS3.
- Page 56 and 57: 52 SECTION 12. VENEMA’S AXIOMS«
- Page 58 and 59: 54 SECTION 13. INCIDENCE GEOMETRYFi
- Page 60 and 61: 56 SECTION 13. INCIDENCE GEOMETRYEx
- Page 62 and 63: 58 SECTION 13. INCIDENCE GEOMETRYFi
- Page 64 and 65: 60 SECTION 13. INCIDENCE GEOMETRY«
- Page 66 and 67: 62 SECTION 14. BETWEENNESSFigure 14
- Page 68 and 69: 64 SECTION 14. BETWEENNESSTheorem 1
- Page 70 and 71: 66 SECTION 14. BETWEENNESS(a) To sh
- Page 72 and 73: 68 SECTION 14. BETWEENNESSThe follo
- Page 74 and 75: 70 SECTION 14. BETWEENNESSExample 1
- Page 76 and 77: 72 SECTION 14. BETWEENNESSorf(A) >
- Page 78 and 79: 74 SECTION 14. BETWEENNESS« CC BY-
- Page 80 and 81: 76 SECTION 15. THE PLANE SEPARATION
- Page 82 and 83: 78 SECTION 15. THE PLANE SEPARATION
- Page 84 and 85: 80 SECTION 16. ANGLESFigure 16.1: T
- Page 86 and 87: 82 SECTION 16. ANGLESCorollary 16.6
- Page 88 and 89: 84 SECTION 16. ANGLESFigure 16.6: I
- Page 90 and 91: 86 SECTION 16. ANGLESD cannot be in
- Page 92 and 93: 88 SECTION 17. THE CROSSBAR THEOREM
- Page 96 and 97: 92 SECTION 18. LINEAR PAIRSFigure 1
- Page 98 and 99: 94 SECTION 18. LINEAR PAIRSγ + ∠
- Page 100 and 101: 96 SECTION 19. ANGLE BISECTORSwith
- Page 102 and 103: 98 SECTION 19. ANGLE BISECTORSAngle
- Page 104 and 105: 100 SECTION 20. THE CONTINUITY AXIO
- Page 106 and 107: 102 SECTION 20. THE CONTINUITY AXIO
- Page 108 and 109: 104 SECTION 21. SIDE-ANGLE-SIDEBirk
- Page 110 and 111: 106 SECTION 21. SIDE-ANGLE-SIDEFigu
- Page 112 and 113: 108 SECTION 22. NEUTRAL GEOMETRYEll
- Page 114 and 115: 110 SECTION 22. NEUTRAL GEOMETRY«
- Page 116 and 117: 112 SECTION 23. ANGLE-SIDE-ANGLEFig
- Page 118 and 119: 114 SECTION 23. ANGLE-SIDE-ANGLE«
- Page 120 and 121: 116 SECTION 24. EXTERIOR ANGLESTheo
- Page 122 and 123: 118 SECTION 24. EXTERIOR ANGLES« C
- Page 124 and 125: 120 SECTION 25. ANGLE-ANGLE-SIDEBy
- Page 126 and 127: 122 SECTION 26. SIDE-SIDE-SIDECase
- Page 128 and 129: 124 SECTION 26. SIDE-SIDE-SIDEBy si
- Page 130 and 131: 126 SECTION 27. SCALENE AND TRIANGL
- Page 132 and 133: 128 SECTION 27. SCALENE AND TRIANGL
- Page 134 and 135: 130 SECTION 28. CHARACTERIZATION OF
- Page 136 and 137: 132 SECTION 28. CHARACTERIZATION OF
- Page 138 and 139: 134 SECTION 29. TRANSVERSALSFigure
- Page 140 and 141: 136 SECTION 29. TRANSVERSALSCorolla
- Page 142 and 143: 138 SECTION 30. TRIANGLES IN NEUTRA
Section 18Linear PairsDefinition 18.1 Two angles ∠BAD and ∠DAC are called a linear pair−→ −→if AB and AC are opposite rays.Definition 18.2 Two angles ∠BAD and ∠DAC are called supplementaryangles if ∠BAD + ∠DAC = 180.Figure 18.1: Angles angles ∠BAD and ∠DAC form a linear pairLemma 18.3 (Linear Pair Lemma) If C ∗ A ∗ B and D is in the interiorof ∠BAE then E is in the interior of ∠DAC.Proof. Since D is in the interior of ∠BAE, E and D are on the same sideof line ←→ AB by the definition of interior of an angle.Since ←→ AB = ←→ AC, this means that E and D are on the same side of line ←→ AC,i.e.,E ∈ H ←→ D, ACBy the crossbar theorem, ray −→ AD intersects segment BE (see figure 18.2).91