Plane Geometry - Bruce E. Shapiro
Plane Geometry - Bruce E. Shapiro Plane Geometry - Bruce E. Shapiro
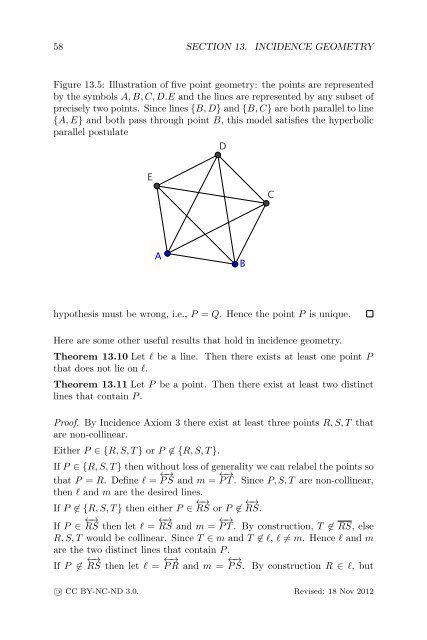
58 SECTION 13. INCIDENCE GEOMETRYFigure 13.5: Illustration of five point geometry: the points are representedby the symbols A, B, C, D.E and the lines are represented by any subset ofprecisely two points. Since lines {B, D} and {B, C} are both parallel to line{A, E} and both pass through point B, this model satisfies the hyperbolicparallel postulatehypothesis must be wrong, i.e., P = Q. Hence the point P is unique.Here are some other useful results that hold in incidence geometry.Theorem 13.10 Let l be a line. Then there exists at least one point Pthat does not lie on l.Theorem 13.11 Let P be a point. Then there exist at least two distinctlines that contain P .Proof. By Incidence Axiom 3 there exist at least three points R, S, T thatare non-collinear.Either P ∈ {R, S, T } or P ∉ {R, S, T }.If P ∈ {R, S, T } then without loss of generality we can relabel the points sothat P = R. Define l = ←→ P S and m = ←→ P T . Since P, S, T are non-collinear,then l and m are the desired lines.If P ∉ {R, S, T } then either P ∈ ←→ RS or P ∉ ←→ RS.If P ∈ ←→ RS then let l = ←→ RS and m = ←→ P T . By construction, T ∉ RS, elseR, S, T would be collinear. Since T ∈ m and T ∉ l, l ≠ m. Hence l and mare the two distinct lines that contain P .If P ∉ ←→ RS then let l = ←→ P R and m = ←→ P S. By construction R ∈ l, but« CC BY-NC-ND 3.0. Revised: 18 Nov 2012
SECTION 13. INCIDENCE GEOMETRY 59R ∉ m, else P, R, S would be collinear, and we have assumed otherwise.Hence l and m are distinct lines that contain P .Theorem 13.12 Let l be a line. Then there exist two distinct lines m andn that intersect l.Proof. Let l be a line.By incidence axiom 2 there are two points P, Q thatlie on l.By theorem 13.10 there exists a third point R that does lie on l.By incidence axiom 1, there exist lines m = ←→ P R and n = ←→ QR.Since P ∈ l and P ∈ m, l intersects m (definition of intersection).Since Q ∈ l and Q ∈ n, l intersects n (definition of intersection).Since R ∉ l then any line that contains R is different from l. The two linesm and n contain R, hence m ≠ l and n ≠ l.Suppose m = n. By definition of m, P ∈ m. By defintion of n, Q ∈ n.Hence if m = n, Q ∈ m, i.e., both P and Q line on m. Hence m = l bythe uniqueness part of incidence axiom 1. This contradicts the previousparagraph, m ≠ l. Hence the assumption m = n must be wrong.Hence m ≠ n, which means there are two distinc lines that intersect l.Theorem 13.13 Let P be a point. Then there exists at least one line thatdoes not contain P .Theorem 13.14 There exist three distinct lines such that no point lies onall three of them.Theorem 13.15 Let P be a point. Then there exist points Q and R suchthat P, Q, R are non-collinear.Proof. Let P and Q be points such that Q ≠ P . By incidence axiom 1,there is a unique line l that contains P and Q.By theorem 13.10 there exists at least one point R that does lie on l. Thepoints P, Q, R are non-collinear.Theorem 13.16 Let P ≠ Q be points. Then there exists a point Q suchthat P, Q, R are non-collinear.Revised: 18 Nov 2012 « CC BY-NC-ND 3.0.
- Page 12 and 13: 8 SECTION 2. NCTMTechnology. Techno
- Page 14 and 15: 10 SECTION 2. NCTM« CC BY-NC-ND 3.
- Page 16 and 17: 12 SECTION 3. CA STANDARDIn 2005 Ca
- Page 18 and 19: 14 SECTION 3. CA STANDARDThe Califo
- Page 20 and 21: 16 SECTION 3. CA STANDARDCalifornia
- Page 22 and 23: 18 SECTION 4. COMMON COREAt all lev
- Page 24 and 25: 20 SECTION 5. LOGIC AND PROOFour th
- Page 26 and 27: 22 SECTION 5. LOGIC AND PROOFRene D
- Page 28 and 29: 24 SECTION 5. LOGIC AND PROOF“I
- Page 30 and 31: 26 SECTION 6. REAL NUMBERSThe inter
- Page 32 and 33: 28 SECTION 6. REAL NUMBERS“The Su
- Page 34 and 35: 30 SECTION 7. EUCLID’S ELEMENTSEu
- Page 36 and 37: 32 SECTION 7. EUCLID’S ELEMENTSFi
- Page 38 and 39: 34 SECTION 8. HILBERT’S AXIOMSiom
- Page 40 and 41: 36 SECTION 8. HILBERT’S AXIOMS«
- Page 42 and 43: 38 SECTION 9. BIRKHOFF/MACLANE AXIO
- Page 44 and 45: 40 SECTION 9. BIRKHOFF/MACLANE AXIO
- Page 46 and 47: 42 SECTION 10. THE SMSG AXIOMSP is
- Page 48 and 49: 44 SECTION 10. THE SMSG AXIOMS« CC
- Page 50 and 51: 46 SECTION 11. THE UCSMP AXIOMSin s
- Page 52 and 53: 48 SECTION 11. THE UCSMP AXIOMS« C
- Page 54 and 55: 50 SECTION 12. VENEMA’S AXIOMS3.
- Page 56 and 57: 52 SECTION 12. VENEMA’S AXIOMS«
- Page 58 and 59: 54 SECTION 13. INCIDENCE GEOMETRYFi
- Page 60 and 61: 56 SECTION 13. INCIDENCE GEOMETRYEx
- Page 64 and 65: 60 SECTION 13. INCIDENCE GEOMETRY«
- Page 66 and 67: 62 SECTION 14. BETWEENNESSFigure 14
- Page 68 and 69: 64 SECTION 14. BETWEENNESSTheorem 1
- Page 70 and 71: 66 SECTION 14. BETWEENNESS(a) To sh
- Page 72 and 73: 68 SECTION 14. BETWEENNESSThe follo
- Page 74 and 75: 70 SECTION 14. BETWEENNESSExample 1
- Page 76 and 77: 72 SECTION 14. BETWEENNESSorf(A) >
- Page 78 and 79: 74 SECTION 14. BETWEENNESS« CC BY-
- Page 80 and 81: 76 SECTION 15. THE PLANE SEPARATION
- Page 82 and 83: 78 SECTION 15. THE PLANE SEPARATION
- Page 84 and 85: 80 SECTION 16. ANGLESFigure 16.1: T
- Page 86 and 87: 82 SECTION 16. ANGLESCorollary 16.6
- Page 88 and 89: 84 SECTION 16. ANGLESFigure 16.6: I
- Page 90 and 91: 86 SECTION 16. ANGLESD cannot be in
- Page 92 and 93: 88 SECTION 17. THE CROSSBAR THEOREM
- Page 94 and 95: 90 SECTION 17. THE CROSSBAR THEOREM
- Page 96 and 97: 92 SECTION 18. LINEAR PAIRSFigure 1
- Page 98 and 99: 94 SECTION 18. LINEAR PAIRSγ + ∠
- Page 100 and 101: 96 SECTION 19. ANGLE BISECTORSwith
- Page 102 and 103: 98 SECTION 19. ANGLE BISECTORSAngle
- Page 104 and 105: 100 SECTION 20. THE CONTINUITY AXIO
- Page 106 and 107: 102 SECTION 20. THE CONTINUITY AXIO
- Page 108 and 109: 104 SECTION 21. SIDE-ANGLE-SIDEBirk
- Page 110 and 111: 106 SECTION 21. SIDE-ANGLE-SIDEFigu
58 SECTION 13. INCIDENCE GEOMETRYFigure 13.5: Illustration of five point geometry: the points are representedby the symbols A, B, C, D.E and the lines are represented by any subset ofprecisely two points. Since lines {B, D} and {B, C} are both parallel to line{A, E} and both pass through point B, this model satisfies the hyperbolicparallel postulatehypothesis must be wrong, i.e., P = Q. Hence the point P is unique.Here are some other useful results that hold in incidence geometry.Theorem 13.10 Let l be a line. Then there exists at least one point Pthat does not lie on l.Theorem 13.11 Let P be a point. Then there exist at least two distinctlines that contain P .Proof. By Incidence Axiom 3 there exist at least three points R, S, T thatare non-collinear.Either P ∈ {R, S, T } or P ∉ {R, S, T }.If P ∈ {R, S, T } then without loss of generality we can relabel the points sothat P = R. Define l = ←→ P S and m = ←→ P T . Since P, S, T are non-collinear,then l and m are the desired lines.If P ∉ {R, S, T } then either P ∈ ←→ RS or P ∉ ←→ RS.If P ∈ ←→ RS then let l = ←→ RS and m = ←→ P T . By construction, T ∉ RS, elseR, S, T would be collinear. Since T ∈ m and T ∉ l, l ≠ m. Hence l and mare the two distinct lines that contain P .If P ∉ ←→ RS then let l = ←→ P R and m = ←→ P S. By construction R ∈ l, but« CC BY-NC-ND 3.0. Revised: 18 Nov 2012