Plane Geometry - Bruce E. Shapiro
Plane Geometry - Bruce E. Shapiro Plane Geometry - Bruce E. Shapiro
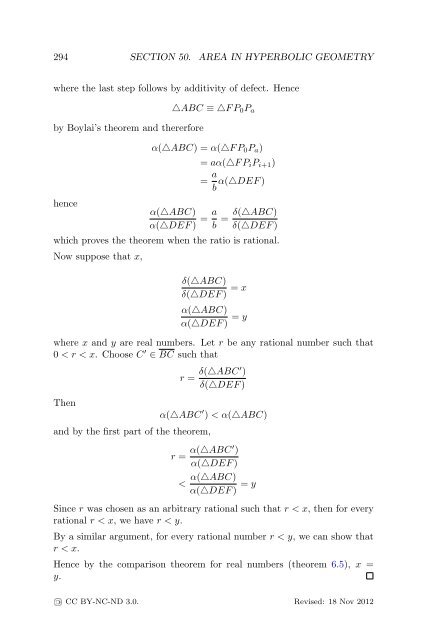
294 SECTION 50. AREA IN HYPERBOLIC GEOMETRYwhere the last step follows by additivity of defect. Henceby Boylai’s theorem and thererforehence△ABC ≡ △F P 0 P aα(△ABC) = α(△F P 0 P a )= aα(△F P i P i+1 )= a b α(△DEF )α(△ABC)α(△DEF ) = a b = δ(△ABC)δ(△DEF )which proves the theorem when the ratio is rational.Now suppose that x,δ(△ABC)δ(△DEF ) = xα(△ABC)α(△DEF ) = ywhere x and y are real numbers. Let r be any rational number such that0 < r < x. Choose C ′ ∈ BC such thatThenand by the first part of the theorem,r = δ(△ABC′ )δ(△DEF )α(△ABC ′ ) < α(△ABC)r = α(△ABC′ )α(△DEF )< α(△ABC)α(△DEF ) = ySince r was chosen as an arbitrary rational such that r < x, then for everyrational r < x, we have r < y.By a similar argument, for every rational number r < y, we can show thatr < x.Hence by the comparison theorem for real numbers (theorem 6.5), x =y.« CC BY-NC-ND 3.0. Revised: 18 Nov 2012
SECTION 50. AREA IN HYPERBOLIC GEOMETRY 295Theorem 50.11 Area is proportional to defect: there exists a constant ksuch thatα(△ABC) = kδ(△ABC)for every triangle △ABC (same k for every triangle).Corollary 50.12 There is an upper bound to the area of triangles.Proof. Since δ(△ABC) < 180 for every triangle, thenα(△ABC) < 180kCorollary 50.13 If α(△ABC) = α(△DEF ) then △ABC ≡ △DEF .Proof.δ(△ABC) = 1 k α(△ABC)By Boylai’s theorem △ABC ≡ △DEF .= 1 k α(△DEF )= δ(△DEF )Theorem 50.14 Let R 1 and R 2 be polygonal regions such that α(R 1 ) =α(R 2 ). Then R 1 ≡ R 2 .Theorem 50.15 There exist triangles with the same base and height butdifferent areas.Proof. Let l be a line and P a point with d(P, l) = 1.Let Q 0 be the foot of the perpendicular from P to l.Define Q 1 , Q 2 , . . . in l such that Q i Q i+1 = 1.Define T i = △P Q i−1 Q i .equal to 1.Then the base and height of each T i are eachSuppose that all the T i have the same area, α(T i ) = a for all i.Then all the T i have the same defect, δ(T i ) = d for all i. By additivity ofdefectδ(△P Q 0 Q n ) = nd < 180Since we can choose n arbitrarily large, this violates the Archimedian orderingproperty. Hence the defects cannot all be equal, and hece the areascannot all be equal.Revised: 18 Nov 2012 « CC BY-NC-ND 3.0.
- Page 248 and 249: 244 SECTION 44. ESTIMATING πn π n
- Page 250 and 251: 246 SECTION 44. ESTIMATING πTable
- Page 252 and 253: 248 SECTION 44. ESTIMATING πnums =
- Page 254 and 255: 250 SECTION 44. ESTIMATING π« CC
- Page 256 and 257: 252 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 258 and 259: 254 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 260 and 261: 256 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 262 and 263: 258 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 264 and 265: 260 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 266 and 267: 262 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 268 and 269: 264 SECTION 46. HYPERBOLIC GEOMETRY
- Page 270 and 271: 266 SECTION 46. HYPERBOLIC GEOMETRY
- Page 272 and 273: 268 SECTION 46. HYPERBOLIC GEOMETRY
- Page 274 and 275: 270 SECTION 46. HYPERBOLIC GEOMETRY
- Page 276 and 277: 272SECTION 47.PERPENDICULAR LINES I
- Page 278 and 279: 274SECTION 47.PERPENDICULAR LINES I
- Page 280 and 281: 276 SECTION 48. PARALLEL LINES IN H
- Page 282 and 283: 278 SECTION 48. PARALLEL LINES IN H
- Page 284 and 285: 280 SECTION 48. PARALLEL LINES IN H
- Page 286 and 287: 282 SECTION 48. PARALLEL LINES IN H
- Page 288 and 289: 284 SECTION 49. TRIANGLES IN HYPERB
- Page 290 and 291: 286 SECTION 49. TRIANGLES IN HYPERB
- Page 292 and 293: 288 SECTION 49. TRIANGLES IN HYPERB
- Page 294 and 295: 290 SECTION 50. AREA IN HYPERBOLIC
- Page 296 and 297: 292 SECTION 50. AREA IN HYPERBOLIC
- Page 300 and 301: 296 SECTION 50. AREA IN HYPERBOLIC
- Page 302 and 303: 298 SECTION 51. THE POINCARE DISK M
- Page 304 and 305: 300 SECTION 51. THE POINCARE DISK M
- Page 306 and 307: 302 SECTION 51. THE POINCARE DISK M
- Page 308 and 309: 304 SECTION 51. THE POINCARE DISK M
- Page 310 and 311: 306 SECTION 52. ARC LENGTHAs we see
- Page 312 and 313: 308 SECTION 52. ARC LENGTHProof. Su
- Page 314 and 315: 310 SECTION 52. ARC LENGTHThus the
- Page 316 and 317: 312 SECTION 52. ARC LENGTHMeasuring
- Page 318 and 319: 314 SECTION 53. SPHERICAL GEOMETRYW
- Page 320 and 321: 316 SECTION 53. SPHERICAL GEOMETRYF
- Page 322 and 323: 318 SECTION 53. SPHERICAL GEOMETRYP
- Page 324 and 325: 320 APPENDIX A. SYMBOLS USEDAppendi
- Page 326 and 327: 322 APPENDIX A. SYMBOLS USEDTechnol
294 SECTION 50. AREA IN HYPERBOLIC GEOMETRYwhere the last step follows by additivity of defect. Henceby Boylai’s theorem and thererforehence△ABC ≡ △F P 0 P aα(△ABC) = α(△F P 0 P a )= aα(△F P i P i+1 )= a b α(△DEF )α(△ABC)α(△DEF ) = a b = δ(△ABC)δ(△DEF )which proves the theorem when the ratio is rational.Now suppose that x,δ(△ABC)δ(△DEF ) = xα(△ABC)α(△DEF ) = ywhere x and y are real numbers. Let r be any rational number such that0 < r < x. Choose C ′ ∈ BC such thatThenand by the first part of the theorem,r = δ(△ABC′ )δ(△DEF )α(△ABC ′ ) < α(△ABC)r = α(△ABC′ )α(△DEF )< α(△ABC)α(△DEF ) = ySince r was chosen as an arbitrary rational such that r < x, then for everyrational r < x, we have r < y.By a similar argument, for every rational number r < y, we can show thatr < x.Hence by the comparison theorem for real numbers (theorem 6.5), x =y.« CC BY-NC-ND 3.0. Revised: 18 Nov 2012