Plane Geometry - Bruce E. Shapiro

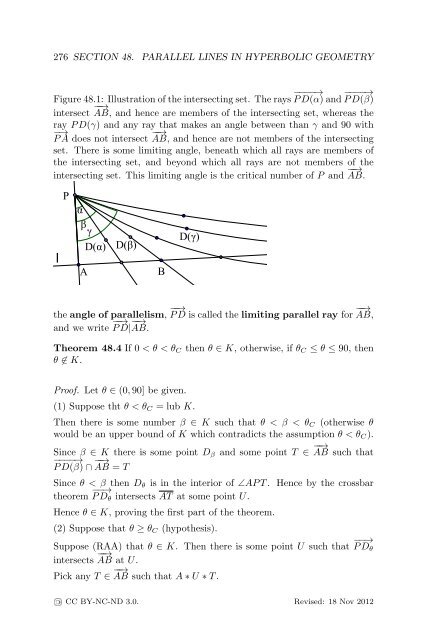
276 SECTION 48. PARALLEL LINES IN HYPERBOLIC GEOMETRYFigure 48.1: Illustration of the intersecting set. The rays −−−−→ P D(α) and −−−−→ P D(β)intersect −→ AB, and hence are members of the intersecting set, whereas theray P D(γ) and any ray that makes an angle between than γ and 90 with−→P A does not intersect −→ AB, and hence are not members of the intersectingset. There is some limiting angle, beneath which all rays are members ofthe intersecting set, and beyond which all rays are not members of theintersecting set. This limiting angle is the critical number of P and −→ AB.Pαβ γD(α)D(β)D(γ)ABthe angle of parallelism, −−→ P D is called the limiting parallel ray for −→ AB,and we write −−→ P D| −→ AB.Theorem 48.4 If 0 < θ < θ C then θ ∈ K, otherwise, if θ C ≤ θ ≤ 90, thenθ ∉ K.Proof. Let θ ∈ (0, 90] be given.(1) Suppose tht θ < θ C = lub K.Then there is some number β ∈ K such that θ < β < θ C (otherwise θwould be an upper bound of K which contradicts the assumption θ < θ C ).Since β ∈ K there is some point D β and some point T ∈ −→ AB such that−−−−→P D(β) ∩ −→ AB = TSince θ < β then D θ is in the interior of ∠AP T . Hence by the crossbartheorem −−→ P D θ intersects AT at some point U.Hence θ ∈ K, proving the first part of the theorem.(2) Suppose that θ ≥ θ C (hypothesis).Suppose (RAA) that θ ∈ K. Then there is some point U such that −−→ P D θintersects −→ AB at U.Pick any T ∈ −→ AB such that A ∗ U ∗ T .« CC BY-NC-ND 3.0. Revised: 18 Nov 2012