Plane Geometry - Bruce E. Shapiro

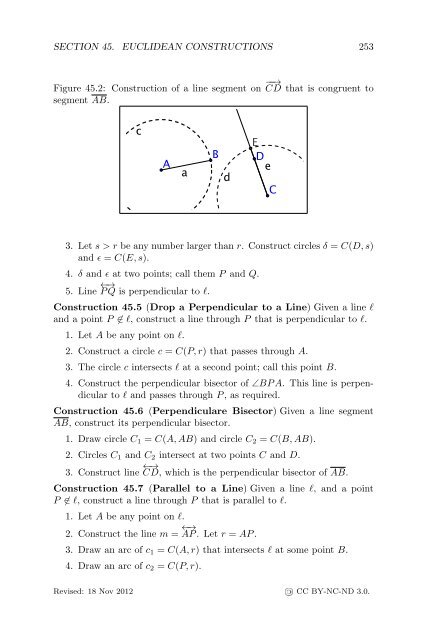
SECTION 45. EUCLIDEAN CONSTRUCTIONS 253Figure 45.2: Construction of a line segment on −−→ CD that is congruent tosegment AB.3. Let s > r be any number larger than r. Construct circles δ = C(D, s)and ɛ = C(E, s).4. δ and ɛ at two points; call them P and Q.5. Line ←→ P Q is perpendicular to l.Construction 45.5 (Drop a Perpendicular to a Line) Given a line land a point P ∉ l, construct a line through P that is perpendicular to l.1. Let A be any point on l.2. Construct a circle c = C(P, r) that passes through A.3. The circle c intersects l at a second point; call this point B.4. Construct the perpendicular bisector of ∠BP A. This line is perpendicularto l and passes through P , as required.Construction 45.6 (Perpendiculare Bisector) Given a line segmentAB, construct its perpendicular bisector.1. Draw circle C 1 = C(A, AB) and circle C 2 = C(B, AB).2. Circles C 1 and C 2 intersect at two points C and D.3. Construct line ←→ CD, which is the perpendicular bisector of AB.Construction 45.7 (Parallel to a Line) Given a line l, and a pointP ∉ l, construct a line through P that is parallel to l.1. Let A be any point on l.2. Construct the line m = ←→ AP . Let r = AP .3. Draw an arc of c 1 = C(A, r) that intersects l at some point B.4. Draw an arc of c 2 = C(P, r).Revised: 18 Nov 2012 « CC BY-NC-ND 3.0.