Plane Geometry - Bruce E. Shapiro
Plane Geometry - Bruce E. Shapiro Plane Geometry - Bruce E. Shapiro
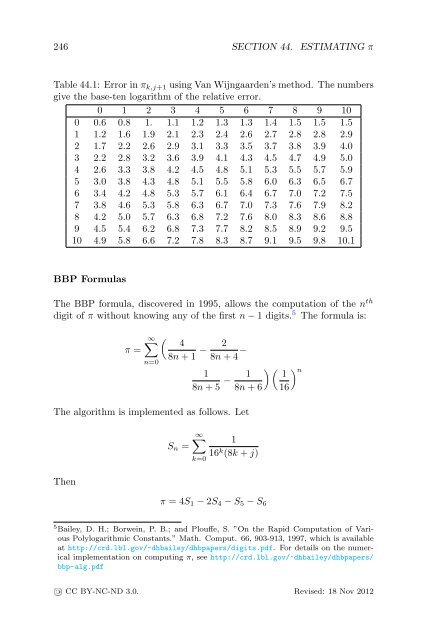
246 SECTION 44. ESTIMATING πTable 44.1: Error in π k,j+1 using Van Wijngaarden’s method. The numbersgive the base-ten logarithm of the relative error.0 1 2 3 4 5 6 7 8 9 100 0.6 0.8 1. 1.1 1.2 1.3 1.3 1.4 1.5 1.5 1.51 1.2 1.6 1.9 2.1 2.3 2.4 2.6 2.7 2.8 2.8 2.92 1.7 2.2 2.6 2.9 3.1 3.3 3.5 3.7 3.8 3.9 4.03 2.2 2.8 3.2 3.6 3.9 4.1 4.3 4.5 4.7 4.9 5.04 2.6 3.3 3.8 4.2 4.5 4.8 5.1 5.3 5.5 5.7 5.95 3.0 3.8 4.3 4.8 5.1 5.5 5.8 6.0 6.3 6.5 6.76 3.4 4.2 4.8 5.3 5.7 6.1 6.4 6.7 7.0 7.2 7.57 3.8 4.6 5.3 5.8 6.3 6.7 7.0 7.3 7.6 7.9 8.28 4.2 5.0 5.7 6.3 6.8 7.2 7.6 8.0 8.3 8.6 8.89 4.5 5.4 6.2 6.8 7.3 7.7 8.2 8.5 8.9 9.2 9.510 4.9 5.8 6.6 7.2 7.8 8.3 8.7 9.1 9.5 9.8 10.1BBP FormulasThe BBP formula, discovered in 1995, allows the computation of the n thdigit of π without knowing any of the first n − 1 digits. 5 The formula is:π =∞∑n=0Å 48n + 1 − 28n + 4 −18n + 5 − 1 ã Å ã 1 n8n + 6 16The algorithm is implemented as follows. LetThenS n =∞∑k=0116 k (8k + j)π = 4S 1 − 2S 4 − S 5 − S 65 Bailey, D. H.; Borwein, P. B.; and Plouffe, S. ”On the Rapid Computation of VariousPolylogarithmic Constants.” Math. Comput. 66, 903-913, 1997, which is availableat http://crd.lbl.gov/~dhbailey/dhbpapers/digits.pdf. For details on the numericalimplementation on computing π, see http://crd.lbl.gov/~dhbailey/dhbpapers/bbp-alg.pdf« CC BY-NC-ND 3.0. Revised: 18 Nov 2012
SECTION 44. ESTIMATING π 247Define the notation{x} = x mod 1, the fractional part of x. Then{ n}∑{16 n 16 n−k ∞S p } =8k + p +∑ 16 n−k8k + pk=0k=n+1{ n{∑ 16 n−k , 8k + p }=8k + pk=0}∞∑ 16 n−k+8k + pDefines p (n) =k=n+1{ n∑ 16 n−k , 8k + p }k=0Then the n th hex-digit of π is given by8k + pd n = ⌊16 {4{s 1 (n)}2{s 4 (n)} − {s 5 (n)}−{s 6 (n)}}⌋where ⌊x⌋ denotes the greatest integer less than or equal to x. The followinggives a Mathematica implementation:s[p_, n_]:= Sum[Mod[16^(n-k), 8k+p]/(8k+p),{k,0,n}];r[p_, n_]:= Mod[s[p,n],1];hexit[n_]:= Floor[16.0*Mod[4r[1,n]-2r[4,n]-r[5,n]-r[6,n], 1]];Thenhexit/@Range[0,11]gives the first 12 hex figures to the right hand side of the radix point:{2, 4, 3, 15, 6, 10, 8, 8, 8, 5, 10, 3}which corresponds toπ − 3 ≈ .243F 6A88 85A3 16The correct value of in hex to 16 figures isπ ≈ 3.243F 6A88 85A3 08D3 16To convert the number back to decimal, divide each integer by its correspondingpower of 16.Revised: 18 Nov 2012 « CC BY-NC-ND 3.0.
- Page 200 and 201: 196 SECTION 38. THE PYTHAGOREAN THE
- Page 202 and 203: 198 SECTION 38. THE PYTHAGOREAN THE
- Page 204 and 205: 200 SECTION 38. THE PYTHAGOREAN THE
- Page 206 and 207: 202 SECTION 39. CIRCLESFigure 39.1:
- Page 208 and 209: 204 SECTION 39. CIRCLESFigure 39.3:
- Page 210 and 211: 206 SECTION 39. CIRCLESFigure 39.5:
- Page 212 and 213: 208 SECTION 39. CIRCLESTheorem 39.1
- Page 214 and 215: 210 SECTION 39. CIRCLESLet D be the
- Page 216 and 217: 212 SECTION 39. CIRCLES« CC BY-NC-
- Page 218 and 219: 214 SECTION 40. CIRCLES AND TRIANGL
- Page 220 and 221: 216 SECTION 40. CIRCLES AND TRIANGL
- Page 222 and 223: 218 SECTION 40. CIRCLES AND TRIANGL
- Page 224 and 225: 220 SECTION 41. EUCLIDEAN CIRCLESFi
- Page 226 and 227: 222 SECTION 41. EUCLIDEAN CIRCLESFi
- Page 228 and 229: 224 SECTION 41. EUCLIDEAN CIRCLESFi
- Page 230 and 231: 226 SECTION 41. EUCLIDEAN CIRCLESFi
- Page 232 and 233: 228 SECTION 41. EUCLIDEAN CIRCLESBy
- Page 234 and 235: 230 SECTION 42. AREA AND CIRCUMFERE
- Page 236 and 237: 232 SECTION 42. AREA AND CIRCUMFERE
- Page 238 and 239: 234 SECTION 42. AREA AND CIRCUMFERE
- Page 240 and 241: 236 SECTION 42. AREA AND CIRCUMFERE
- Page 242 and 243: 238 SECTION 43. INDIANA BILL 246the
- Page 244 and 245: 240 SECTION 44. ESTIMATING πFigure
- Page 246 and 247: 242 SECTION 44. ESTIMATING πso tha
- Page 248 and 249: 244 SECTION 44. ESTIMATING πn π n
- Page 252 and 253: 248 SECTION 44. ESTIMATING πnums =
- Page 254 and 255: 250 SECTION 44. ESTIMATING π« CC
- Page 256 and 257: 252 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 258 and 259: 254 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 260 and 261: 256 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 262 and 263: 258 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 264 and 265: 260 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 266 and 267: 262 SECTION 45. EUCLIDEAN CONSTRUCT
- Page 268 and 269: 264 SECTION 46. HYPERBOLIC GEOMETRY
- Page 270 and 271: 266 SECTION 46. HYPERBOLIC GEOMETRY
- Page 272 and 273: 268 SECTION 46. HYPERBOLIC GEOMETRY
- Page 274 and 275: 270 SECTION 46. HYPERBOLIC GEOMETRY
- Page 276 and 277: 272SECTION 47.PERPENDICULAR LINES I
- Page 278 and 279: 274SECTION 47.PERPENDICULAR LINES I
- Page 280 and 281: 276 SECTION 48. PARALLEL LINES IN H
- Page 282 and 283: 278 SECTION 48. PARALLEL LINES IN H
- Page 284 and 285: 280 SECTION 48. PARALLEL LINES IN H
- Page 286 and 287: 282 SECTION 48. PARALLEL LINES IN H
- Page 288 and 289: 284 SECTION 49. TRIANGLES IN HYPERB
- Page 290 and 291: 286 SECTION 49. TRIANGLES IN HYPERB
- Page 292 and 293: 288 SECTION 49. TRIANGLES IN HYPERB
- Page 294 and 295: 290 SECTION 50. AREA IN HYPERBOLIC
- Page 296 and 297: 292 SECTION 50. AREA IN HYPERBOLIC
- Page 298 and 299: 294 SECTION 50. AREA IN HYPERBOLIC
246 SECTION 44. ESTIMATING πTable 44.1: Error in π k,j+1 using Van Wijngaarden’s method. The numbersgive the base-ten logarithm of the relative error.0 1 2 3 4 5 6 7 8 9 100 0.6 0.8 1. 1.1 1.2 1.3 1.3 1.4 1.5 1.5 1.51 1.2 1.6 1.9 2.1 2.3 2.4 2.6 2.7 2.8 2.8 2.92 1.7 2.2 2.6 2.9 3.1 3.3 3.5 3.7 3.8 3.9 4.03 2.2 2.8 3.2 3.6 3.9 4.1 4.3 4.5 4.7 4.9 5.04 2.6 3.3 3.8 4.2 4.5 4.8 5.1 5.3 5.5 5.7 5.95 3.0 3.8 4.3 4.8 5.1 5.5 5.8 6.0 6.3 6.5 6.76 3.4 4.2 4.8 5.3 5.7 6.1 6.4 6.7 7.0 7.2 7.57 3.8 4.6 5.3 5.8 6.3 6.7 7.0 7.3 7.6 7.9 8.28 4.2 5.0 5.7 6.3 6.8 7.2 7.6 8.0 8.3 8.6 8.89 4.5 5.4 6.2 6.8 7.3 7.7 8.2 8.5 8.9 9.2 9.510 4.9 5.8 6.6 7.2 7.8 8.3 8.7 9.1 9.5 9.8 10.1BBP FormulasThe BBP formula, discovered in 1995, allows the computation of the n thdigit of π without knowing any of the first n − 1 digits. 5 The formula is:π =∞∑n=0Å 48n + 1 − 28n + 4 −18n + 5 − 1 ã Å ã 1 n8n + 6 16The algorithm is implemented as follows. LetThenS n =∞∑k=0116 k (8k + j)π = 4S 1 − 2S 4 − S 5 − S 65 Bailey, D. H.; Borwein, P. B.; and Plouffe, S. ”On the Rapid Computation of VariousPolylogarithmic Constants.” Math. Comput. 66, 903-913, 1997, which is availableat http://crd.lbl.gov/~dhbailey/dhbpapers/digits.pdf. For details on the numericalimplementation on computing π, see http://crd.lbl.gov/~dhbailey/dhbpapers/bbp-alg.pdf« CC BY-NC-ND 3.0. Revised: 18 Nov 2012