Plane Geometry - Bruce E. Shapiro

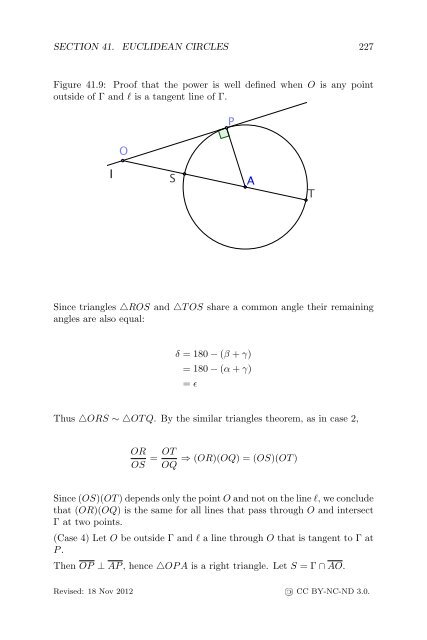
SECTION 41. EUCLIDEAN CIRCLES 227Figure 41.9: Proof that the power is well defined when O is any pointoutside of Γ and l is a tangent line of Γ.Since triangles △ROS and △T OS share a common angle their remainingangles are also equal:δ = 180 − (β + γ)= 180 − (α + γ)= ɛThus △ORS ∼ △OT Q. By the similar triangles theorem, as in case 2,OROS = OT ⇒ (OR)(OQ) = (OS)(OT )OQSince (OS)(OT ) depends only the point O and not on the line l, we concludethat (OR)(OQ) is the same for all lines that pass through O and intersectΓ at two points.(Case 4) Let O be outside Γ and l a line through O that is tangent to Γ atP .Then OP ⊥ AP , hence △OP A is a right triangle. Let S = Γ ∩ AO.Revised: 18 Nov 2012 « CC BY-NC-ND 3.0.