Plane Geometry - Bruce E. Shapiro

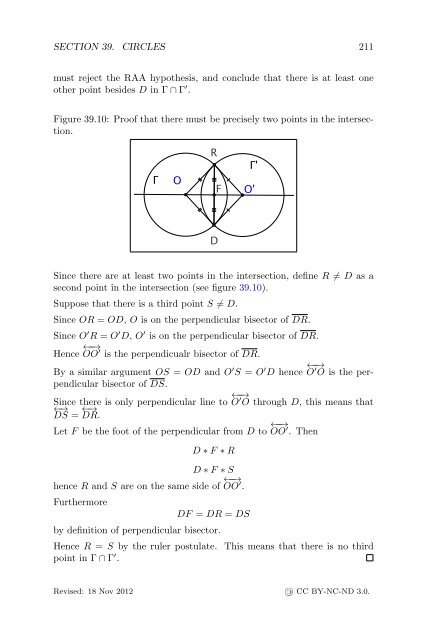
SECTION 39. CIRCLES 211must reject the RAA hypothesis, and conclude that there is at least oneother point besides D in Γ ∩ Γ ′ .Figure 39.10: Proof that there must be precisely two points in the intersection.Since there are at least two points in the intersection, define R ≠ D as asecond point in the intersection (see figure 39.10).Suppose that there is a third point S ≠ D.Since OR = OD, O is on the perpendicular bisector of DR.Since O ′ R = O ′ D, O ′ is on the perpendicular bisector of DR.Hence ←−→ OO ′ is the perpendicualr bisector of DR.By a similar argument OS = OD and O ′ S = O ′ D hence ←−→ O ′ O is the perpendicularbisector of DS.Since there is only perpendicular line to ←−→ O ′ O through D, this means that←→DS = ←→ DR.Let F be the foot of the perpendicular from D to ←−→ OO ′ . ThenD ∗ F ∗ RD ∗ F ∗ Shence R and S are on the same side of ←−→ OO ′ .FurthermoreDF = DR = DSby definition of perpendicular bisector.Hence R = S by the ruler postulate. This means that there is no thirdpoint in Γ ∩ Γ ′ .Revised: 18 Nov 2012 « CC BY-NC-ND 3.0.