Plane Geometry - Bruce E. Shapiro

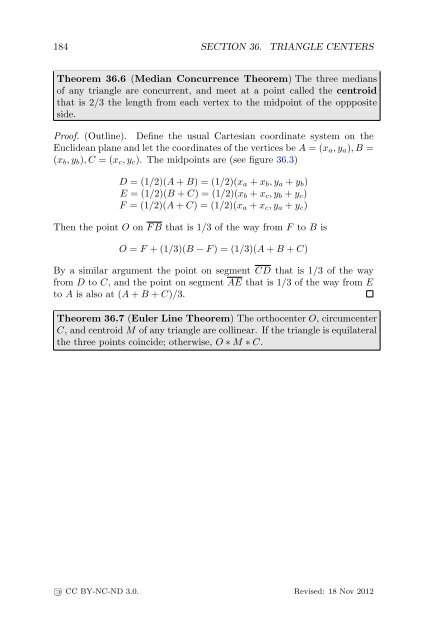
184 SECTION 36. TRIANGLE CENTERSTheorem 36.6 (Median Concurrence Theorem) The three mediansof any triangle are concurrent, and meet at a point called the centroidthat is 2/3 the length from each vertex to the midpoint of the opppositeside.Proof. (Outline). Define the usual Cartesian coordinate system on theEuclidean plane and let the coordinates of the vertices be A = (x a , y a ), B =(x b , y b ), C = (x c , y c ). The midpoints are (see figure 36.3)D = (1/2)(A + B) = (1/2)(x a + x b , y a + y b )E = (1/2)(B + C) = (1/2)(x b + x c , y b + y c )F = (1/2)(A + C) = (1/2)(x a + x c , y a + y c )Then the point O on F B that is 1/3 of the way from F to B isO = F + (1/3)(B − F ) = (1/3)(A + B + C)By a similar argument the point on segment CD that is 1/3 of the wayfrom D to C, and the point on segment AE that is 1/3 of the way from Eto A is also at (A + B + C)/3.Theorem 36.7 (Euler Line Theorem) The orthocenter O, circumcenterC, and centroid M of any triangle are collinear. If the triangle is equilateralthe three points coincide; otherwise, O ∗ M ∗ C.« CC BY-NC-ND 3.0. Revised: 18 Nov 2012