v2007.09.13 - Convex Optimization
v2007.09.13 - Convex Optimization v2007.09.13 - Convex Optimization
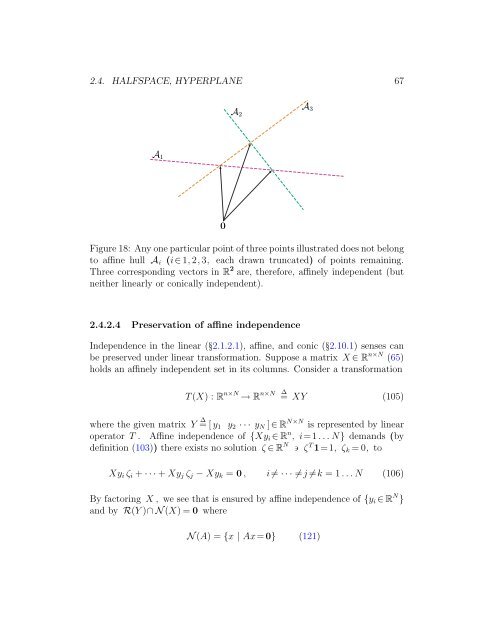
66 CHAPTER 2. CONVEX GEOMETRYHyperplanes in R mn may, of course, also be represented using matrixvariables.∂H = {Y | 〈A, Y 〉 = b} = {Y | 〈A, Y −Y p 〉 = 0} ⊂ R mn (100)Vector a from Figure 16 is normal to the hyperplane illustrated. Likewise,nonzero vectorized matrix A is normal to hyperplane ∂H ;2.4.2.2 Vertex-description of hyperplaneA ⊥ ∂H in R mn (101)Any hyperplane in R n may be described as the affine hull of a minimal set ofpoints {x l ∈ R n , l = 1... n} arranged columnar in a matrix X ∈ R n×n (65):∂H = aff{x l ∈ R n , l = 1... n} ,dim aff{x l ∀l}=n−1= aff X , dim aff X = n−1= x 1 + R{x l − x 1 , l=2... n} , dim R{x l − x 1 , l=2... n}=n−1= x 1 + R(X − x 1 1 T ) , dim R(X − x 1 1 T ) = n−1whereR(A) = {Ax | ∀x} (120)(102)2.4.2.3 Affine independence, minimal setFor any particular affine set, a minimal set of points constituting itsvertex-description is an affinely independent descriptive set and vice versa.Arbitrary given points {x i ∈ R n , i=1... N} are affinely independent(a.i.) if and only if, over all ζ ∈ R N ζ T 1=1, ζ k = 0 (confer2.1.2)x i ζ i + · · · + x j ζ j − x k = 0, i≠ · · · ≠j ≠k = 1... N (103)has no solution ζ ; in words, iff no point from the given set can be expressedas an affine combination of those remaining. We deducel.i. ⇒ a.i. (104)Consequently, {x i , i=1... N} is an affinely independent set if and only if{x i −x 1 , i=2... N} is a linearly independent (l.i.) set. [ [152,3] ] (Figure 18)XThis is equivalent to the property that the columns of1 T (for X ∈ R n×Nas in (65)) form a linearly independent set. [147,A.1.3]
2.4. HALFSPACE, HYPERPLANE 67A 1A 2A 30Figure 18: Any one particular point of three points illustrated does not belongto affine hull A i (i∈1, 2, 3, each drawn truncated) of points remaining.Three corresponding vectors in R 2 are, therefore, affinely independent (butneither linearly or conically independent).2.4.2.4 Preservation of affine independenceIndependence in the linear (2.1.2.1), affine, and conic (2.10.1) senses canbe preserved under linear transformation. Suppose a matrix X ∈ R n×N (65)holds an affinely independent set in its columns. Consider a transformationT(X) : R n×N → R n×N ∆ = XY (105)where the given matrix Y ∆ = [y 1 y 2 · · · y N ]∈ R N×N is represented by linearoperator T . Affine independence of {Xy i ∈ R n , i=1... N} demands (bydefinition (103)) there exists no solution ζ ∈ R N ζ T 1=1, ζ k = 0, toXy i ζ i + · · · + Xy j ζ j − Xy k = 0, i≠ · · · ≠j ≠k = 1... N (106)By factoring X , we see that is ensured by affine independence of {y i ∈ R N }and by R(Y )∩ N(X) = 0 whereN(A) = {x | Ax=0} (121)
- Page 15 and 16: LIST OF FIGURES 1559 Quadratic func
- Page 17 and 18: LIST OF FIGURES 17E Projection 5791
- Page 19 and 20: Chapter 1OverviewConvex Optimizatio
- Page 21 and 22: ˇx 4ˇx 3ˇx 2Figure 2: Applicatio
- Page 23 and 24: 23Figure 4: This coarsely discretiz
- Page 25 and 26: ases (biorthogonal expansion). We e
- Page 27 and 28: 27Figure 7: These bees construct a
- Page 29 and 30: its membership to the EDM cone. The
- Page 31 and 32: 31appendicesProvided so as to be mo
- Page 33 and 34: Chapter 2Convex geometryConvexity h
- Page 35 and 36: 2.1. CONVEX SET 35Figure 9: A slab
- Page 37 and 38: 2.1. CONVEX SET 372.1.6 empty set v
- Page 39 and 40: 2.1. CONVEX SET 392.1.7.1 Line inte
- Page 41 and 42: 2.1. CONVEX SET 41(a)R 2(b)R 3(c)(d
- Page 43 and 44: 2.1. CONVEX SET 43This theorem in c
- Page 45 and 46: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 47 and 48: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 49 and 50: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 51 and 52: 2.2. VECTORIZED-MATRIX INNER PRODUC
- Page 53 and 54: 2.3. HULLS 53Figure 12: Convex hull
- Page 55 and 56: 2.3. HULLS 55Aaffine hull (drawn tr
- Page 57 and 58: 2.3. HULLS 57The union of relative
- Page 59 and 60: 2.4. HALFSPACE, HYPERPLANE 59of dim
- Page 61 and 62: 2.4. HALFSPACE, HYPERPLANE 61H +ay
- Page 63 and 64: 2.4. HALFSPACE, HYPERPLANE 63Inters
- Page 65: 2.4. HALFSPACE, HYPERPLANE 65Conver
- Page 69 and 70: 2.4. HALFSPACE, HYPERPLANE 69tradit
- Page 71 and 72: 2.4. HALFSPACE, HYPERPLANE 71There
- Page 73 and 74: 2.5. SUBSPACE REPRESENTATIONS 732.5
- Page 75 and 76: 2.5. SUBSPACE REPRESENTATIONS 752.5
- Page 77 and 78: 2.6. EXTREME, EXPOSED 77In other wo
- Page 79 and 80: 2.6. EXTREME, EXPOSED 792.6.1 Expos
- Page 81 and 82: 2.7. CONES 812.6.1.3.1 Definition.
- Page 83 and 84: 2.7. CONES 830Figure 24: Boundary o
- Page 85 and 86: 2.7. CONES 852.7.2 Convex coneWe ca
- Page 87 and 88: 2.7. CONES 87Thus the simplest and
- Page 89 and 90: 2.7. CONES 89nomenclature generaliz
- Page 91 and 92: 2.8. CONE BOUNDARY 91Proper cone {0
- Page 93 and 94: 2.8. CONE BOUNDARY 93the same extre
- Page 96 and 97: 96 CHAPTER 2. CONVEX GEOMETRYBCADFi
- Page 98 and 99: 98 CHAPTER 2. CONVEX GEOMETRYThe po
- Page 100 and 101: 100 CHAPTER 2. CONVEX GEOMETRY2.9.0
- Page 102 and 103: 102 CHAPTER 2. CONVEX GEOMETRYwhere
- Page 104 and 105: 104 CHAPTER 2. CONVEX GEOMETRY√2
- Page 106 and 107: 106 CHAPTER 2. CONVEX GEOMETRYwhich
- Page 108 and 109: 108 CHAPTER 2. CONVEX GEOMETRY2.9.2
- Page 110: 110 CHAPTER 2. CONVEX GEOMETRYA con
- Page 115 and 116: 2.9. POSITIVE SEMIDEFINITE (PSD) CO
2.4. HALFSPACE, HYPERPLANE 67A 1A 2A 30Figure 18: Any one particular point of three points illustrated does not belongto affine hull A i (i∈1, 2, 3, each drawn truncated) of points remaining.Three corresponding vectors in R 2 are, therefore, affinely independent (butneither linearly or conically independent).2.4.2.4 Preservation of affine independenceIndependence in the linear (2.1.2.1), affine, and conic (2.10.1) senses canbe preserved under linear transformation. Suppose a matrix X ∈ R n×N (65)holds an affinely independent set in its columns. Consider a transformationT(X) : R n×N → R n×N ∆ = XY (105)where the given matrix Y ∆ = [y 1 y 2 · · · y N ]∈ R N×N is represented by linearoperator T . Affine independence of {Xy i ∈ R n , i=1... N} demands (bydefinition (103)) there exists no solution ζ ∈ R N ζ T 1=1, ζ k = 0, toXy i ζ i + · · · + Xy j ζ j − Xy k = 0, i≠ · · · ≠j ≠k = 1... N (106)By factoring X , we see that is ensured by affine independence of {y i ∈ R N }and by R(Y )∩ N(X) = 0 whereN(A) = {x | Ax=0} (121)