v2007.09.13 - Convex Optimization

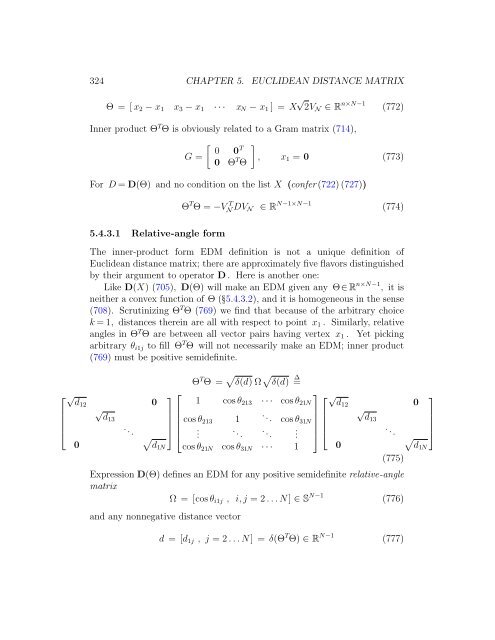
324 CHAPTER 5. EUCLIDEAN DISTANCE MATRIXΘ = [x 2 − x 1 x 3 − x 1 · · · x N − x 1 ] = X √ 2V N ∈ R n×N−1 (772)Inner product Θ T Θ is obviously related to a Gram matrix (714),[ 0 0TG =0 Θ T Θ], x 1 = 0 (773)For D = D(Θ) and no condition on the list X (confer (722) (727))Θ T Θ = −V T NDV N ∈ R N−1×N−1 (774)5.4.3.1 Relative-angle formThe inner-product form EDM definition is not a unique definition ofEuclidean distance matrix; there are approximately five flavors distinguishedby their argument to operator D . Here is another one:Like D(X) (705), D(Θ) will make an EDM given any Θ∈ R n×N−1 , it isneither a convex function of Θ (5.4.3.2), and it is homogeneous in the sense(708). Scrutinizing Θ T Θ (769) we find that because of the arbitrary choicek = 1, distances therein are all with respect to point x 1 . Similarly, relativeangles in Θ T Θ are between all vector pairs having vertex x 1 . Yet pickingarbitrary θ i1j to fill Θ T Θ will not necessarily make an EDM; inner product(769) must be positive semidefinite.Θ T Θ = √ δ(d) Ω √ δ(d) =∆⎡√ ⎤⎡⎤⎡√ ⎤d12 0 1 cos θ 213 · · · cos θ 21N d12 0√ d13 ⎢⎥cosθ 213 1... cos θ √31Nd13 ⎣ ... ⎦⎢⎥⎢⎥√ ⎣ ....... . ⎦⎣... ⎦√0d1N cos θ 21N cos θ 31N · · · 1 0d1N(775)Expression D(Θ) defines an EDM for any positive semidefinite relative-anglematrixΩ = [cos θ i1j , i,j = 2...N] ∈ S N−1 (776)and any nonnegative distance vectord = [d 1j , j = 2...N] = δ(Θ T Θ) ∈ R N−1 (777)