v2007.09.13 - Convex Optimization

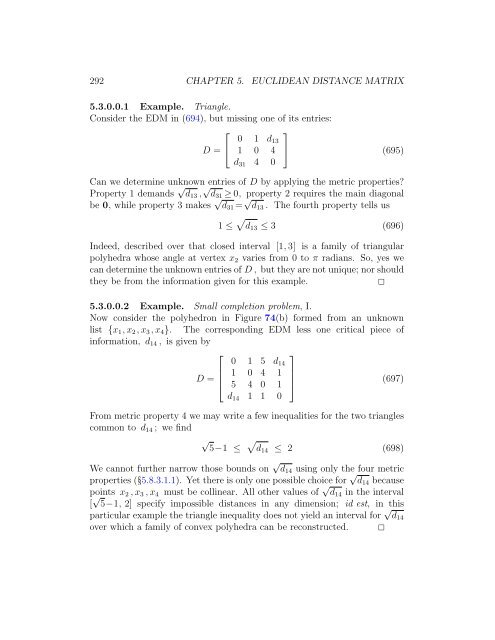
292 CHAPTER 5. EUCLIDEAN DISTANCE MATRIX5.3.0.0.1 Example. Triangle.Consider the EDM in (694), but missing one of its entries:⎡0⎤1 d 13D = ⎣ 1 0 4 ⎦ (695)d 31 4 0Can we determine unknown entries of D by applying the metric properties?Property 1 demands √ d 13 , √ d 31 ≥ 0, property 2 requires the main diagonalbe 0, while property 3 makes √ d 31 = √ d 13 . The fourth property tells us1 ≤ √ d 13 ≤ 3 (696)Indeed, described over that closed interval [1, 3] is a family of triangularpolyhedra whose angle at vertex x 2 varies from 0 to π radians. So, yes wecan determine the unknown entries of D , but they are not unique; nor shouldthey be from the information given for this example.5.3.0.0.2 Example. Small completion problem, I.Now consider the polyhedron in Figure 74(b) formed from an unknownlist {x 1 ,x 2 ,x 3 ,x 4 }. The corresponding EDM less one critical piece ofinformation, d 14 , is given by⎡⎤0 1 5 d 14D = ⎢ 1 0 4 1⎥⎣ 5 4 0 1 ⎦ (697)d 14 1 1 0From metric property 4 we may write a few inequalities for the two trianglescommon to d 14 ; we find√5−1 ≤√d14 ≤ 2 (698)We cannot further narrow those bounds on √ d 14 using only the four metricproperties (5.8.3.1.1). Yet there is only one possible choice for √ d 14 becausepoints x 2 ,x 3 ,x 4 must be collinear. All other values of √ d 14 in the interval[ √ 5−1, 2] specify impossible distances in any dimension; id est, in thisparticular example the triangle inequality does not yield an interval for √ d 14over which a family of convex polyhedra can be reconstructed.