v2007.09.13 - Convex Optimization
Share Embed Flag
Download ePaper

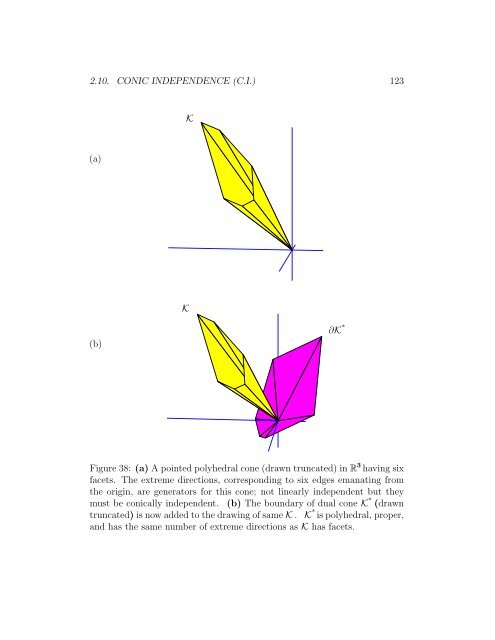
v2007.09.13 - Convex Optimization

v2007.09.13 - Convex Optimization v2007.09.13 - Convex Optimization

convexoptimization.com
from convexoptimization.com More from this publisher
10.07.2015 Views

122 CHAPTER 2. CONVEX GEOMETRY2.10.0.0.2 Exercise. Conically independent columns and rows.We suspect the number of conically independent columns (rows) of X tobe the same for X †T , where † denotes matrix pseudoinverse (E). Provewhether it holds that the columns (rows) of X are c.i. ⇔ the columns (rows)of X †T are c.i.2.10.1 Preservation of conic independenceIndependence in the linear (2.1.2.1), affine (2.4.2.4), and conic senses canbe preserved under linear transformation. Suppose a matrix X ∈ R n×N (240)holds a conically independent set columnar. Consider the transformationT(X) : R n×N → R n×N ∆ = XY (242)where the given matrix Y = ∆ [y 1 y 2 · · · y N ]∈ R N×N is represented by linearoperator T . Conic independence of {Xy i ∈ R n , i=1... N} demands, bydefinition (239),Xy i ζ i + · · · + Xy j ζ j − Xy l ζ l = 0, i≠ · · · ≠j ≠l = 1... N (243)have no nontrivial solution ζ ∈ R N + . That is ensured by conic independenceof {y i ∈ R N } and by R(Y )∩ N(X) = 0 ; seen by factoring X .2.10.1.1 linear maps of cones[18,7] If K is a convex cone in Euclidean space R and T is any linearmapping from R to Euclidean space M , then T(K) is a convex cone in Mand x ≼ y with respect to K implies T(x) ≼ T(y) with respect to T(K).If K is closed or has nonempty interior in R , then so is T(K) in M .If T is a linear bijection, then x ≼ y ⇔ T(x) ≼ T(y). Further, if F isa face of K , then T(F) is a face of T(K).2.10.2 Pointed closed convex K & conic independenceThe following bullets can be derived from definitions (155) and (239) inconjunction with the extremes theorem (2.8.1.1.1):The set of all extreme directions from a pointed closed convex coneK ⊂ R n is not necessarily a linearly independent set, yet it must be a conicallyindependent set; (compare Figure 15 on page 60 with Figure 38(a))

2.10. CONIC INDEPENDENCE (C.I.) 123K(a)K(b)∂K ∗Figure 38: (a) A pointed polyhedral cone (drawn truncated) in R 3 having sixfacets. The extreme directions, corresponding to six edges emanating fromthe origin, are generators for this cone; not linearly independent but theymust be conically independent. (b) The boundary of dual cone K ∗ (drawntruncated) is now added to the drawing of same K . K ∗ is polyhedral, proper,and has the same number of extreme directions as K has facets.

2.10. CONIC INDEPENDENCE (C.I.) 123K(a)K(b)∂K ∗Figure 38: (a) A pointed polyhedral cone (drawn truncated) in R 3 having sixfacets. The extreme directions, corresponding to six edges emanating fromthe origin, are generators for this cone; not linearly independent but theymust be conically independent. (b) The boundary of dual cone K ∗ (drawntruncated) is now added to the drawing of same K . K ∗ is polyhedral, proper,and has the same number of extreme directions as K has facets.

logo

products

Resources

Company

Terms of service
Privacy policy
Cookie policy
Cookie settings
Imprint
Change language
Made with love in Switzerland
© 2024 Yumpu.com all rights reserved

Hooray! Your file is uploaded and ready to be published.

Saved successfully!

Ooh no, something went wrong!