Chapter 1 Topics in Analytic Geometry

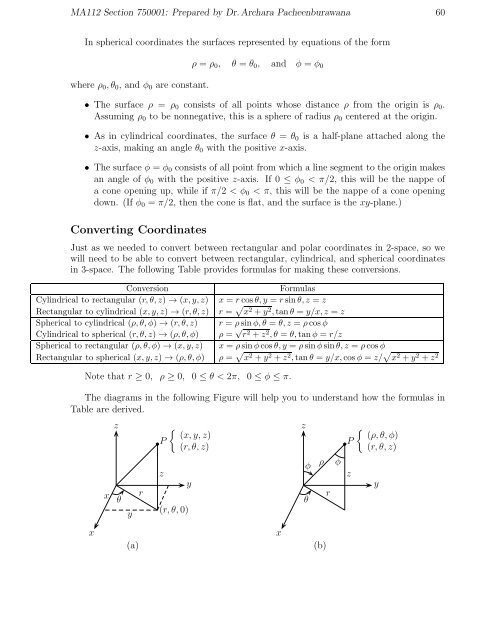
MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 60In spherical coordinates the surfaces represented by equations of the formwhere ρ 0 ,θ 0 , and φ 0 are constant.ρ = ρ 0 , θ = θ 0 , and φ = φ 0• The surface ρ = ρ 0 consists of all points whose distance ρ from the origin is ρ 0 .Assuming ρ 0 to be nonnegative, this is a sphere of radius ρ 0 centered at the origin.• As in cylindrical coordinates, the surface θ = θ 0 is a half-plane attached along thez-axis, making an angle θ 0 with the positive x-axis.• The surface φ = φ 0 consists of all point from which a line segment to the origin makesan angle of φ 0 with the positive z-axis. If 0 ≤ φ 0 < π/2, this will be the nappe ofa cone opening up, while if π/2 < φ 0 < π, this will be the nappe of a cone openingdown. (If φ 0 = π/2, then the cone is flat, and the surface is the xy-plane.)Converting CoordinatesJust as we needed to convert between rectangular and polar coordinates in 2-space, so wewill need to be able to convert between rectangular, cylindrical, and spherical coordinatesin 3-space. The following Table provides formulas for making these conversions.ConversionFormulasCylindrical to rectangular (r,θ,z) → (x,y,z) x = rcosθ,y = rsinθ,z = zRectangular to cylindrical (x,y,z) → (r,θ,z) r = √ x 2 +y 2 ,tanθ = y/x,z = zSpherical to cylindrical (ρ,θ,φ) → (r,θ,z) r = ρsinφ,θ = θ,z = ρcosφCylindrical to spherical (r,θ,z) → (ρ,θ,φ) ρ = √ r 2 +z 2 ,θ = θ,tanφ = r/zSpherical to rectangular (ρ,θ,φ) → (x,y,z) x = ρsinφcosθ,y = ρsinφsinθ,z = ρcosφRectangular to spherical (x,y,z) → (ρ,θ,φ) ρ = √ x 2 +y 2 +z 2 ,tanθ = y/x,cosφ = z/ √ x 2 +y 2 +z 2Note that r ≥ 0, ρ ≥ 0, 0 ≤ θ < 2π, 0 ≤ φ ≤ π.The diagrams in the following Figure will help you to understand how the formulas inTable are derived.xzθyr•Pz{(x,y,z)(r,θ,z)(r,θ,0)yzφθρrφ•Pz{(ρ,θ,φ)(r,θ,z)yx(a)x(b)