Chapter 1 Topics in Analytic Geometry

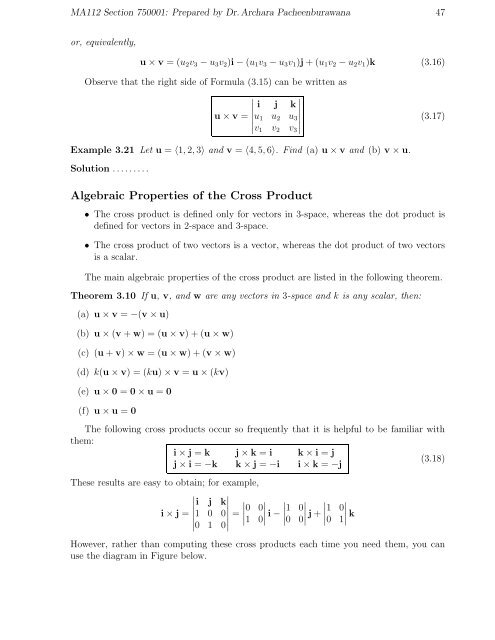
MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 47or, equivalently,u×v = (u 2 v 3 −u 3 v 2 )i−(u 1 v 3 −u 3 v 1 )j+(u 1 v 2 −u 2 v 1 )k (3.16)Observe that the right side of Formula (3.15) can be written as∣ i j k ∣∣∣∣∣u×v =u 1 u 2 u 3(3.17)∣v 1 v 2 v 3Example 3.21 Let u = 〈1,2,3〉 and v = 〈4,5,6〉. Find (a) u×v and (b) v×u.Solution .........Algebraic Properties of the Cross Product• The cross product is defined only for vectors in 3-space, whereas the dot product isdefined for vectors in 2-space and 3-space.• The cross product of two vectors is a vector, whereas the dot product of two vectorsis a scalar.The main algebraic properties of the cross product are listed in the following theorem.Theorem 3.10 If u, v, and w are any vectors in 3-space and k is any scalar, then:(a) u×v = −(v ×u)(b) u×(v+w) = (u×v)+(u×w)(c) (u+v)×w = (u×w)+(v×w)(d) k(u×v) = (ku)×v = u×(kv)(e) u×0 = 0×u = 0(f) u×u = 0The following cross products occur so frequently that it is helpful to be familiar withthem:i×j = k j×k = i k×i = j(3.18)j×i = −k k×j = −i i×k = −jThese results are easy to obtain; for example,i j k∣ ∣ ∣ ∣∣∣i×j =1 0 0∣0 1 0∣ = 0 0∣∣∣ 1 0∣ i− 1 0∣∣∣ 0 0∣ j+ 1 00 1∣ kHowever, rather than computing these cross products each time you need them, you canuse the diagram in Figure below.