Chapter 1 Topics in Analytic Geometry

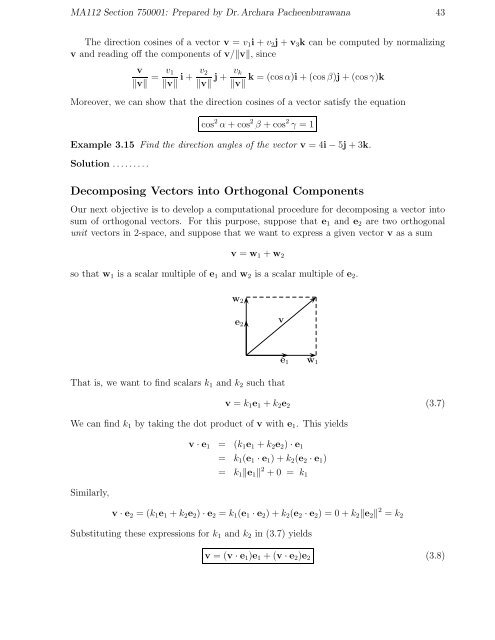
MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 43The direction cosines of a vector v = v 1 i+v 2 j+v 3 k can be computed by normalizingv and reading off the components of v/‖v‖, sincev‖v‖ = v 1‖v‖ i+ v 2‖v‖ j+ v k‖v‖ k = (cosα)i+(cosβ)j+(cosγ)kMoreover, we can show that the direction cosines of a vector satisfy the equationcos 2 α+cos 2 β +cos 2 γ = 1Example 3.15 Find the direction angles of the vector v = 4i−5j+3k.Solution .........Decomposing Vectors into Orthogonal ComponentsOur next objective is to develop a computational procedure for decomposing a vector intosum of orthogonal vectors. For this purpose, suppose that e 1 and e 2 are two orthogonalunit vectors in 2-space, and suppose that we want to express a given vector v as a sumv = w 1 +w 2so that w 1 is a scalar multiple of e 1 and w 2 is a scalar multiple of e 2 .w 2e 2ve 1 w 1That is, we want to find scalars k 1 and k 2 such thatv = k 1 e 1 +k 2 e 2 (3.7)We can find k 1 by taking the dot product of v with e 1 . This yieldsSimilarly,v·e 1 = (k 1 e 1 +k 2 e 2 )·e 1= k 1 (e 1 ·e 1 )+k 2 (e 2 ·e 1 )= k 1 ‖e 1 ‖ 2 +0 = k 1v·e 2 = (k 1 e 1 +k 2 e 2 )·e 2 = k 1 (e 1 ·e 2 )+k 2 (e 2 ·e 2 ) = 0+k 2 ‖e 2 ‖ 2 = k 2Substituting these expressions for k 1 and k 2 in (3.7) yieldsv = (v·e 1 )e 1 +(v·e 2 )e 2 (3.8)