Chapter 1 Topics in Analytic Geometry

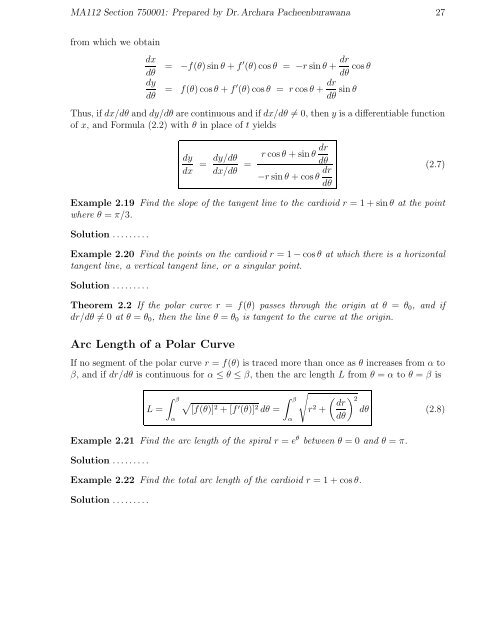
MA112 Section 750001: Prepared by Dr.Archara Pacheenburawana 27from which we obtaindxdθdydθ= −f(θ)sinθ+f ′ (θ)cosθ = −rsinθ+ drdθ cosθ= f(θ)cosθ+f ′ (θ)cosθ = rcosθ+ drdθ sinθThus, if dx/dθ and dy/dθ arecontinuous and if dx/dθ ≠ 0, then y is a differentiable functionof x, and Formula (2.2) with θ in place of t yieldsdydx = dy/dθ rcosθ +sinθ drdx/dθ = dθ−rsinθ +cosθ drdθ(2.7)Example 2.19 Find the slope of the tangent line to the cardioid r = 1+sinθ at the pointwhere θ = π/3.Solution .........Example 2.20 Find the points on the cardioid r = 1−cosθ at which there is a horizontaltangent line, a vertical tangent line, or a singular point.Solution .........Theorem 2.2 If the polar curve r = f(θ) passes through the origin at θ = θ 0 , and ifdr/dθ ≠ 0 at θ = θ 0 , then the line θ = θ 0 is tangent to the curve at the origin.Arc Length of a Polar CurveIf no segment of the polar curve r = f(θ) is traced more than once as θ increases from α toβ, and if dr/dθ is continuous for α ≤ θ ≤ β, then the arc length L from θ = α to θ = β isL =∫ βα√[f(θ)]2 +[f ′ (θ)] 2 dθ =∫ βα√r 2 +( ) 2 drdθ (2.8)dθExample 2.21 Find the arc length of the spiral r = e θ between θ = 0 and θ = π.Solution .........Example 2.22 Find the total arc length of the cardioid r = 1+cosθ.Solution .........