2. The Classical Single-degree-of-freedom System

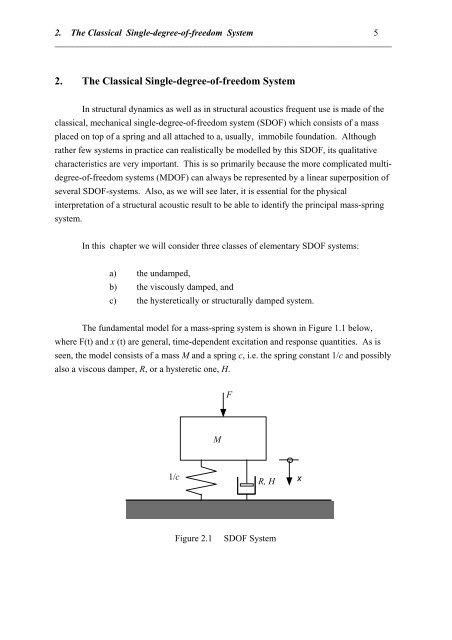
2. The Classical Single-degree-of-freedom System 5__________________________________________________________________________2. The Classical Single-degree-of-freedom SystemIn structural dynamics as well as in structural acoustics frequent use is made of theclassical, mechanical single-degree-of-freedom system (SDOF) which consists of a massplaced on top of a spring and all attached to a, usually, immobile foundation. Althoughrather few systems in practice can realistically be modelled by this SDOF, its qualitativecharacteristics are very important. This is so primarily because the more complicated multidegree-of-freedomsystems (MDOF) can always be represented by a linear superposition ofseveral SDOF-systems. Also, as we will see later, it is essential for the physicalinterpretation of a structural acoustic result to be able to identify the principal mass-springsystem.In this chapter we will consider three classes of elementary SDOF systems:a) the undamped,b) the viscously damped, andc) the hysteretically or structurally damped system.The fundamental model for a mass-spring system is shown in Figure 1.1 below,where F(t) and x (t) are general, time-dependent excitation and response quantities. As isseen, the model consists of a mass M and a spring c, i.e. the spring constant 1/c and possiblyalso a viscous damper, R, or a hysteretic one, H.FM1/cR, HxFigure 2.1SDOF System

2. The Classical Single-degree-of-freedom System 6__________________________________________________________________________2.1 The Undamped SystemIn the homogeneous case, i.e. where an external force is missing, the governingequation can be written as:M &x + 1 c x = 0 (2.1)If we assume a solution on the form x(t) = x ˆ e iωtthen the condition,1c − ω 2 M = 0 (2.2)results. Since both 1/c and M are given, the equation furnishes a condition for ω which alsois the solution,ω o = 1/ cM (2.3)This solution, of course, is the natural frequency with which the system vibrates.Let us now consider the so-called frequency response function for the classicalsystem.We will consider an excitation of the formF(t) =)F e iωt (2.4)and assume, as before, the solution x(t) = xe ) iωt . Instead of the homogeneous differentialequation (2.1) we obtain now the inhomogeneous, i.e.M &x + 1 c x = F(t)(2.1a)and we can immediately write the frequency response function for the system asxF =c1 −ω 2 Mc= N(ω ) (2.5)

2. The Classical Single-degree-of-freedom System 8__________________________________________________________________________The damping ratio is the ratio of the actual viscous damping and the so-called criticaldamping, given by R o= 2 M / c . This means that the system has a displacement which canbe written as:x(t) = ˆx e −ω oβt e iω o1−β 2 t= ˆx e −αt e i ω o ′ t (2.11)It is seen from equation (2.11) that the vibration has a complex eigen-frequency of which theimaginary part describes the harmonic motion and the real, the amplitude decay, see Fig. 2.2.x(t)Re[eiω ο t]te -αtFigure 2.2Free vibration of a damped SDOF systemWhen we consider the forced response, we assume a force function as above but proceeddirectly to the mobility representation, i.e.⎛iω M + R − iω ⎞⎝⎜ω 2 c⎠⎟ ˆv e iωt = ˆF e iωt (2.12)This leads to the mobility,Y(ω) =⎛iω M −⎝1(2.13)1 ⎞ω 2 c ⎠ + R

2. The Classical Single-degree-of-freedom System 9__________________________________________________________________________2.3 The Structurally Damped SystemA closer look at the behaviour of real structures shows that the viscous dampingmodel is not particularly representative. One finds often a frequency dependence of thedamping of real structures which cannot be reproduced by the viscous damper.An alternative is offered by the hysteretic model, also denoted the structural dampingmodel. This not only has the advantage of reproducing the damping realistically but alsosimplifies the analysis of real systems. It must be emphasised, however, that the hystereticmodel can introduce difficulties if one attempts a complete analysis of the free vibrations of asystem. This is due to the fact that the model is based on the relaxation in a material (amemory function) which can produce non-causal effects in conjunction with free vibrations.We will therefore confine ourselves to handle the inhomogeneous case, i.e. where an externalforce is applied to the system. In such a case the governing equation can be written as:⎜⎛iωM +⎝( )1+ iη ⎞viωc ⎠ ˆ e iωt= ˆ F e iωt (2.14)where η is the so called loss-factor such that, in Figure 2.1, H = η c .Accordingly the mobility is found to be given byY(ω ) =1⎛iω ⎜ M − 1 + iη ⎞⎝ ω 2 ⎟c ⎠(2.15)for the structurally damped system.In the discussion above we have, in fact, introduced a complex stiffness for which theimaginary part corresponds to the so-called loss factor of the system. This form of structuraldamping is denoted stiffness-proportional, structural damping and is frequently used instructural dynamics and structural acoustics. For a detailed examination of the concept ofcomplex stiffness the reader is referred to [1] and [2].The expression for the mobility in eqn. (2.15) can be re-written asY(ω) =⎛iω M −⎝11 ⎞ω 2 c ⎠ + ηωc(2.16)

2. The Classical Single-degree-of-freedom System 10__________________________________________________________________________From a comparison of equations (2.13) and (2.16) it is seen that for the SDOF systemthe viscous damping and the structural damping can be made analogous if R = η/ωc.