Solving Open Sentences Involving Absolute Value 7-6
Solving Open Sentences Involving Absolute Value 7-6
Solving Open Sentences Involving Absolute Value 7-6
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
OBJECTIVE:<br />
You will be able to solve open sentences involving absolute value and<br />
graph the solutions.<br />
We need to start with a discussion of what absolute value means.<br />
<strong>Absolute</strong> value is a means of determining the distance from zero.<br />
If I ask for the absolute value of 4, the answer is “4” since 4 is four units<br />
away from zero.<br />
If I ask for the absolute value of -4, the answer is “4” since -4 is four<br />
units away from zero.<br />
|4| = 4 |-4| = 4<br />
© William James Calhoun, 2001<br />
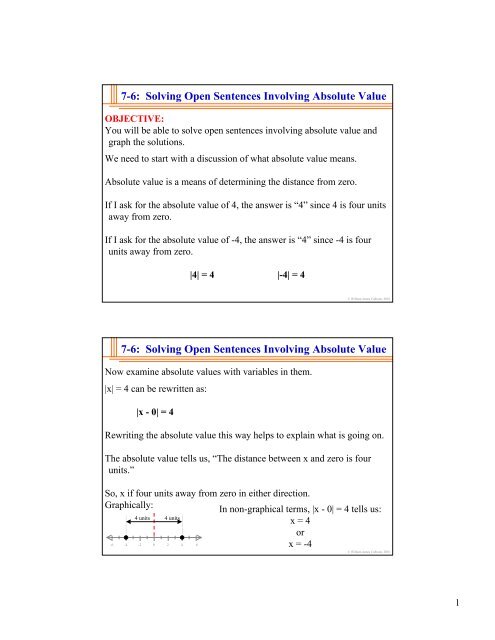
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
Now examine absolute values with variables in them.<br />
|x| = 4 can be rewritten as:<br />
|x - 0| = 4<br />
Rewriting the absolute value this way helps to explain what is going on.<br />
The absolute value tells us, “The distance between x and zero is four<br />
units.”<br />
So, x if four units away from zero in either direction.<br />
Graphically:<br />
4 units 4 units<br />
In non-graphical terms, |x - 0| = 4 tells us:<br />
x = 4<br />
or<br />
-6 -4 -2 0 2 4 6<br />
x = -4<br />
© William James Calhoun, 2001<br />
1
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
EXAMPLE 1: Solve |x - 3| = 5.<br />
Use the definition of absolute value.<br />
This problem is saying, “The distance between x and 3 is 5 units.”<br />
Graphically:<br />
Run both directions 5 units.<br />
5 units 5 units<br />
-3 -1 1 3 5 7 9<br />
Center on the 3.<br />
The solution is then:<br />
x = -2 or x = 8<br />
{-2, 8}<br />
Another way to solve this problem without graphing it first follows.<br />
The problem tells us that the distance between x and 3 is 5 units, so we know either:<br />
x - 3 = 5<br />
+3 +3<br />
or<br />
solve<br />
x - 3 = -5<br />
+3 +3<br />
x = 8<br />
these<br />
x = -2<br />
equations<br />
The solution is then:<br />
x = -2 or x = 8<br />
{-2, 8}<br />
This second method is the CPM and is how I recommend you work these problems.<br />
© William James Calhoun, 2001<br />
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
Now, what if we introduce inequalities into the absolute value mix.<br />
What does |x| < n mean?<br />
Again, this can be rewritten as:<br />
|x - 0| < n<br />
This tells us that, “The distance between x and zero is less than n.”<br />
Using a real number for n, we can see graphically what is going on.<br />
|x| < 4<br />
Center on the 0.<br />
Run four units to the right, but<br />
aka<br />
do not include the number four<br />
|x - 0| < 4<br />
since it is not “or equal to.” equal to.”<br />
4 units 4 units<br />
Since the problem says the<br />
distance is “less than 4,” we<br />
need to shade everything<br />
between the center line and 4.<br />
Now, run four units to the left,<br />
but do not include the number<br />
negative four since it is not “or<br />
Since the problem says the distance<br />
is “less than 4,” we need to shade<br />
everything between the center line<br />
and -4.<br />
-6 -4 -2 0 2 4 6<br />
The answer to the inequality, |x| < 4 is then:<br />
{x | -4 < x < 4}<br />
If |x| < 4 then we can see that either:<br />
x < 4 AND x > -4<br />
We will use this to solve inequalities.<br />
© William James Calhoun, 2001<br />
2
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
EXAMPLE 2: Solve |3 + 2x| < 11 and graph the solution set.<br />
Use what we just saw to rewrite the problem as two inequalities connected with “and.”<br />
3 + 2x < 11 and 3 + 2x > -11<br />
-3 -3 Now solve the -3 -3<br />
2x < 8 inequalities. 2x > -14<br />
2 2 2 2<br />
x < 4 and<br />
x > -7<br />
{x | -7 < x < 4}<br />
Notice the inequality switch and<br />
sign change on the 11!<br />
Graph it:<br />
-8 -6 -4 -2 0 2 4<br />
© William James Calhoun, 2001<br />
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
When the problem was an absolute value “less than” something, like<br />
this:<br />
|x - #| < #<br />
the solution had “and” in it because the set of answers were contained<br />
between two numbers.<br />
When the problem is an absolute value “greater than” something, like<br />
this:<br />
|x - #| > #<br />
the solution will be different.<br />
The solution set is not contained by the end number.<br />
The solution set will be outside the bounds of the end number.<br />
The solution will NOT contain “and”, so it must contain…<br />
OR.<br />
© William James Calhoun, 2001<br />
3
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
Look at |x| > 4.<br />
Remember this can be written as:<br />
|x - 0| > 4.<br />
This tells us, “The distance between x and zero is greater than 4 units.”<br />
Graphically:<br />
Center on the 0.<br />
Run four units to the right, but<br />
do not include the number four<br />
since it is not “or equal to.”<br />
Since the problem says the<br />
distance is “greater than 4,”<br />
we need to shade everything<br />
to the right of 4.<br />
Now, run four units to the left,<br />
but do not include the number<br />
negative four since it is not “or<br />
equal to.”<br />
Since the problem says the<br />
distance is “greater than 4,” we<br />
need to shade everything to the<br />
left of -4.<br />
The solution set is:<br />
{x | x < -4 or x > 4}<br />
Out of set-builder<br />
notation:<br />
x > 4 or x < -4<br />
4 units 4 units<br />
-6 -4 -2 0 2 4 6<br />
We will solve greater than absolute value inequalities<br />
the same way as lesser than absolute value inequalities.<br />
Just remember:<br />
< yields an and answer, and<br />
> yields an or answer.<br />
© William James Calhoun, 2001<br />
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
EXAMPLE 3: Solve |5 + 2y| ≥ 3 and graph the solution set.<br />
Use what we just saw to rewrite the problem as two inequalities connected with “or.”<br />
5 + 2y ≥ 3 or 5 + 2y ≤ -3<br />
-5 -5 Now solve the -5 -5<br />
2y ≥ -2 inequalities. 2y ≤ -8<br />
2 2 2 2<br />
y ≥ -1 or<br />
y ≤ -4<br />
{y | y ≥ -1 or y ≤ -4}<br />
Notice the inequality switch and<br />
sign change on the 11!<br />
Graph it:<br />
-8 -6 -4 -2 0 2 4<br />
© William James Calhoun, 2001<br />
4
7-6: <strong>Solving</strong> <strong>Open</strong> <strong>Sentences</strong> <strong>Involving</strong> <strong>Absolute</strong> <strong>Value</strong><br />
HOMEWORK<br />
Page 424<br />
#19 - 37 odd<br />
© William James Calhoun, 2001<br />
5