Euler's partition theorem and the combinatorics of -sequences
Euler's partition theorem and the combinatorics of -sequences Euler's partition theorem and the combinatorics of -sequences
Let u l and v l be the roots of the polynomial x 2 − lx + 1: Then u l = l + √ l 2 − 4 ; v l = l − √ l 2 − 4 2 2 u l + v l = l; u l v l = 1. a (l) n = un l − v n l u l − v l . For real r, define ∆ (l) r = ul r + v l r . Then for integer n ∆ (l) n = a (l) n+1 − a(l) n−1 . (∆ is the l analog of “2”.) (∆ (l) n/2 )2 = u n l + 2(u lv l ) n/2 + v n l = ∆ (l) n + 2. ∆ (l) −r = u−r l + v −r l = vl r + ur l = ∆(l) r
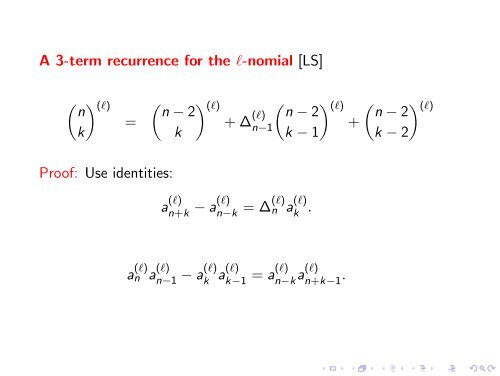
A 3-term recurrence for the l-nomial [LS] ( n k ) (l) = ( n − 2 k ) (l) + ∆ (l) n−1 ( ) n − 2 (l) + k − 1 ( ) n − 2 (l) k − 2 Proof: Use identities: a (l) n+k − a(l) n−k = ∆(l) n a (l) k . a n (l) a (l) n−1 − a(l) k a(l) k−1 = a(l) n−k a(l) n+k−1 .
- Page 1 and 2: Euler’s partition theorem and the
- Page 3 and 4: Overview Euler’s partition theore
- Page 5 and 6: Overview 1, 2, 3, . . . l-sequences
- Page 7 and 8: Overview 1, 2, 3, . . . l-sequences
- Page 9 and 10: Sylvester’s Bijection
- Page 11 and 12: l-sequences For integer l ≥ 2, de
- Page 13 and 14: l-sequences For integer l ≥ 2, de
- Page 15 and 16: l = 2 The l-Euler theorem [BME2]: T
- Page 17 and 18: l = 3 The l-Euler theorem [BME2]: T
- Page 19: The insertion step To insert a k +
- Page 22 and 23: Binary numeration system 1 0 1 1 0
- Page 24 and 25: Binary numeration system 1 0 1 1 0
- Page 26: Theorem [Fraenkel 1985] Every nonne
- Page 31 and 32: Lecture Hall Partitions
- Page 33 and 34: The Lecture Hall Theorem [BME1] The
- Page 35 and 36: Θ (l) n : Bijection for the l-Lect
- Page 44 and 45: Truncated lecture hall partitions L
- Page 46 and 47: Theorem [Corteel,S 2004] Given posi
- Page 48 and 49: The l-nomial coefficient Example (
- Page 50 and 51: Let u l and v l be the roots of the
- Page 54 and 55: An l-nomial theorem [LS]: An analog
- Page 56 and 57: A coin-flipping interpretation of t
- Page 58 and 59: Define a q-analog of the l-nomial:
- Page 60 and 61: Another q-analog of the l-nomial Le
- Page 62 and 63: Question: When l = 2, several refin
- Page 64 and 65: Question: What is the generating fu
- Page 66 and 67: CanaDAM 2009 2nd Canadian Discrete
A 3-term recurrence for <strong>the</strong> l-nomial [LS]<br />
( n<br />
k<br />
) (l)<br />
=<br />
( n − 2<br />
k<br />
) (l)<br />
+ ∆ (l)<br />
n−1<br />
( ) n − 2 (l)<br />
+<br />
k − 1<br />
( ) n − 2 (l)<br />
k − 2<br />
Pro<strong>of</strong>: Use identities:<br />
a (l)<br />
n+k − a(l)<br />
n−k = ∆(l) n a (l)<br />
k .<br />
a n<br />
(l) a (l)<br />
n−1 − a(l) k<br />
a(l) k−1 = a(l) n−k a(l) n+k−1 .