Solution to Laplace's Equation in Cylindrical Coordinates 1 ...
Solution to Laplace's Equation in Cylindrical Coordinates 1 ...
Solution to Laplace's Equation in Cylindrical Coordinates 1 ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
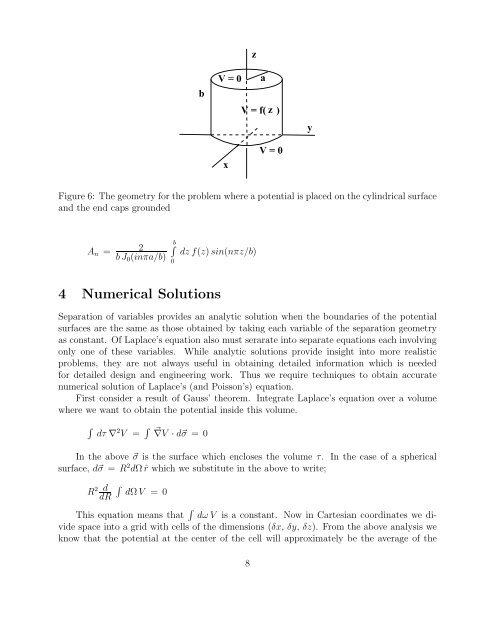
V = 0<br />
z<br />
a<br />
z V = f( )<br />
y<br />
x<br />
V = 0<br />
Figure 6: The geometry for the problem where a potential is placed on the cyl<strong>in</strong>drical surface<br />
and the end caps grounded<br />
A n =<br />
2<br />
bJ 0 (<strong>in</strong>πa/b)<br />
∫ b<br />
0<br />
dz f(z) s<strong>in</strong>(nπz/b)<br />
4 Numerical <strong>Solution</strong>s<br />
Separation of variables provides an analytic solution when the boundaries of the potential<br />
surfaces are the same as those obta<strong>in</strong>ed by tak<strong>in</strong>g each variable of the separation geometry<br />
as constant. Of Laplace’s equation also must serarate <strong>in</strong><strong>to</strong> separate equations each <strong>in</strong>volv<strong>in</strong>g<br />
only one of these variables. While analytic solutions provide <strong>in</strong>sight <strong>in</strong><strong>to</strong> more realistic<br />
problems, they are not always useful <strong>in</strong> obta<strong>in</strong><strong>in</strong>g detailed <strong>in</strong>formation which is needed<br />
for detailed design and eng<strong>in</strong>eer<strong>in</strong>g work. Thus we require techniques <strong>to</strong> obta<strong>in</strong> accurate<br />
numerical solution of Laplace’s (and Poisson’s) equation.<br />
First consider a result of Gauss’ theorem. Integrate Laplace’s equation over a volume<br />
where we want <strong>to</strong> obta<strong>in</strong> the potential <strong>in</strong>side this volume.<br />
∫<br />
dτ ∇ 2 V = ∫ ⃗ ∇V · d⃗σ = 0<br />
In the above ⃗σ is the surface which encloses the volume τ. In the case of a spherical<br />
surface, d⃗σ = R 2 dΩ ˆr which we substitute <strong>in</strong> the above <strong>to</strong> write;<br />
R 2 d<br />
dR<br />
∫<br />
dΩV = 0<br />
This equation means that ∫ dω V is a constant. Now <strong>in</strong> Cartesian coord<strong>in</strong>ates we divide<br />
space <strong>in</strong><strong>to</strong> a grid with cells of the dimensions (δx, δy, δz). From the above analysis we<br />
know that the potential at the center of the cell will approximately be the average of the<br />
8