download report - Sapienza

Scientific Report 2007-2009
Theoretical physics
T22. Towards a theory of chaos explained as travel on Riemann
surfaces
The fact that the distinction among integrable or nonintegrable
behaviors of a dynamical system is somehow
connected with the analytic structure of the solutions of
the model under consideration as functions of the independent
variable “time” (considered as a complex variable)
is by no means a novel notion. It goes back to
classical work by Carl Jacobi, Henri Poincaré, Sophia
Kowalevskaya, Paul Painlevé, and, in recent times, attracted
the attention of Martin Kruskal and others. A
simple-minded rendition of Kruskal’s teachings on this
subject can be described as follows: for an evolution to
be integrable, it should be expressible, at least in principle,
via formulas that are not excessively multivalued
in terms of the dependent variable, entailing that, to the
extent this evolution is expressible by analytic functions
of the dependent variable (considered as a complex variable),
it might possess branch points, but it should not
feature an infinity of them that is dense in the complex
plane of the independent variable. Many interesting results
were obtained along this line of research, mainly
by use only of numerical and local techniques (like the
Painlevé analysis), which, albeit useful and widely applicable,
provide no information on the global properties of
the Riemann surfaces of the solutions (e.g. the number
and location of the movable branch points and how the
sheets of the Riemann surface are connected together at
those branch points), a detailed analysis of which provides
a much deeper understanding of the dynamics.
a rich behaviour, possibly including irregular or chaotic
characteristics. It was shown in which sense the model
displays sensitive dependence on the initial conditions
and on the parameters, describing a mechanism to explain
the transition from regular to irregular motions [1].
We have also studied the complexification of the
one-dimensional Newtonian particle in a monomial
potential, discussing cyclic motions on the associated
Riemann surface, corresponding to a class of real
and autonomous Newtonian dynamics in the plane.
For small data, the cyclic time trajectories lead to
isochronous dynamics. For bigger data the situation is
quite complicated; computer experiments show that, for
sufficiently small degree of the monomial, the motion is
generically periodic with integer period, which depends
in a quite sensitive way on the initial data. If the
degree of the monomial is sufficiently high, computer
experiments show essentially chaotic behaviour. We
have suggested a possible theoretical explanation of
these different behaviours. We have also introduced
a one-parameter family of 2-dimensional mappings,
describing the motion of the center of the circle, as a
convenient representation of the cyclic dynamics; we
call such mapping the center map. Computer experiments
for the center map show a typical multi-fractal
behaviour with periodicity islands [2]. Therefore the
above complexification procedure generates dynamics
amenable to analytic treatment and possessing a high
degree of complexity.
Figure 1: Example of locus of the roots (left) and branchcut
structure (right) of the algebraic equation that defines
the Riemann surface associated to the solution of the 3-body
model studied in [1] for a certain choice of the coupling constants
and the initial data.
In the last few years people in our group, in collaboration
with other researchers, have contributed to deepen
the mechanism for the onset of irregular (chaotic) motions
in a deterministic context by introducing a new
dynamical system, interpretable as a 3-body problem in
the (complex) plane, which is simple enough that a full
description of the Riemann surface of its solution can be
performed (via analytical, geometro-algebraic and combinatoric
techniques), yet complicated enough to feature
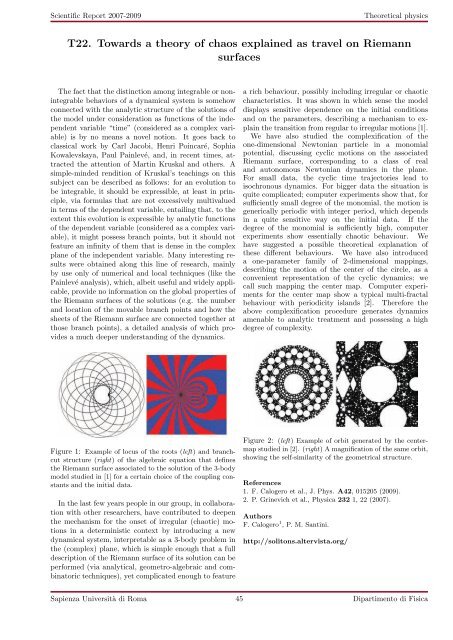
Figure 2: (left) Example of orbit generated by the centermap
studied in [2]. (right) A magnification of the same orbit,
showing the self-similarity of the geometrical structure.
References
1. F. Calogero et al., J. Phys. A42, 015205 (2009).
2. P. Grinevich et al., Physica 232 1, 22 (2007).
Authors
F. Calogero 1 , P. M. Santini.
http://solitons.altervista.org/
Sapienza Università di Roma 45 Dipartimento di Fisica