download report - Sapienza
download report - Sapienza
download report - Sapienza
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Scientific Report 2007-2009<br />
Theoretical physics<br />
T21. Propagation and breaking of weakly nonlinear<br />
and quasi one dimensional waves in Nature<br />
Take any system of nonlinear PDEs i) characterized,<br />
for example, by nonlinearities of hydrodynamic type and<br />
ii) whose linear limit, at least in some approximation,<br />
is described by the wave equation. Then, iii) looking<br />
at the propagation of quasi one dimensional waves and<br />
iv) neglecting dispersion and dissipation, one obtains,<br />
at the second order in the proper multiscale expansion,<br />
the dispersionless Kadomtsev - Petviashvili equation in<br />
n + 1 dimensions (dKP n ): (u t + uu x ) x<br />
+ ∆ ⊥ u = 0, u =<br />
u(x, ⃗y, t), ⃗y = (y 1 , . . . , y n−1 ), ∆ ⊥ = n−1 ∑<br />
∂y 2 i<br />
. Therefore<br />
i=1<br />
dKP n arises in several physical contexts, like acoustics,<br />
plasma physics and hydrodynamics.<br />
We remark that the 1+1 dimensional version of dKP n<br />
is the celebrated Riemann-Hopf equation u t + uu x = 0,<br />
the prototype model in the description of the gradient<br />
catastrophe (or wave breaking) of one dimensional<br />
waves. Therefore a natural question arises: do solutions<br />
of dKP n break and, if so, is it possible to give an analytic<br />
description of such a multidimensional wave breaking?<br />
It was observed long ago that the commutation of multidimensional<br />
vector fields can generate integrable nonlinear<br />
partial differential equations (PDEs) in arbitrary<br />
dimensions. Some of these equations are dispersionless<br />
(or quasi-classical) limits of integrable PDEs, having<br />
dKP 2 as prototype example, they arise in various problems<br />
of Mathematical Physics and are intensively studied<br />
in the recent literature.<br />
We have recently developed the Inverse Spectral<br />
Transform (IST) for 1-parameter families of multidimensional<br />
vector fields, and used it to construct the formal<br />
solution of the Cauchy problem for distinguished examples<br />
of nonlinear PDEs of Mathematical Physics. This<br />
IST and its associated nonlinear Riemann-Hilbert Dressing<br />
scheme turn out to be efficient tools to study also<br />
other relevant properties of the solution space of the<br />
PDE under consideration: i) the characterization of a<br />
distinguished class of spectral data for which the associated<br />
nonlinear RH problem is linearized, corresponding<br />
to a class of implicit solutions of the PDE; ii) the<br />
construction of the longtime behaviour of the solutions<br />
of the Cauchy problem; iii) the possibility to establish<br />
whether or not the lack of dispersive terms in the nonlinear<br />
PDE causes the breaking of localized initial profiles<br />
and, if yes, to investigate in a surprisingly explicit<br />
way the analytic aspects of such a multidimensional wave<br />
breaking.<br />
In this way it was possible to establish that localized<br />
initial data evolving according to dKP 2 generically<br />
break. This exact theory has been recently used to<br />
build a uniform approximation of the solution of the<br />
Cauchy problem for dKP n , for small and localized initial<br />
data, showing that such initial data evolving according<br />
to dKP n break, in the long time regime, if and only if<br />
1 ≤ n ≤ 3; i.e., in physical space. Such a wave breaking<br />
takes place, generically, in a point of the paraboloidal<br />
wave front, and the analytic aspects of it are given explicitly<br />
in terms of the small initial data.<br />
The existence of a critical dimensionality above which<br />
small data do not break has a clear origin, since, in the<br />
model, two terms act in opposite way: the nonlinearity<br />
is responsible for the steepening of the profile, while the<br />
n−1 diffraction channels, represented by the transversal<br />
Laplacian, have an opposite effect; for n = 1, 2, 3 the<br />
nonlinearity prevails and wave breaking takes place (but<br />
at longer and longer time scales, as n increases), while,<br />
for n ≥ 4, the number of transversal diffraction channels<br />
is enough to prevent such phenomenon, in the longtime<br />
regime.<br />
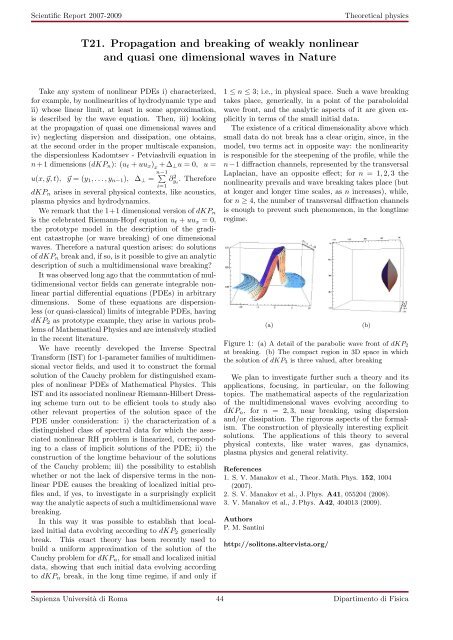
(a)<br />
Figure 1: (a) A detail of the parabolic wave front of dKP 2<br />
at breaking. (b) The compact region in 3D space in which<br />
the solution of dKP 3 is three valued, after breaking<br />
We plan to investigate further such a theory and its<br />
applications, focusing, in particular, on the following<br />
topics. The mathematical aspects of the regularization<br />
of the multidimensional waves evolving according to<br />
dKP n , for n = 2, 3, near breaking, using dispersion<br />
and/or dissipation. The rigorous aspects of the formalism.<br />
The construction of physically interesting explicit<br />
solutions. The applications of this theory to several<br />
physical contexts, like water waves, gas dynamics,<br />
plasma physics and general relativity.<br />
(b)<br />
References<br />
1. S. V. Manakov et al., Theor. Math. Phys. 152, 1004<br />
(2007).<br />
2. S. V. Manakov et al., J. Phys. A41, 055204 (2008).<br />
3. V. Manakov et al., J. Phys. A42, 404013 (2009).<br />
Authors<br />
P. M. Santini<br />
http://solitons.altervista.org/<br />
<strong>Sapienza</strong> Università di Roma 44 Dipartimento di Fisica